Unit Circle With Tangent
The unit circle with tangent gives the values of the tangent function (which is usually referred to as "tan") for different standard angles from 0° to 360°. Usually, the general unit circle gives the values of sin (sine function) and cos (cosine function). These values can be used to compute the unit circle with tangent by using the relation between tan, sin, and cos, which is tan x = (sin x)/(cos x).
Let us learn more about unit circle with tangent values along with unit circle with the tangent chart. Also, let us see how to graph the tangent function using the unit circle.
How to Compute Unit Circle With Tangent Values?
The unit circle with tangent is also known as the trigonometric circle of the tangent function. It gives the values of the trigonometric function "tan" for different standard angles that lie between 0° and 360°. The standard angles between 0° and 360° are tabulated below both in terms of degrees and radians. We are going to first compute the "unit circle with tangent chart" through which we can easily draw the unit circle with tangent.
Unit Circle With Tangent Chart
To compute the value of tangent, let us recall the values of sin and cos at the standard angles from 0 to 2π.
| Degrees | Radians | sin | cos |
|---|---|---|---|
| 0° | 0 | 0 | 1 |
| 30° | π/6 | 1/2 | √3/2 |
| 45° | π/4 | √2/2 | √2/2 |
| 60° | π/3 | √3/2 | 1/2 |
| 90° | π/2 | 1 | 0 |
| 120° | 2π/3 | √3/2 | -1/2 |
| 135° | 3π/4 | √2/2 | -√2/2 |
| 150° | 5π/6 | 1/2 | -√3/2 |
| 180° | π | 0 | -1 |
| 210° | 7π/6 | -1/2 | -√3/2 |
| 225° | 5π/4 | -√2/2 | -√2/2 |
| 240° | 4π/3 | -√3/2 | -1/2 |
| 270° | 3π/2 | -1 | 0 |
| 300° | 5π/3 | -√3/2 | 1/2 |
| 315° | 7π/4 | -√2/2 | √2/2 |
| 330° | 11π/6 | -1/2 | √3/2 |
| 360° | 2π | 0 | 1 |
Now, we will use the identity tan x = (sin x)/(cos x) in each row to compute the corresponding value of tangent. Then we get
| Degrees | Radians | tan x = (sin x) ÷ (cos x) |
|---|---|---|
| 0° | 0 | 0 ÷ 1 = 0 |
| 30° | π/6 | 1/2 ÷ √3/2 = √3/3 |
| 45° | π/4 | √2/2 ÷ √2/2 = 1 |
| 60° | π/3 | √3/2 ÷ 1/2 = √3 |
| 90° | π/2 | 1 ÷ 0 = Not defined |
| 120° | 2π/3 | √3/2 ÷ -1/2 = -√3 |
| 135° | 3π/4 | √2/2 ÷ -√2/2 = -1 |
| 150° | 5π/6 | 1/2 ÷ -√3/2 = -√3/3 |
| 180° | π | 0 ÷ -1 = 0 |
| 210° | 7π/6 | -1/2 ÷ -√3/2 = √3/3 |
| 225° | 5π/4 | -√2/2 ÷ -√2/2 = 1 |
| 240° | 4π/3 | -√3/2 ÷ -1/2 = √3 |
| 270° | 3π/2 | -1 ÷ 0 = Not defined |
| 300° | 5π/3 | -√3/2 ÷ 1/2 = -√3 |
| 315° | 7π/4 | -√2/2 ÷ √2/2 = -1 |
| 330° | 11π/6 | -1/2 ÷ √3/2 = -√3/3 |
| 360° | 2π | 0 ÷ 1 = 0 |
Thus, the unit circle with tangent chart is as follows:
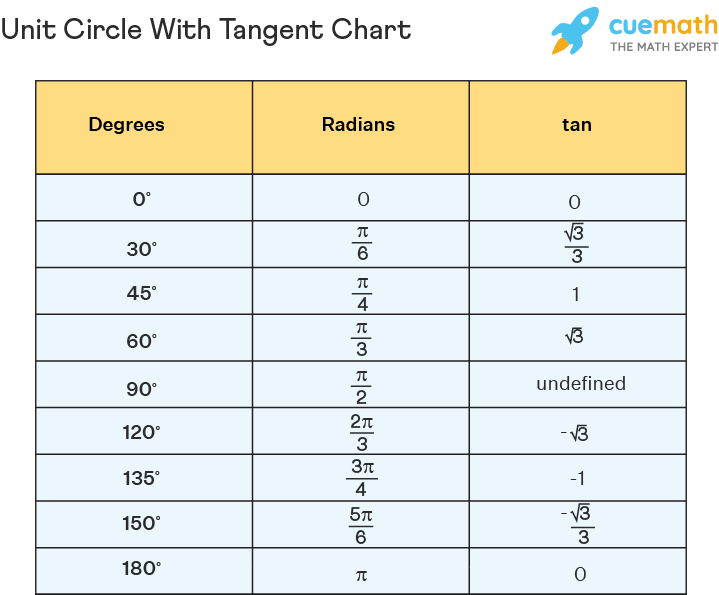

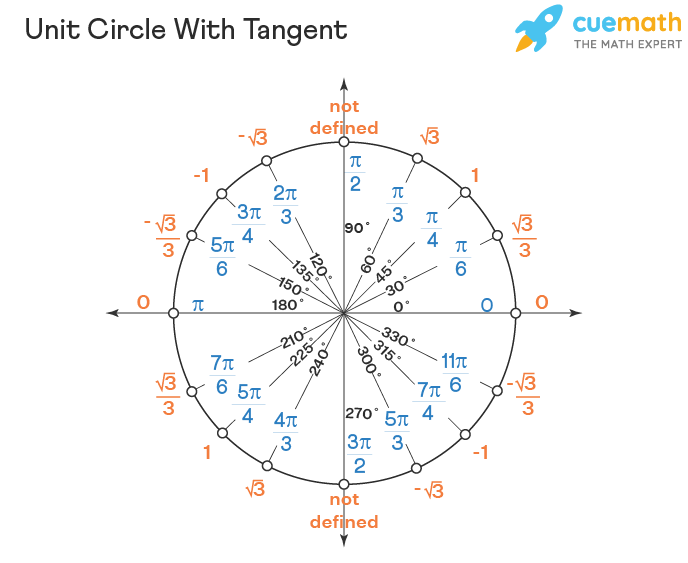
Unit Circle With Tangent
We have already calculated the values of tangent at different standard angles in the previous section. Let us just plot the angles in the unit circle along with their corresponding values of tangent. This gives the unit circle with tangent.
Is this giving a feel that we can't remember it? Here are some tricks to remember the unit circle with tangent.
How to Remember Unit Circle With Tangent?
Here are the hints to remember the unit circle with tangent values.
- Just remember 5 values "0, √3/3, 1, √3, and undefined" in order. These values are the values of tangent in the first quadrant in the anti-clockwise direction.
- We get the same values in every quadrant such that the values are symmetric about both the x-axis and y-axis (but the signs may be changed).
- Keep in mind that tan values are positive ONLY in first and third quadrants.
In the second and fourth quadrants, the tan values are negative.
Graphing Tangent Using Unit Circle
From the unit circle with tangent, we can clearly see that tan is NOT defined for the angles π/2 and 3π/2. So we get vertical asymptotes at x = π/2 and at x = 3π/2 in the graph of tangent function. Let us plot the x-axis with the angles from 0 to 2π with the intervals of π/4 and y-axis with real numbers. Let us just use the "unit circle with tangent chart" to plot the points and join them by curves taking care of the vertical asymptotes. While plotting the points, let us use the following decimal approximations:
- √3 ≈ 1.732
- √3/3 ≈ 0.577
Here is the graph of the tangent function using the unit circle.

Important Points on Unit Circle With Tangent:
- Tangent is NOT defined only at π/2 and 3π/2 on the unit circle.
- The tan values on unit circle are symmetric with respect to both the axes except for the signs.
- Tan is positive in 1st and 3rd quadrants and
tan is negative in 2nd and 4th quadrants.
Related Topics:
Examples on Unit Circle With Tangent
-
Example 1: By the unit circle with tangent, state the values of: a) tan 225° b) tan 270°.
Solution:
By the tan values from unit circle, it directly follows that:
a) The value of tan 225° is 1.
b) The value of tan 270° is Not defined.
Answer: a) tan 225° = 1; b) tan 270° = Not defined.
-
Example 2: Use the unit circle with tangent to compute the values of: a) tan 495° b) tan 900°.
Solution:
When the angle is beyond 360°, then we find its coterminal angle by adding or subtracting multiples of 360° to get the angle to be within 0° and 360°.
a) The co-terminal angle of 495° = 495° - 360° = 135°.
tan 495° = tan 135° = -1.
b) The co-terminal angle of 900° = 900° - 2(360°) = 180°.
tan 900° = tan 180° = 0.
Answer: a) tan 495° = -1; b) tan 900° = 0.
-
Example 3: Evaluate 2 tan 5π/6 + 5 tan 7π/6 + 3 tan 11π/6.
Solution:
By the unit circle with tangent,
- tan 5π/6 = -√3/3
- tan 7π/6 = √3/3
- tan 11π/6 = -√3/3
Thus, 2 tan 5π/6 + 5 tan 7π/6 + 3 tan 11π/6
= 2 (-√3/3) + 5 (√3/3) + 3 (-√3/3)
= 0
Answer: 2 tan 5π/6 + 5 tan 7π/6 + 3 tan 11π/6 = 0.

FAQs on Unit Circle With Tangent
How do You Find Unit Circle With Tangent?
We already have cos and sin values on the unit circle where each point on the unit circle gives the coordinates (cos, sin). We have an identity tan x = (sin x) / (cos x). So divide the y-coordinate by the x-coordinate of each point on the unit circle to find the corresponding tangent value.
What Part of Unit Circle is Tangent?
In unit circle, tangent is not usually present, instead just cos and sin values are present. If we divide the sin by cos corresponding to an angle, then we can get the tangent of the angle. This is because tan x = (sin x)/(cos x).
What is tan 11π/6 From Unit Circle With Tangent?
11π/6 in terms of degrees is 330°. By unit circle with tangent tan 11π/6 = tan 330° = -√3/3.
How to Find Tangent of a Number Using Unit Circle?
On the unit circle, we have cos and sin values. For example, for the angle 45°, the corresponding point on the unit circle is (cos 45°, sin 45°) = (√2/2, √2/2). Since tan x = (sin x)/(cos x), we just divide sin value by cos value to get the corresponding tan value. In this example, tan 45° = (sin 45°)/(cos45°) = (√2/2) / (√2/2) = 1.
What is tan 5π/4 From Unit Circle With Tangent?
5π/4 in terms of degrees is 225°. By unit circle with tangent tan 5π/4 = tan 225° = 1.
How to Compute Unit Circle With Tangent Values?
To compute the unit circle with tangent values:
- Draw the unit circle with standard angles.
- Write the corresponding point for each angle on the circle which represents (cos, sin)
- Divide sin by cos values to get corresponding tan values.
Where is Tangent Undefined on Unit Circle?
Since tan x = (sin x)/(cos x), tan x is not defined wherever cos x = 0. On the unit circle, cos x is 0 when x = π/2 and when x = 3π/2. At these values tan is undefined.
visual curriculum