Trigonometry Formulas

Trigonometry formulas are sets of different formulas involving trigonometric identities, used to solve problems based on the sides and angles of a right-angled triangle. Additionally, there are many trigonometric identities and formulas that can be used to simplify expressions, solve equations, and evaluate integrals.

These trigonometry formulas include trigonometric functions like sine, cosine, tangent, cosecant, secant, and cotangent for given angles. Let us learn these formulas involving Pythagorean identities, product identities, co-function identities (shifting angles), sum & difference identities, double angle identities, half-angle identities, etc. in detail in the following sections.

1.	What are Trigonometry Formulas?
2.	Basic Trigonometry Formulas
3.	Reciprocal Identities
4.	Pythagorean Identities
5.	Trigonometric Ratio Table
6.	Unit Circle Formulas
7.	Trigonometry Periodic Identities (in Radians)
8.	Co-function Identities(in Degrees)
9.	Sum and Difference Identities
10.	Multiple and Sub-Multiple Angles
11.	Sum and Product of Identities
12.	Inverse Trigonometry Formulas
13.	Sine and Cosine Laws
14.	FAQs on Trigonometry Formulas

Trigonometry formulas are mathematical expressions that relate the angles and sides of a right triangle. They are used in trigonometry to solve a wide range of problems related to angles, distances, and heights. By using these formulas, one can find the missing side or angle in a right triangle.

In addition to basic formulas such as the Pythagorean theorem, there are also many trigonometric identities and formulas that can be used to simplify expressions, solve equations, and evaluate integrals. These formulas are essential tools for engineers, mathematicians, and scientists working in a variety of fields.

List of All Formulas of Trigonometry

Let us look at the below sets of different trigonometry formulas.

• Basic Trig Ratio Formulas: formulas relating to the basic trigonometric ratios sin, cos, tan, etc.
• Reciprocal Identities: formulas dealing with the reciprocal relationship between trig ratios.
• Trigonometric Ratio Table: Trigonometry values are depicted for standard angles in the trigonometry table.
• Periodic Identities: trigonometry formulas that help in finding values of trig functions for a shift in angles by π/2, π, 2π, etc.
• Co-function Identities: formulas that depict interrelationships between the trigonometry functions.
• Sum and Difference Identities: formulas used to find the value of the trigonometry function for the sum or difference in angles.
• Half, Double and Triple Identities: formulas used to find the values of trig functions for half, double or triple angles.
• Sum to Product Identities: formulas used to represent the product of trigonometry functions as their sum or vice-versa.
• Inverse Trigonometry Formulas: formulas related to inverse trig functions like sine inverse, cosine inverse, etc.
• Sine Law and Cosine Law

Some basic trigonometry formulas can be observed in the image below. Let us study them in detail in the following sections.

Basic trigonometry formulas are used to find the relationship between trig ratios and the ratio of the corresponding sides of a right-angled triangle. There are basic 6 trigonometric ratios used in trigonometry, also called trigonometric functions- sine, cosine, secant, co-secant, tangent, and co-tangent, written as sin, cos, sec, csc, tan, cot in short. The trigonometric functions and identities are derived using a right-angled triangle as the reference. We can find out the sine, cosine, tangent, secant, cosecant, and cotangent values, given the dimensions of a right-angled triangle, using trigonometry formulas as,

Trigonometric Ratio Formulas

• sin θ = Perpendicular/Hypotenuse
• cos θ = Base/Hypotenuse
• tan θ = Perpendicular/Base
• sec θ = Hypotenuse/Base
• cosec θ = Hypotenuse/Perpendicular
• cot θ = Base/Perpendicular

Cosecant, secant, and cotangent are the reciprocals of the basic trigonometric ratios sine, cosine, and tangent respectively. All of the reciprocal identities are also derived using a right-angled triangle as a reference. These reciprocal trigonometric identities are derived using trigonometric functions. The trigonometry formulas on reciprocal identities, given below, are used frequently to simplify trigonometric problems.

• cosec θ = 1/sin θ; sin θ = 1/cosec θ
• sec θ = 1/cos θ; cos θ = 1/sec θ
• cot θ = 1/tan θ; tan θ = 1/cot θ

Pythagoras theorem states that "in a right triangle, if 'c' is the hypotenuse and 'a' and 'b' are the two legs then c² = a² + b²". Using this theorem and trigonometric ratios, Pythagorean identities are derived. These identities are used to convert one trig ratio into other. The Pythagorean trig identities are mentioned below:

• sin²θ + cos²θ = 1
• sec²θ - tan²θ = 1
• csc²θ - cot²θ = 1

Here is a table for trigonometry formulas for angles that are commonly used for solving trigonometry problems. The trigonometric ratios table helps in finding the values of trigonometric standard angles such as 0°, 30°, 45°, 60°, and 90°.

Angles (In Degrees)	0°	30°	45°	60°	90°	180°	270°	360°
Angles (In Radians)	0°	π/6	π/4	π/3	π/2	π	3π/2	2π
sin	0	1/2	1/√2	√3/2	1	0	-1	0
cos	1	√3/2	1/√2	1/2	0	-1	0	1
tan	0	1/√3	1	√3	∞	0	∞	0
cosec	∞	2	√2	2/√3	1	∞	-1	∞
sec	1	2/√3	√2	2	∞	-1	∞	1
cot	∞	√3	1	1/√3	0	∞	0	∞

The unit circle is a circle with a radius of 1 and center at the origin of a coordinate plane. It is used in trigonometry (as shown below) to define the values of trigonometric functions for all angles, including those outside the range of 0 to 90 degrees.

Here are some of the formulas associated with the unit circle:

• sin θ = y/1; csc θ = 1/y
• cos θ = x/1; sec θ = 1/x
• tan θ = sinθ/cosθ = y/x; cot θ = cosθ/sinθ = x/y

Trigonometry formulas involving periodic identities are used to shift the angles by π/2, π, 2π, etc. All trigonometric identities are cyclic in nature which means that they repeat themselves after a period. This period differs for different trigonometry formulas on periodic identities. For example, tan 30° = tan 210° but the same is not true for cos 30° and cos 210°. You can refer to the trigonometry formulas given below to verify the periodicity of sine and cosine functions in different quadrants.

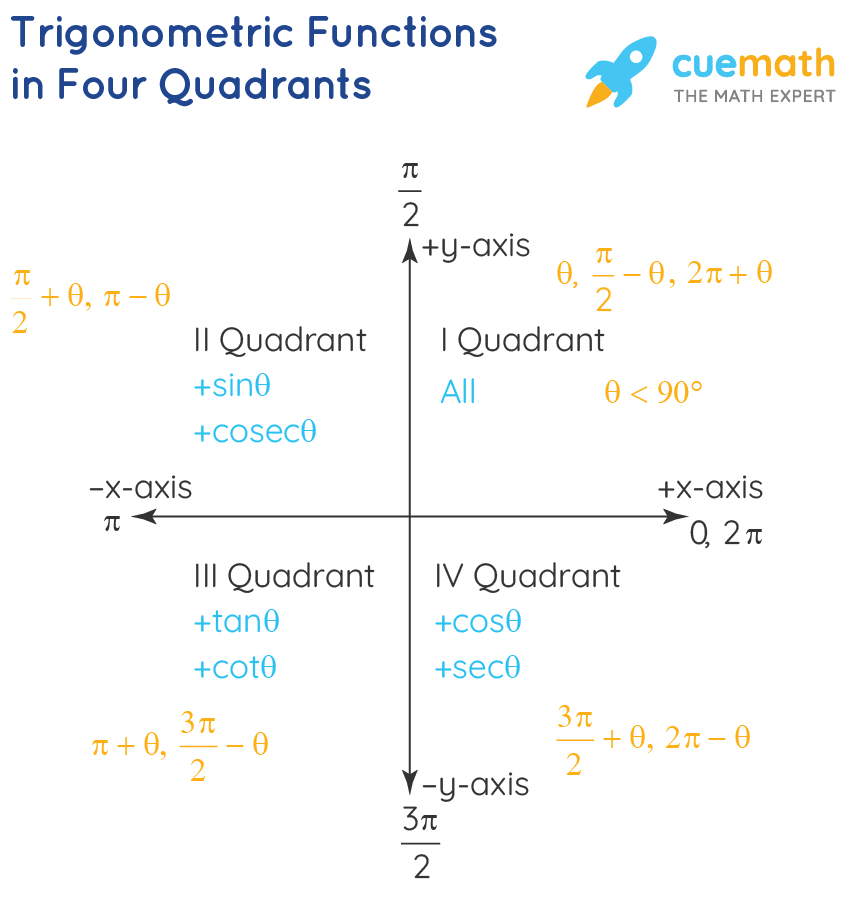
First Quadrant:

• sin (π/2 – θ) = cos θ
• cos (π/2 – θ) = sin θ
• sin (2π + θ) = sin θ
• cos (2π + θ) = cos θ

Second Quadrant:

• sin (π/2 + θ) = cos θ
• cos (π/2 + θ) = – sin θ
• sin (π – θ) = sin θ
• cos (π – θ) = – cos θ

Third Quadrant:

• sin (π + θ) = – sin θ
• cos (π + θ) = – cos θ
• sin (3π/2 – θ) = – cos θ
• cos (3π/2 – θ) = – sin θ

Fourth Quadrant:

• sin (3π/2 + θ) = – cos θ
• cos (3π/2 + θ) = sin θ
• sin (2π – θ) = – sin θ
• cos (2π – θ) = cos θ

The trigonometry formulas on cofunction identities provide the interrelationship between the different trigonometry functions. The co-function trigonometry formulas are represented in degrees below:

• sin(90° − x) = cos x
• cos(90° − x) = sin x
• tan(90° − x) = cot x
• cot(90° − x) = tan x
• sec(90° − x) = cosec x
• cosec(90° − x) = sec x

The cofunction identities in terms of radians can be obtained by replacing 90° with π/2 in the above formulas.

The sum and difference identities include the trigonometry formulas of sin(x + y), cos(x - y), cot(x + y), etc.

• sin(x + y) = sin(x)cos(y) + cos(x)sin(y)
• cos(x + y) = cos(x)cos(y) - sin(x)sin(y)
• tan(x + y) = (tan x + tan y)/(1 - tan x • tan y)
• sin(x – y) = sin(x)cos(y) - cos(x)sin(y)
• cos(x – y) = cos(x)cos(y) + sin(x)sin(y)
• tan(x − y) = (tan x - tan y)/(1 + tan x • tan y)

Trigonometry formulas for multiple and sub-multiple angles can be used to calculate the value of trigonometric functions for half angle, double angle, triple angle, etc.

Half-Angle Identities

The half angle trigonometric formulas involve x/2 and are as follows.

• sin (x/2) = ±√[(1 - cos x)/2]
• cos (x/2) = ± √[(1 + cos x)/2]
• tan (x/2) = ±√[(1 - cos x)/(1 + cos x)] (or) tan (x/2) = (1 - cos x)/sin x

Double Angle Identities

The double angle trigonometry formulas are used to find the double angle (2x) of trig functions.

• sin (2x) = 2sin(x) • cos(x) = [2tan x/(1 + tan² x)]
• cos (2x) = cos²(x) - sin²(x) = [(1 - tan² x)/(1 + tan² x)] = 2cos²(x) - 1 = 1 - 2sin²(x)
• tan (2x) = [2tan(x)]/ [1 - tan²(x)]
• sec (2x) = sec² x/(2 - sec² x)
• cosec (2x) = (sec x • cosec x)/2

Triple Angle Identities

The trip angle (3x) trig formulas are as follows:

• sin 3x = 3sin x - 4sin³x
• cos 3x = 4cos³x - 3cos x
• tan 3x = [3tanx - tan³x]/[1 - 3tan²x]

Trigonometric formulas for sum or product identities are used to represent the sum of any two trigonometric functions in their product form, or vice-versa.

Product to Sum Formulas

• sinx⋅cosy = [sin(x + y) + sin(x − y)]/2
• cosx⋅cosy = [cos(x + y) + cos(x − y)]/2
• sinx⋅siny = [cos(x − y) − cos(x + y)]/2

Sum to Product Formulas

The combination of two acute angles A and B can be presented through the trigonometric ratios, in the below trigonometry formulas.

• sinx + siny = 2[sin((x + y)/2)cos((x − y)/2)]
• sinx − siny = 2[cos((x + y)/2)sin((x − y)/2)]
• cosx + cosy = 2[cos((x + y)/2)cos((x − y)/2)]
• cosx − cosy = −2[sin((x + y)/2)sin((x − y)/2)]

Using the inverse trigonometry formulas, trigonometric ratios are inverted to create the inverse trigonometric functions, like, sin θ = x and θ = sin ⁻¹x. Here x can have values in whole numbers, decimals, fractions, and exponents.

• sin^-1 (-x) = -sin^-1 x
• cos^-1 (-x) = π - cos^-1 x
• tan^-1 (-x) = -tan^-1 x
• cosec^-1 (-x) = -cosec^-1 x
• sec^-1 (-x) = π - sec^-1 x
• cot^-1 (-x) = π - cot^-1 x

Sine Law: The sine law and the cosine law give a relationship between the sides and angles of a triangle. The sine law gives the ratio of the sides and the angle opposite to the side. As an example, the ratio is taken for the side 'a' and its opposite angle 'A'.

(sin A)/a = (sin B)/b = (sin C)/c

Cosine Law: The cosine law helps to find the length of a side, for the given lengths of the other two sides and the included angle. As an example the length 'a' can be found with the help of the other two sides 'b' and 'c' and their included angle 'A'.

• a² = b² + c² - 2bc cosA
• b² = a² + c² - 2ac cosB
• c² = a² + b² - 2ab cosC

where, a, b, c are the lengths of the sides of the triangle, and A, B, C are the angles of the triangle.

☛ Related Topics:

• Trigonometry Formulas For Class 10
• Trigonometric Formulas Class 11
• Trigonometric Formulas for Class 12

 

What are Trigonometric Formulas?

Trigonometric formulas are formulas that used to solve problems based on the sides and angles of a right-angled triangle. These formulas can be used to evaluate trigonometric ratios (also referred to as trigonometric functions), sin, cos, tan, csc, sec, and cot.

What is the Basic Trigonometry Formula?

Basic trigonometry formulas involve the representing of basic trigonometric ratios in terms of the ratio of corresponding sides of a right-angled triangle. These are given as, sin θ = Opposite Side/Hypotenuse, cos θ = Adjacent Side/Hypotenuse, tan θ = Opposite Side/Adjacent Side.

What are the Involving Pythagorean Identities in Trigonometry?

The three fundamental trigonometry formulas involving the Pythagorean identities are given as,

• sin²A + cos²A = 1
• 1 + tan²A = sec²A
• 1 + cot²A = cosec²A

What are Trigonometric Ratios' Formulas?

The three main functions in trigonometry are Sine, Cosine, and Tangent. Trigonometry ratios' formulas are given as,

• Sine Function: sin(θ) = Opposite / Hypotenuse
• Cosine Function: cos(θ) = Adjacent / Hypotenuse
• Tangent Function: tan(θ) = Opposite / Adjacent

What are Trigonometry Formulas for Even and Odd Identities?

The trigonometry formulas involving even and odd identities are given as,

• sin(–x) = –sin x
• cos(–x) = cos x
• tan(–x) = –tan x
• csc (–x) = –csc x
• sec (–x) = sec x
• cot (–x) = –cot x

What are Addition Trigonometric Formulas?

The trigonometry formulas for trigonometry ratios when the angles are in addition are given as,

• sin(x + y) = sin(x)cos(y) + cos(x)sin(y)
• cos(x + y) = cos(x)cos(y) - sin(x)sin(y)
• tan(x + y) = (tan x + tan y)/(1 - tan x • tan y)

Trigonometry Formulas Are Applicable to Which Triangle?

Trigonometry formulas are applicable to right-angled triangles. These trig formulas represent the trigonometric ratios in terms of the ratio of corresponding sides of a right-angled triangle. But the formulas like sine rule and cosine rule can be applied for non-right triangles as well.

How to Remember Trigonometry Formulas Easily?

The trick to learning basic trigonometry formulas is using the mnemonic "SOHCAHTOA", which can be used to memorize trigonometric ratios as,
SOH: Sine = Opposite / Hypotenuse
CAH: Cosine = Adjacent / Hypotenuse
TOA: Tangent = Opposite / Adjacent

What is sin 3x Trigonometry Formula?

Trigonometry formula, sin 3x is the sine of three times of an angle in a right-angled triangle, it is expressed as: sin 3x = 3sin x - 4sin³x.