Sin 145 Degrees

The value of sin 145 degrees is 0.5735764. . .. Sin 145 degrees in radians is written as sin (145° × π/180°), i.e., sin (29π/36) or sin (2.530727. . .). In this article, we will discuss the methods to find the value of sin 145 degrees with examples.

• Sin 145°: 0.5735764. . .
• Sin (-145 degrees): -0.5735764. . .
• Sin 145° in radians: sin (29π/36) or sin (2.5307274 . . .)

What is the Value of Sin 145 Degrees?

The value of sin 145 degrees in decimal is 0.573576436. . .. Sin 145 degrees can also be expressed using the equivalent of the given angle (145 degrees) in radians (2.53072 . . .).

We know, using degree to radian conversion, θ in radians = θ in degrees × (pi/180°)
⇒ 145 degrees = 145° × (π/180°) rad = 29π/36 or 2.5307 . . .
∴ sin 145° = sin(2.5307) = 0.5735764. . .

Explanation:

For sin 145 degrees, the angle 145° lies between 90° and 180° (Second Quadrant). Since sine function is positive in the second quadrant, thus sin 145° value = 0.5735764. . .
Since the sine function is a periodic function, we can represent sin 145° as, sin 145 degrees = sin(145° + n × 360°), n ∈ Z.
⇒ sin 145° = sin 505° = sin 865°, and so on.
Note: Since, sine is an odd function, the value of sin(-145°) = -sin(145°).

Methods to Find Value of Sin 145 Degrees

The sine function is positive in the 2nd quadrant. The value of sin 145° is given as 0.57357. . .. We can find the value of sin 145 degrees by:

• Using Trigonometric Functions
• Using Unit Circle

Sin 145° in Terms of Trigonometric Functions

Using trigonometry formulas, we can represent the sin 145 degrees as:

• ± √(1-cos²(145°))
• ± tan 145°/√(1 + tan²(145°))
• ± 1/√(1 + cot²(145°))
• ± √(sec²(145°) - 1)/sec 145°
• 1/cosec 145°

Note: Since 145° lies in the 2nd Quadrant, the final value of sin 145° will be positive.

We can use trigonometric identities to represent sin 145° as,

• sin(180° - 145°) = sin 35°
• -sin(180° + 145°) = -sin 325°
• cos(90° - 145°) = cos(-55°)
• -cos(90° + 145°) = -cos 235°

Sin 145 Degrees Using Unit Circle

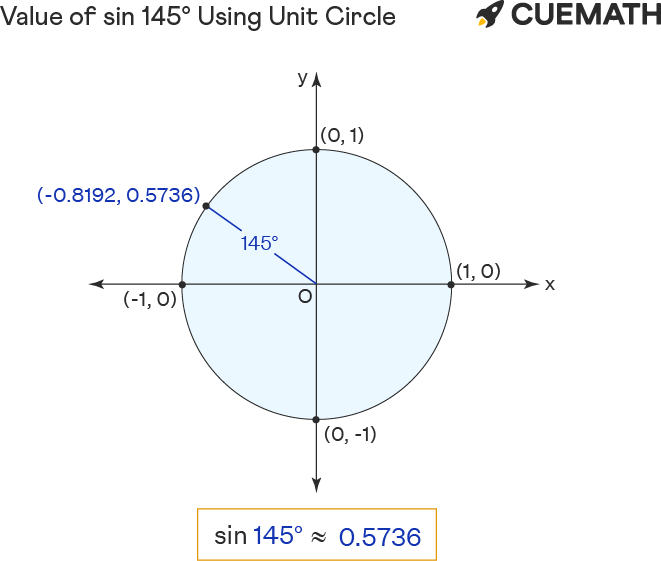
To find the value of sin 145 degrees using the unit circle:

• Rotate ‘r’ anticlockwise to form a 145° angle with the positive x-axis.
• The sin of 145 degrees equals the y-coordinate(0.5736) of the point of intersection (-0.8192, 0.5736) of unit circle and r.

Hence the value of sin 145° = y = 0.5736 (approx)

☛ Also Check:

• sin 10 degrees
• sin 36 degrees
• sin 270 degrees
• sin 330 degrees
• sin 43 degrees
• sin 44 degrees

FAQs on Sin 145 Degrees

What is Sin 145 Degrees?

Sin 145 degrees is the value of sine trigonometric function for an angle equal to 145 degrees. The value of sin 145° is 0.5736 (approx).

How to Find the Value of Sin 145 Degrees?

The value of sin 145 degrees can be calculated by constructing an angle of 145° with the x-axis, and then finding the coordinates of the corresponding point (-0.8192, 0.5736) on the unit circle. The value of sin 145° is equal to the y-coordinate (0.5736). ∴ sin 145° = 0.5736.

What is the Value of Sin 145° in Terms of Cosec 145°?

Since the cosecant function is the reciprocal of the sine function, we can write sin 145° as 1/cosec(145°). The value of cosec 145° is equal to 1.74344.

How to Find Sin 145° in Terms of Other Trigonometric Functions?

Using trigonometry formula, the value of sin 145° can be given in terms of other trigonometric functions as:

• ± √(1-cos²(145°))
• ± tan 145°/√(1 + tan²(145°))
• ± 1/√(1 + cot²(145°))
• ± √(sec²(145°) - 1)/sec 145°
• 1/cosec 145°

☛ Also check: trigonometric table

What is the Value of Sin 145 Degrees in Terms of Cos 145°?

Using trigonometric identities, we can write sin 145° in terms of cos 145° as, sin(145°) = √(1-cos²(145°)). Here, the value of cos 145° is equal to -0.8191520.