Cos 7 Degrees
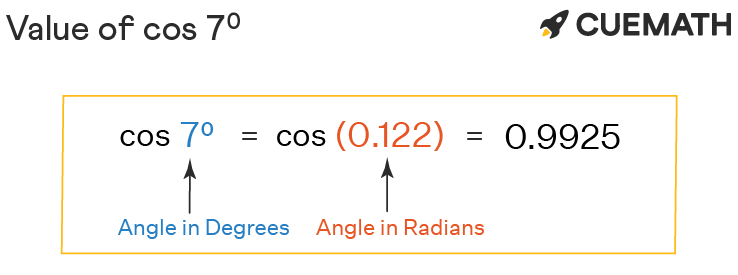
The value of cos 7 degrees is 0.9925461. . .. Cos 7 degrees in radians is written as cos (7° × π/180°), i.e., cos (0.122173. . .). In this article, we will discuss the methods to find the value of cos 7 degrees with examples.
- Cos 7°: 0.9925461. . .
- Cos (-7 degrees): 0.9925461. . .
- Cos 7° in radians: cos (0.1221730 . . .)
What is the Value of Cos 7 Degrees?
The value of cos 7 degrees in decimal is 0.992546151. . .. Cos 7 degrees can also be expressed using the equivalent of the given angle (7 degrees) in radians (0.12217 . . .)
We know, using degree to radian conversion, θ in radians = θ in degrees × (pi/180°)
⇒ 7 degrees = 7° × (π/180°) rad = 0.1221 . . .
∴ cos 7° = cos(0.1221) = 0.9925461. . .
Explanation:
For cos 7 degrees, the angle 7° lies between 0° and 90° (First Quadrant). Since cosine function is positive in the first quadrant, thus cos 7° value = 0.9925461. . .
Since the cosine function is a periodic function, we can represent cos 7° as, cos 7 degrees = cos(7° + n × 360°), n ∈ Z.
⇒ cos 7° = cos 367° = cos 727°, and so on.
Note: Since, cosine is an even function, the value of cos(-7°) = cos(7°).
Methods to Find Value of Cos 7 Degrees
The cosine function is positive in the 1st quadrant. The value of cos 7° is given as 0.99254. . .. We can find the value of cos 7 degrees by:
- Using Trigonometric Functions
- Using Unit Circle
Cos 7° in Terms of Trigonometric Functions
Using trigonometry formulas, we can represent the cos 7 degrees as:
- ± √(1-sin²(7°))
- ± 1/√(1 + tan²(7°))
- ± cot 7°/√(1 + cot²(7°))
- ±√(cosec²(7°) - 1)/cosec 7°
- 1/sec 7°
Note: Since 7° lies in the 1st Quadrant, the final value of cos 7° will be positive.
We can use trigonometric identities to represent cos 7° as,
- -cos(180° - 7°) = -cos 173°
- -cos(180° + 7°) = -cos 187°
- sin(90° + 7°) = sin 97°
- sin(90° - 7°) = sin 83°
Cos 7 Degrees Using Unit Circle
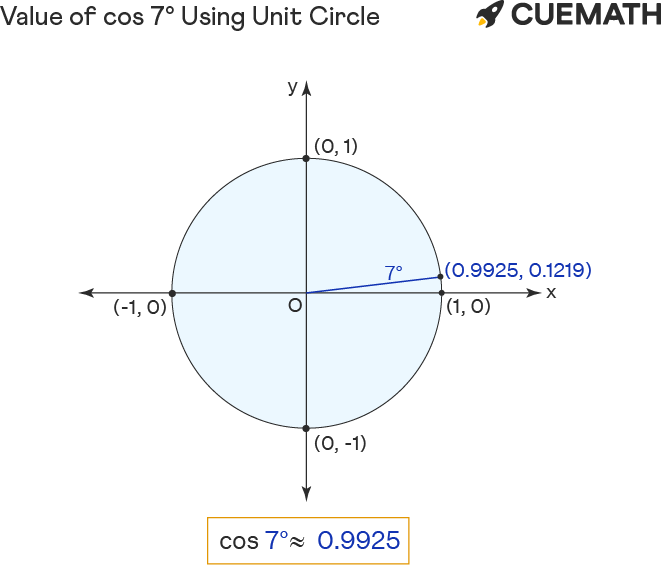
To find the value of cos 7 degrees using the unit circle:
- Rotate ‘r’ anticlockwise to form 7° angle with the positive x-axis.
- The cos of 7 degrees equals the x-coordinate(0.9925) of the point of intersection (0.9925, 0.1219) of unit circle and r.
Hence the value of cos 7° = x = 0.9925 (approx)
☛ Also Check:
FAQs on Cos 7 Degrees
What is Cos 7 Degrees?
Cos 7 degrees is the value of cosine trigonometric function for an angle equal to 7 degrees. The value of cos 7° is 0.9925 (approx)
What is the Value of Cos 7° in Terms of Sec 7°?
Since the secant function is the reciprocal of the cosine function, we can write cos 7° as 1/sec(7°). The value of sec 7° is equal to 1.007509.
How to Find Cos 7° in Terms of Other Trigonometric Functions?
Using trigonometry formula, the value of cos 7° can be given in terms of other trigonometric functions as:
- ± √(1-sin²(7°))
- ± 1/√(1 + tan²(7°))
- ± cot 7°/√(1 + cot²(7°))
- ± √(cosec²(7°) - 1)/cosec 7°
- 1/sec 7°
☛ Also check: trigonometry table
What is the Value of Cos 7 Degrees in Terms of Tan 7°?
We know, using trig identities, we can write cos 7° as 1/√(1 + tan²(7°)). Here, the value of tan 7° is equal to 0.122784.
How to Find the Value of Cos 7 Degrees?
The value of cos 7 degrees can be calculated by constructing an angle of 7° with the x-axis, and then finding the coordinates of the corresponding point (0.9925, 0.1219) on the unit circle. The value of cos 7° is equal to the x-coordinate (0.9925). ∴ cos 7° = 0.9925.