Tangent Circle Formula
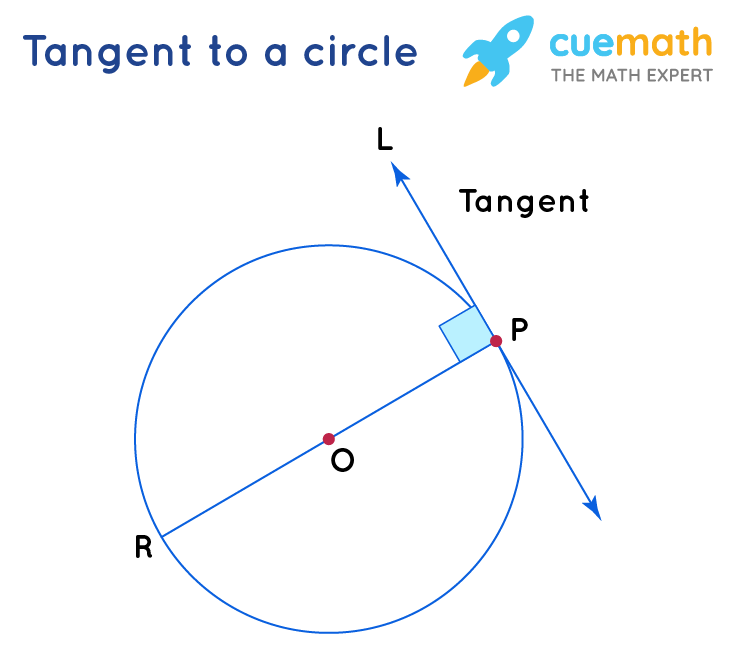
A tangent of a circle in geometry is defined as a straight line that touches the circle at only one point. A tangent never enters the circle’s interior.
The tangent has two important properties:
- A tangent touches a circle at exactly one point on it.
- The tangent touches the circle’s radius at a right angle.
What Is Tangent Circle Formula?
Let us now learn about the equation of the tangent. Tangent is a line and to write the equation of a line we need two things:
1. Slope (m)
2. A point on the line
General equation of the tangent to a circle:
1) The tangent to a circle equation x2 + y2 = a2 for a line y = mx +c is given by the equation y = mx ± a √[1+ m2].
2) The tangent to a circle equation x2+ y2 = a2 at (\(a_1, b_1)\) is x\(a_1\)+y\(b _1\)= a2
Thus, the equation of the tangent can be given as xa1+yb1 = a2, where (\(a_1, b_1)\) are the coordinates from which the tangent is made.

Let us now have a look at a few solved examples using the tangent circle formula.
Examples using Tangent Circle Formula
Example 1: Point (1,5) lies on a curve given by y=f(x)=x3-x+5. Find the equation of the tangent line to the curve that passes through the given point.
Solution: To write the equation of a line we need two things:
1. Slope
2. A point on the line
It is given that the curve contains a point (1,5)
The slope is the same as the slope of the curve at x=1 which is equal to the function’s derivative at that point:
f(x) = x3- x + 5
f'(x) = 3x2 - 1
f'(x) = 3(1)2 - 1 = 2
Substituting the slope m in the point-slope form of the line we would have:
\[\begin{align*} y-y_0 &= m_{tangent}(x-x_0)\\ y-5 &=2(x-1)\\ \end{align*}\]
Converting the above equation into \(y\)-intercept form as:
y-5 = 2(x-1)
y-5 = 2x-2
=2x+3
Answer: The equation for the tangent line is y=2x+ 3
Example 3: Find the equation to the pair of tangents drawn from the origin to the circle x2 + y2 - 4x - 4y + 7 = 0
Solution: We use the relation obtained in the last example, T2 = S\({S_1},\) to write the desired equation. Here, \(({x_1},{y_1})\) is (0, 0) while g = - 2,f = - 2 and c = 7. Thus the joint equation is
\[\begin{array}{l}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,&{T^2}(0,0) = S(x,y)S(0,0)\\\\ \Rightarrow & {( - 2x - 2y + 7)^2} = ({x^2} + {y^2} - 4x - 4y + 7)(7)\\\\ \Rightarrow & 4{x^2} + 4{y^2} + 49 + 8xy - 28x - 28y\\\\ &= 7{x^2} + 7{y^2} - 28x - 28y + 49\\\\ \Rightarrow & 3{x^2} - 8xy + 3{y^2} = 0\end{array}\]
Answer: As expected, since the tangents have been drawn from the origin, the obtained equation is a homogenous one.
FAQs on Tangent Circle Formula
What Is Tangent of the Circle Formula?
A tangent of a circle in geometry is defined as a straight line that touches the circle at only one point. The tangent formula is the tangent to circle equation which is y = mx ± a √[1+ m2], if the tangent is represented in the slope form and the tangent to the circle equation is x\(a_1\)+y\(b_1\)= a2 when tangent is given in the two-point form.
What Is the Tangent Circle Formula?
General equation of the tangent to a circle:
1) The tangent to a circle equation x2 + y2 = a2 for a line y = mx +c is given by the equation y = mx ± a √[1+ m2].
2) The tangent to a circle equation x2+ y2 = a2 at (\(a_1, b_1)\) is x\(a_1\)+y\(b_1)\)= a2
Thus, the equation of the tangent can be given as xa1+yb1 = a2, where (\(a_1, b_1)\) are the coordinates from which the tangent is made.
What Is the Equation of Tangent of Circle in Slope Form?
Equation of the tangent of slope 'm', to the circle x2 + y2 + 2gx + 2fy + c = 0 is given by (y + f) = m(x + g) ± r √[1+ m2, where r is the radius of the circle.
What Is the Equation of the Condition of Tangency?
A line will touch a circle when the distance of the center of the circle to the line is equal to the radius of the circle, i.e., if d = r and on squaring we obtain r2·(m2 + 1) = c2 the condition for a line y = mx + c to be a tangent to the circle x2 + y2 = r2.
visual curriculum