Sets Formulas

Set formulas are the formulas associated with set theory in mathematics. Set is a collection of well-defined objects and it has distinct elements. Knowledge of sets helps us apply the set formulas, in the areas related to statistics, probability, geometry, and sequences.

The set formulas include the union, intersection, complement, and difference of sets. Venn diagrams are popularly used to visualize set formulas to arrive at their proof. Let's learn about set formulas with a few solved examples.

What Are the Sets Formulas?

Set formulas have been derived from the set theory, and that can be used for ready reference. Let us recall the set notation, symbols, definitions, and properties of sets before the formula here.

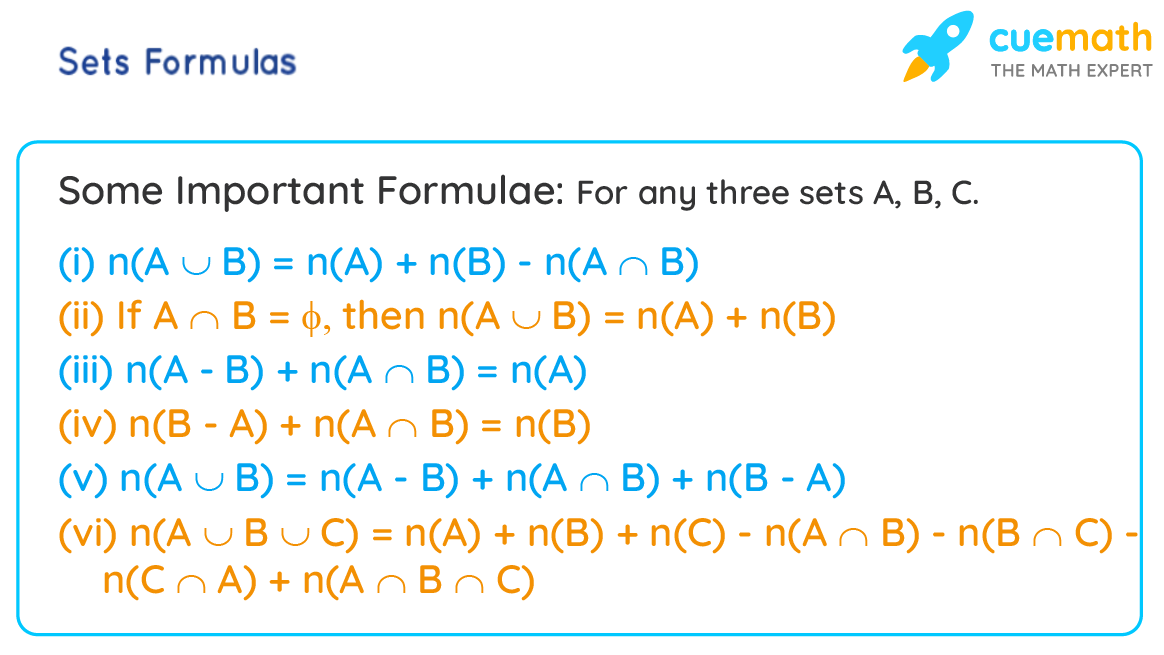
• If n(A) and n(B) denote the number of elements in two finite sets A and B respectively, then for any two overlapping sets A and B, n(A∪B) = n(A) + n(B) - n(A⋂B)
• If A and B are disjoint sets, n(A∪B) = n(A) + n(B)
• If A, B and C are 3 finite sets in U then, n(A∪B∪C)= n(A) +n(B) + n(C) - n(B⋂C) - n (A⋂ B)- n (A⋂C) + n(A⋂B⋂C)

Sets Formulas on Properties of Sets

Set formulas have almost similar properties as real numbers or natural numbers. The sets also follow the commutative property, associative property, distributive property. The set formula based on the properties of sets is as follows. 

Commutativity:

• A⋂B = B⋂A 
• A∪B = B∪A

Associativity:

• A⋂ (B⋂C) = (A⋂B)⋂C
• A∪ (B∪C) = (A∪B)∪C

Distributivity:A ⋂(B∪C) = (A ⋂B) ∪ (A⋂C)

Idempotent Law:

• A ⋂ A = A
• A ∪ A = A

Law of Ø and ∪:

•  A⋂ Ø = Ø
• U ⋂ A = A
• A ∪ Ø = A
• U ∪ A = U

Sets Formulas of Complement Sets

Set formulas for complement of a set include the basic complement law, the de morgan's laws, the double complement, and the law of empty set and universal set. 

• Complement Law : A∪A' = U, A⋂A' = Ø and A' = U -  A
• De Morgan's Laws: (A ∪B)' = A' ⋂B' and (A⋂B)' = A' ∪ B'
• Law of Double complementation: (A')' = A
• Laws of Empty set and Universal Set: Ø' = ∪ and ∪' = Ø

Sets Formulas of Difference of Sets

The set formulas or difference of sets across two sets, across a null set, and for complement of set is as follows.

• A - A = Ø
• B - A = B⋂ A'
• B - A = B - (A⋂B)
• (A - B) = A if A⋂B =  Ø
• (A - B) ⋂ C = (A⋂ C) - (B⋂C)
• A ΔB = (A-B) U (B- A) 
• n(AUB) = n(A - B) + n(B - A) + n(A⋂B)
• n(A - B) =  n(A∪B) - n(B)
• n(A - B) = n(A) - n(A⋂B)
• n(A^') = n(∪) - n(A)

Other Important Sets Formulas

• n(U) =  n(A) + n(B) + - n(A⋂B) + n((A∪B)^')
• n((A∪B)^') = n(U) +  n(A⋂B) - n(A) - n(B)

Let us have a look at a few solved examples to understand the sets formulas better.

Solved Examples Using Sets Formulas

Example 1: In a club, each person plays chess or carrom or both. The number of people who play chess, carrom or both are 11, 12 and 3 respectively. Representing this given information as sets and using the set formulae, find the people who play either chess or carrom?

Solution:

Let n(chess)= n(P) and n(chess) = n(Q)

Then we have n(either chess or carrom) = n(P∪Q) and n(chess and carrom) = n(P∩Q)

Given n(P) = 12 , n(Q) = 12 and (P∩Q) = 3

Applying the set formula,  n(P∪Q) = n(P) + n(Q) - n(P∩Q) = 11 + 12 - 3 = 20

Answer: The number of people who play both chess or carrom = 20

Example 2: In a class of 70 students, 45 students like to play soccer, 52 students like to play baseball. All the students like to play at least one of the two games. Using sets formula find how many students like to play soccer or baseball ? How many students like to play only soccer?

Solution:

Given: n(A U B ) = 70, n(A) = 45, n(B) = 52

We are required to find n (A ⋂ B)

Using sets formula, n(A ⋂ B)= n(A) + n(B) - n(A ∪ B)

n(A ⋂ B)= 45 + 52 - 70 = 27

Students who like to play only Soccer = 45 - 27 =18

Answer: 18 students like to play only soccer.

Example 3: There are 100 students, 35 like painting and 45 like dancing, and 10 like both. How many of the students like either of them or neither of them?

Solution:

Total number of students = 100

Number of students that like painting, n(P) = 35

Number of students that like dancing, n(D) = 45

Number of students who like both, n(P∩D) = 10

We are required to find n(A ∪ B) i.e., number of students who like either of them and 

Using sets formula, n(P∪D) = n(P) + n(D) – n(P∩D)

⇒ 45 + 35 - 10 = 70

Number of students who like neither = Total students – n(P∪D) = 100 – 70 = 30 

Answer: Therefore 70 students like either of them and 30 students like neither of them.

FAQs on Sets Formulas