

Remainder Theorem Formula
The remainder theorem formula is used to find the remainder when a polynomial is divided by a linear polynomial. Usually, we use the long division for finding the remainder when a polynomial is divided by the other polynomial. When the divisor polynomial is linear, we don't actually need to perform the long division to find the remainder, instead, we can use the remainder theorem formula.
What Is Remainder Theorem Formula?
The remainder theorem formula is used in the division of polynomials, especially to find the remainder when a polynomial is divided by a linear polynomial. Isn't it sounding great that we can find remainder without needing to use the long division process? Let us see the remainder theorem formula along with its proof.
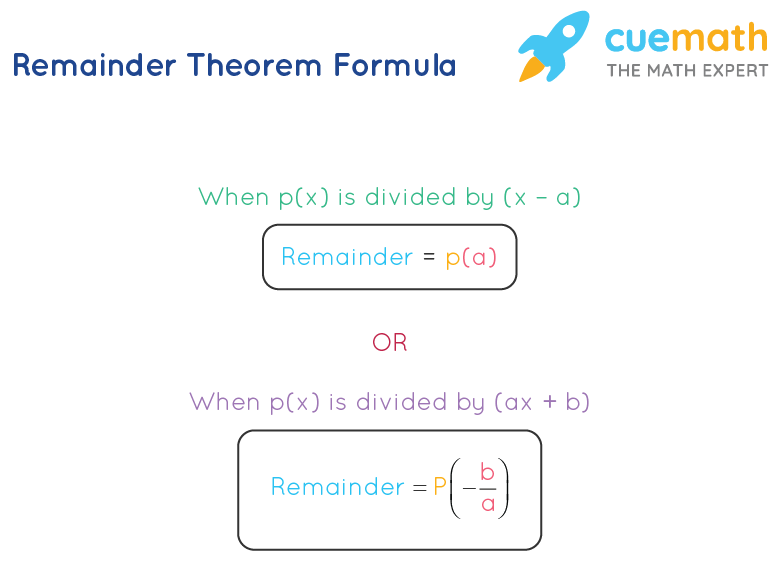
Remainder Theorem Formula
The remainder theorem states that "when a polynomial p(x) is divided by a linear polynomial (x - a), then the remainder is p(a)" (OR) "when a polynomial p(x) is divided by a linear polynomial (ax + b), the remainder is p(-b/a)". The remainder theorem is further used to deduce the factor theorem.
Remainder Theorem Formula Derivation
Let us assume that the quotient and the remainder when a polynomial p(x) is divided by a linear polynomial (x - a) are q(x) and r respectively. We know by Euclid's division lemma that:
Dividend = quotient × divisor + remainder
Here, p(x) = dividend, (x - a) = divisor, q(x) = quotient, and r = remainder.
So we get
p(x) = q(x) (x - a) + r
By substituting x = a,
p(a) = q(a) (a - a) + r
p(a) = q(a) (0) + r
p(a) = r
Thus, the remainder is p(a).
Hence, the remainder theorem formula is derived.

Let us see the applications of the remainder theorem formula in the upcoming section.
Examples Using Remainder Theorem Formula
Example 1: Write the remainders in each of the following cases: a) When f(x) is divided by (x - 2) b) When g(x) is divided by (2x - 1) c) When h(x) is divided by (3x+4).
Solution:
By the remainder theorem formula, the remainder when p(x) is divided by (ax + b) is p(-b/a). Thus:
a) x - 2 = 0 ⇒ x = 2
Thus, the remainder when f(x) is divided by (x - 2) is f(2).
b) 2x - 1 = 0 ⇒ x = 1/2
Thus, the remainder when g(x) is divided by (2x - 1) is g(1/2).
c) 3x + 4 = 0 ⇒ x = -4/3
Thus, the remainder when h(x) is divided by (3x + 4) is h(-4/3).
Answer: a) f(2); b) g(1/2); c) h(-4/3)
Example 2: Find the remainder when the polynomial p(x) = x4 + 2x3 - 4x - 3 is divided by (x - 3).
Solution:
x - 3 = 0 ⇒ x = 3
By the remainder theorem formula, the remainder when p(x) is divided by (x - a) is p(a). Thus the remainder when p(x) is divided by (x - 3) is,
Remainder = p(3)
= 34 + 2 (3)3 - 4(3) - 3
= 81 + 54 - 12 - 3
= 120
Answer: Remainder = 120.
Example 3: Determine whether (2x - 3) is a factor of p(x) = 2x3 + x2 + 4x -15.
Solution:
2x - 3 = 0 ⇒ x = 3/2
We will find the remainder when p(x) is divided by (2x - 3) using the remainder theorem formula and see whether the remainder is zero. If so, we can say that (2x - 3) is a factor of p(x).
Remainder = p(3/2)
= 2(3/2)3 + (3/2)2 + 4(3/2) -15
= 27/4 + 9/4 + 6 - 15
= 0
Answer: (2x - 3) is a factor of p(x).
FAQs on Remainder Theorem Formula
What Is Remainder Theorem Formula?
The remainder theorem formula says when a polynomial f(x) is divided by (x - a), the remainder is f(a). Thus, it is helpful to find the remainder when a polynomial is divided by a linear polynomial.
How To Derive the Remainder Theorem Formula?
Assume that we have to find the remainder r when a polynomial p(x) is divided by (x - a). Assume that the quotient, in this case, is q(x). We apply Euclid's division lemma in this case and then substitute x = a to derive the remainder theorem formula. For detailed derivation, we can see the "Remainder Theorem Formula Derivation" section of this page.
What are the Applications of Remainder Theorem Formula?
The main application of the remainder theorem formula is the factor theorem. While proving the factor theorem, we need the remainder theorem. The factor theorem says if the remainder obtained by dividing p(x) by (x - a) is 0, then (x - a) is a factor of p(x).
How To Use Remainder Theorem Formula?
The remainder theorem formula can be used to find the remainder when a polynomial p(x) is divided by (ax + b). By finding the remainder, we can determine whether (ax + b) is a factor of p(x) as well. If the remainder is 0, then (ax + b) is a factor of p(x), otherwise its not.
visual curriculum