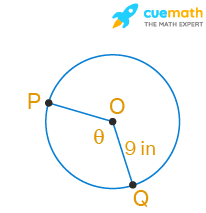
In circle O, radius OQ measures 9 inches and arc PQ measures 6π inches. Circle O is shown. Line segments PO and QO are radii with length of 9 inches. Angle POQ is theta. What is the measure, in radians, of central angle POQ?

Solution:

Given, the radius OQ of circle O measures 9 inches.

The arc PQ measures 6π inches.

We have to find the measure of central angle POQ in radians.

Central angle is the angle subtended by an arc of a circle at the center of a circle.

The formula to find central angle in radians is given by

Central Angle, θ = arc length / radius

Now, θ = 6π/9

= 2π/3

Therefore, the measure of central angle POQ is 2π/3 radians.

In circle O, radius OQ measures 9 inches and arc PQ measures 6π inches. Circle O is shown. Line segments PO and QO are radii with length of 9 inches. Angle POQ is theta. What is the measure, in radians, of central angle POQ?

Summary:

In circle O, radius OQ measures 9 inches and arc PQ measures 6π inches. Circle O is shown. Line segments PO and QO are radii with length of 9 inches. Angle POQ is theta. The measure, in radians, of central angle POQ is 2π/3.