For the function f(x) = -(x + 1)2 + 4, identify the vertex, domain, and range.
Solution:
Given, the function is f(x) = -(x + 1)2 + 4
We have to find the vertex, domain and range for the given function.
The vertex form of a quadratic function is given by
\(f(x)=(x-h)^{2}+k\)
Where, (h, k) is the vertex of the function.
The given function represents a vertical parabola open up, so the vertex is minimum.
From the function,
h = -1, k = 4
Therefore, the vertex is the point (-1, 4)
The domain is all real numbers in the interval \((-\infty,\infty )\)
The range is f(x) lessthan or equal to 4
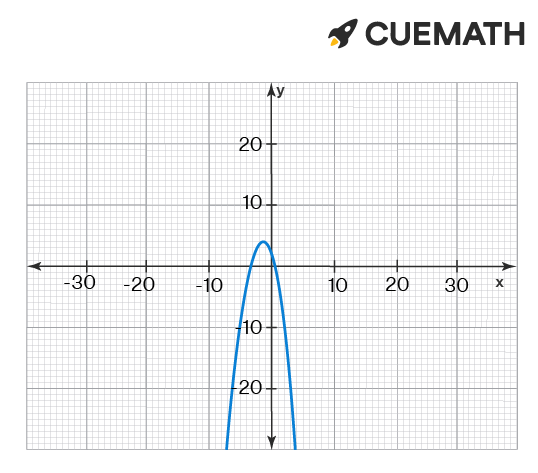
Therefore, the range lies in the interval ( -∞ , 4].
For the function f(x) = -(x + 1)2 + 4, identify the vertex, domain, and range.
Summary:
For the function f(x) = -(x + 1)2 + 4, vertex is (-1, 4), domain is all the real numbers in the interval \((-\infty,\infty )\), range lies in the interval ( -∞ , 4].