Perfect Square Trinomial Formula
The perfect square trinomial formula helps in solving the complex trinomial function. A perfect square trinomial function is the one that is obtained by squaring the binomial expression. A trinomial will be a perfect square if and only if it is in the form ax2 + bx + c and satisfies the condition b2 = 4ac. Let us understand the perfect square trinomial formula using solved examples.

What is Perfect Square Trinomial Formula?
When a binomial expression is squared, we get the trinomial which is a perfect square of the binomial. The perfect square trinomial formula can take two different forms. The forms that the perfect square trinomial formula represents are:
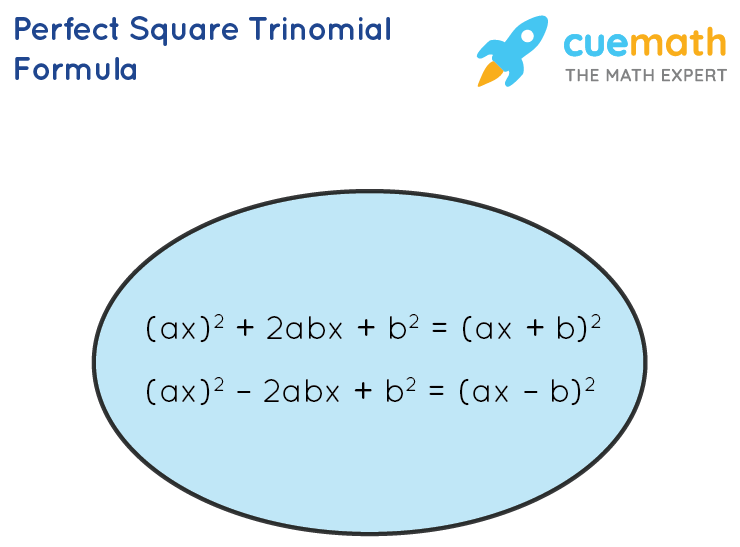
\((ax)^2 + 2abx + b^2 = (ax + b)^2\)
\((ax)^2 - 2abx + b^2 = (ax - b)^2\)
Let us understand the perfect square trinomial formula better using a few solved examples.
Solved Examples Using Perfect Square Trinomial Formula
-
Example 1: Find the factors of x2 + 6x + 9 using the perfect square trinomial formula.
Solution:
Writing the given expression as (ax)2 + 2abx + b2,
x2 + 6x + 9 = (1)x2 + 2(1)(3)x + 32Using the perfect square trinomial formula,
\((ax)^2 + 2abx + b^2 = (ax + b)^2\)
We get,
x2 + 6x + 9 = (x + 3)2Answer: Hence the factor of x2 + 6x + 9 is x + 3.
-
Example 2: Find the factors of x2 + 8x + 16 using the perfect square trinomial formula.
Solution:
Writing the given expression as (ax)2 + 2abx + b2,
x2 + 8x + 16 = (1)x2 + 2(1)(4)x + 42Using the perfect square trinomial formula,
\((ax)^2 + 2abx + b^2 = (ax + b)^2\)
We get,
x2 + 8x + 16 = (x + 4)2Answer: Hence the factor of x2 + 8x + 16 is x + 4.
visual curriculum