Percent Composition Formula
In the percent composition formula, we will learn about calculating the percent composition of a compound, i.e. what is the ratio of the quantity of elements present in terms of percentage. It is majorly used to find the percent composition of different compounds in the field of chemistry. Percent composition gives us the details of the amount of each element present in a compound in terms of mass percentage. The percentage composition formula helps us to calculate the mass percent of each element individually. Let's look into the percent composition formula in detail.

Formula to Calculate Percent Composition
The percent composition of an element is calculated by dividing the molar mass of that element by the total molecular mass of the compound. The percent composition formula is expressed as follows:
\(\%C_E = \dfrac{g_E}{g_T} \times 100\)
where,
- \(\%C_E\) = Percent composition of the element
- \(g_E\) = Mass of the element in one mole of compound
- \(g_T\) = Molar mass of the compound
Steps to Calculate the Percent Composition Formula
- Find the molar mass of all the individual compounds.
- Find the molecular mass of the compound.
- Divide the molar mass (of the specific element) by the molecular mass of the compound.
- Multiply the result by 100 to convert it into a percentage.
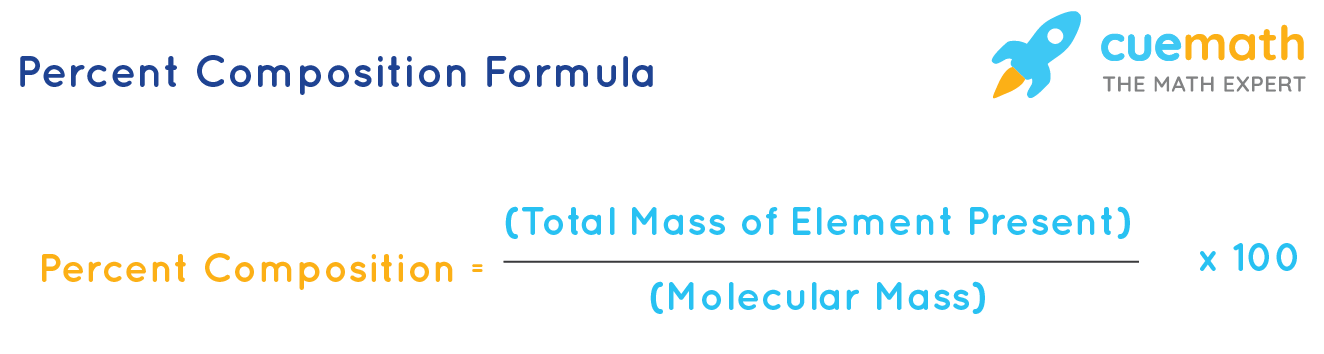
Let us see the applications of the percent composition formula by solving examples.
Solved Examples Using Percent Composition Formula
-
Example 1: Calculate the percent composition of each element in hydrochloric acid (HCl) using the percent composition formula.
Solution:
HCl is made up of Hydrogen (H) and Chlorine (Cl)
Molar mass of H = \(g_{(Hydrogen)}\) = 1 g
Molar mass of Cl = \({g_{(Chlorine)}}\) = 35.5 g
Molecular mass of HCl = \({g_T}\) = 1 + 35.5 = 36.6 g
Using percent composition formula,
Percent composition of hydrogen is:
%\(C_{(Hydrogen)}\) = \(g_{(Hydrogen)}\) / \(g_T\) × 100
= (1/36.5) × 100
= 2.74%
Percent composition of chlorine is:
%\(C_{(Hydrogen)}\) = 100% - %\(C_{(Hydrogen)}\)
= 100% - 2.74% = 97.26%
Answer: Thus, the percent composition of hydrogen and chlorine is 2.74% and 97.26% respectively.
-
Example 2: Find the percent composition of each element in water.
Solution:
\(H_2O\) is made up of hydrogen (H) and oxygen (O)
Molar mass of H = \(g_{(Hydrogen)}\) = 2 × 1 g = 2g (Since, 2 moles of hydrogen is present in water)
Molar mass of O = \(g_{(Oxygen)}\) = 16 g
Molecular mass of \(H_2O\) = gT = 2 + 16 = 18 g
Using percent composition formula,
Percent composition of hydrogen is:
%\(C_{(Hydrogen)}\) = \(g_{(Hydrogen)}\) / \(g_T\) × 100
= (2/18) × 100
= 11.11%
Percent composition of oxygen is:
%\(C_{(Oxygen)}\) = 100% - %\(C_{(Hydrogen)}\)
= 100% - 11.11% = 88.89%
Answer: Thus, the Percent Composition of hydrogen and oxygen is 11.11% and 88.89% respectively.
visual curriculum