Powers of iota
The value of iota, denoted as i, is √-1. This imaginary unit number is used to express complex numbers, where i is defined as imaginary or unit imaginary. Basically, the value of the imaginary unit number, i comes into the picture, when there is a negative number inside the square root, such that a unit imaginary number is equal to the root of -1. Therefore, the square of unit imaginary unit, i is equal to -1 and its cube is equal to the value -i. Similarly, we can evaluate other powers of iota by solving the expressions for different exponents. Higher power of i can be calculated by decomposing the higher exponents of i into smaller ones and thus evaluating the expression.
| 1. | What is Iota and its Value? |
| 2. | Powers of Iota |
| 3. | Square of Iota |
| 4. | Square Root of Iota |
| 5. | Higher Powers of Iota |
| 6. | Powers of Iota Calculator |
| 7. | FAQs |
What is Iota and Its Value?
Iota is an imaginary unit number that is denoted by i and the value of iota is √-1 i.e., i = √−1. While solving quadratic equations, you might have come across situations where the discriminant is negative. For example, consider the quadratic equation x2 + x + 1 = 0. If we use the quadratic formula to solve this, we get the discriminant (the part inside the square root) as a negative value.
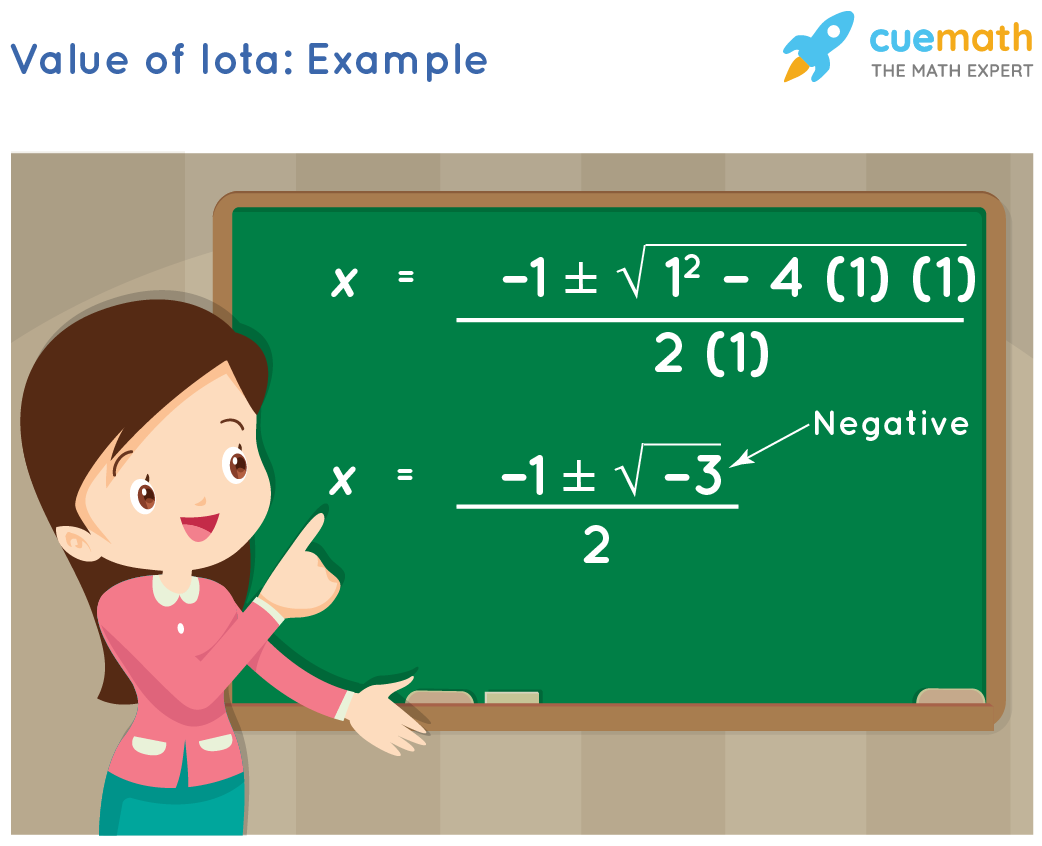
In such cases, we write √−3 as √−3 = √−1 × √3. This would give the solution of the above quadratic equation to be: x = (−1 ± √3\(i\))/2. Hence, the value of iota is helpful in solving square roots with negative values.
Thus, the value of iota is, i = √−1.
Power of i
The powers of i, i repeat in a certain pattern in a cycle. Let us start with calculating the value of powers of i for general cases and try to figure out the pattern.
Square of Iota
We know that the value of iota, i is defined as, i = √−1. If we square both sides of the above equation, we get: i2 = -1 i.e., the value of the square of iota is -1. Therefore, the square of iota is, i2 = −1.
Square Root of Iota
Iota has two square roots, just like all non-zero complex numbers. The value of the square root of iota, given as √i, can be calculated using De Moivre's Theorem.
We know that, i = cos(π/2) + isin(π/2)
= cos(π/2 + 2nπ) + isin(π/2 + 2nπ), n = 0, 1
= cos[(π + 4nπ)/2] + i sin[(π + 4nπ)/2]
Here, we took n = 0, 1 as we need 2 solutions. But we have to find √i = (i)1/2. Let us raise the exponent to 1/2 on both sides. So we get: √i = [cos{(π + 4nπ)/2} + isin{(π + 4nπ)/2}]1/2 = cos[(π + 4nπ)/4] + isin[(π + 4nπ)/4], n = 0, 1
- When n = 0, √\(i\) = cos(π/4) + isin(π/4) = √2/2 + i√2/2
- When n = 1, √\(i\) = cos(5π/4) + isin(5π/4) = −√2/2 − i√2/2
√i = √2/2 + i√2/2 = −√2/2 − i√2/2
Let us see how to calculate some other powers of i.
- i3 = i × i2 = i × −1 = −i
- i4 = i2 × i2 = −1×−1 = 1
- i5 = i × i4 = i × 1 = i
- i6 = i × i5 = i × i = i2 = −1
- i7 = i × i6 = i × −1 = -i
- i8 = ((i)2)4 = (−1)4 =1
- i9 = i × i8 = i × 1 = i
- i10 = i × i9 = i × i = i2 = −1
From the above calculations, we can observe that the values of iota repeat in certain pattern. The following figure represents the values for various powers of i in the form of a continuous circle.

This signifies that \(i\) repeats its values after every 4th power. We can generalize this fact to represent this pattern (where n is any integer), as,
- i4n = 1
- i4n+1 = i
- i4n+2 = -1
- i4n+3 = -i
Higher Power of i
Higher powers of iota can be calculated by decomposing the higher exponents \(i\) into smaller ones and thus evaluating the expression. Finding value if the power of i is a larger number using the previous procedure, will take quite some time and effort. If we observe all the powers of i and the pattern in which it repeats its values in the above equations, we can calculate the value of iota for higher powers as given below,
- Step 1: Divide the given power by 4.
- Step 2: Note the remainder for the division in Step 1, and use it as the new exponent/power of i.
- Step 3: Calculate the value of iota for this new exponent/power using the previously known values, i = √−1; i2 = -1 and i3 = -i.
Example: Find the value of i20296.

- We first divide 20296 by 4 and find the remainder.
- The remainder is 0 (by divisibility rules, we can just divide the number formed by the last two digits which is 96 in this case, to find the remainder).
- Thus, using the above rules, i20296 = i0 = 1
- Therefore, i20296 = 1
We just have to remember that i2 = -1 and i3 = -i. We will find some other higher powers of i using these and the above rules.
|
in |
Remainder n is divided by 4 |
Value |
|---|---|---|
|
i517 |
1 | i517 = i1 = i |
|
i2095 |
3 | i2095 = i2 = -i |
|
i23456 |
0 | i23456 = i0 = 1 |
|
i324770 |
2 | i324770 = i2 = -1 |
Value of Iota for Negative Power
The value of iota for negative power can be calculated following few steps. We first convert it into a positive exponent using the negative exponent law and then we apply the rule: 1/i = -i. This is because:1/i = 1/i • i/i = i/i2 = i/(-1) = -i
Example: Find the value of i-3927

i-3927 = 1/i3927
∵ a-m = 1/am
= 1/i3
∵ Remainder of 3927 divided by 4 is 3
= 1/-i
∵ i3 = -i
= --i
∵ 1/i = -i
= i
Power of i Calculator
Here is the "Powers of Iota Calculator".You can enter any exponent (positive or negative) of i and see the result in a step-by-step manner.
Important Notes
- The value of iota is i = √−1
- The value of the square of iota is, i2 = −1
- The value of the square root of iota is,
√i = √2/2 + i√2/2
Tips and Tricks
- To find any power of iota, say in, just divide n by 4 and find the remainder, r. Then just apply in = ir. Here, you just need to remember two things i2 = −1 and i3 = −i
- To calculate the negative powers of iota, we use the rule 1/i = −i
Solved Examples on Powers of Iota
-
Example 1: Find the power of i for the following: i37, i99, i(-1), i(-50)
Solution:
Since i2 = - 1, we have : i3 = i2 × i = - 1 × i = - i and i4 = ((i)2)2 = (-1)2 = 1. Now, we can calculate i raised to any integer power. For example,
(i)37 = (i)36 × (i) = ((i)4)9 × i = 1 × i = i
i99 = (i)96 × (i)3 = ((i)4)24 × i3 = 1 × −i = −i
i−1 = 1/i = i/i2 = i/(−1) = −i
\(i\)(−50) = 1/\(i\)50 = 1/(\(i\)48 × \(i\)2) = 1/\(i\)2= −1
Therefore, i37 = i, i99 = −i, i(−1) = −i, i(−50) = −1 -
Example 2: Find the value of i4n+k, where n and k are integers, and k is in the set {0, 1, 2, 3}.
Solution:
We have: i4n+k = i4n × ik = (i4)n × ik = 1 × ik = ik. Thus, the value of i4n+k is the same as the value of ik, which depends on the value of k:
k = 0: ik = 1
k = 1: ik = i
k = 2: \(i\)k = -1
k = 3: ik = -i
Therefore, k = 0: i4n+k = 1, k = 1: i4n+k = i , k = 2: i4n+k = -1, k = 3: i4n+k = -i -
Example 3: (a) Find the value of i500 + i501 + i502 + i503, (b) Show that the sum of any four consecutive powers of iota is 0.
Solution:
(a) We have the powers of ias follows.
i500 = ((i)4)125 = 1125 =1
i501 = i500 × i = i
i502 = i500 × i2 = i2 = −1
i503 = i500 × i3 = i3 = −i
Clearly, the sum of these four terms is 0
(b) We have: in + in+1 + in+2 + in+3 = in(1 + i + i2 + i3) = in(1 + i −1 −i) =0. Thus, i500 + i501 + i502 + i503 = 0. Therefore, the sum of any four consecutive powers of iota is 0.

Practice Questions on Powers of iota
FAQs on Powers of Iota
What is Iota in Mathematics?
Iota is an imaginary unit number to express complex numbers, where i is defined as imaginary or unit imaginary. Basically, the value of the imaginary unit number, i is generated, when there is a negative number inside the square root. Thus, the value of i is given as √-1.
What Is the Power Of i?
The power of i is the higher values of i such as i2 = -1, i3 = -i, i4 = 1. All of these powers of i can be generalized as i4n = 1, i4n + 1 = i, i4n + 2 = -1, i4n + 3 = -i.
What is the Value of i in Mathematics?
The value of the imaginary unit number, i is generated when there is a negative number inside the square root. The value of i in Mathematics is √−1.
What is Iota Cube?
Iota cube is given as i3, which can written as i3 = i2⋅i = (−1)⋅i = −i. Thus, the iota cube is −i.
Who Discovered Iota in Mathematics?
The concept of imaginary numbers in Mathematics was first introduced by mathematician “Euler”. He introduced i (read as 'iota') to represent √-1. Also, he defined i2 = -1.
What is the Symbol of Iota?
Iota is an imaginary unit number, denoted using the symbol i.
What is the Power of i to 34?
The value of i power 34 can be calculated as, i34 = ((i)4)8 • (i)2 = 1 × (-1) = -1. Therefore, value of (i)34 = -1.
What is Square Root of Iota?
The value of the square root of iota is, √i = √2/2 + i√2/2.
visual curriculum