Mixed Number to Improper Fraction
In order to convert a mixed number to an improper fraction, we need to multiply the whole number with the denominator and then add this product with the numerator. This forms the new numerator of the improper fraction while the denominator remains the same. The mixed number to improper fraction conversion can be done easily with the help of a few steps discussed on this page.
| 1. | Converting Mixed Number to Improper Fraction |
| 2. | How to Add Mixed Numbers to Improper Fractions? |
| 3. | FAQs on Mixed Number to Improper Fraction |
Converting Mixed Number to Improper Fraction
Before learning how to convert a mixed number to an improper fraction, let us quickly go through the definition of mixed numbers and improper fractions. A mixed fraction is one whose value is always greater than 1 and it has a whole number part and a proper fraction. A mixed number example is \(3\dfrac{2}{5}\) is a mixed number. An improper fraction is one in which the numerator is always greater than or equal to the denominator. Some examples of improper fractions are 4/3, 7/3, 11/5, etc.
Let us understand the method of converting a mixed number to an improper fraction with the help of an example. Let us convert the mixed fraction \(7\dfrac{1}{5}\) to an improper traction using the following steps:
- Step 1: Multiply the denominator with the whole number part. Here, 5 × 7 = 35.
- Step 2: Add the numerator to the product obtained in step 1. So, we get, 35 + 1= 36.
- Step 3: Write the value obtained in step 2 over the denominator. This will be the new numerator while the denominator will remain the same. So, \(7\dfrac{1}{5}\) = 36/5.
This is how we convert a mixed number to an improper fraction. Let us understand this with another example.
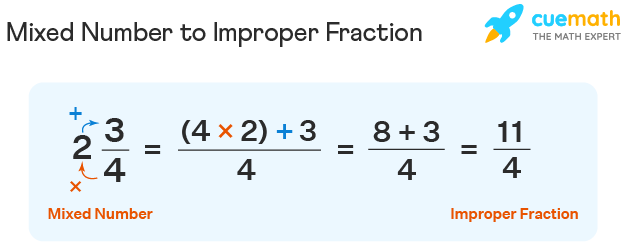
Example: Convert the mixed number to an improper fraction: \(2\dfrac{3}{4}\)
Solution: We can convert the mixed number to an improper fraction by using the following steps.
- Step 1: Let us multiply the denominator with the whole number part. Here, we will multiply 4 by 2, that is, 4 × 2 = 8.
- Step 2: Now, we will add this product to the numerator. This will be 8 + 3 = 11.
- Step 3: So, 11 will be the new numerator while the denominator (4) will remain the same. This means, \(2\dfrac{3}{4}\) = 11/4.
The other way to understand this process is the addition of the whole number part and the fractional part. For example, in the same example \(7\dfrac{1}{5}\), let us add the whole number (7) and the fraction (1/5). We get 7 + 1/5 = 7/1 + 1/5 = (35 + 1)/5 = 36/5. Therefore, this is another way to get the improper fraction from a mixed number.
How to Add Mixed Numbers to Improper Fractions?
In order to add mixed numbers to improper fractions, we first need to convert the mixed number to an improper fraction and then add them using the usual method of addition of fractions. If the given fractions are like fractions, then the addition can be done easily. However, if they are unlike fractions then they need to be converted to like fractions and then added. Let us understand this with the help of an example.
Example 1: Add \(3\dfrac{2}{5}\) + 14/5.
Solution: We will convert \(3\dfrac{2}{5}\) to an improper fraction which will be, 17/5. Now 17/5 + 14/5 = 31/5 = \(6\dfrac{1}{5}\). Therefore, the sum is \(6\dfrac{1}{5}\)
In case of unlike fractions, we need to find the Least Common Multiple (LCM) of the denominators and then convert them to like fractions. After this they can be added in the usual way.
☛ Related Topics
Mixed Number to Improper Fraction Examples
-
Example 1: Convert \(5\dfrac{2}{3}\) to an improper fraction.
Solution: In this question, a mixed number is given to us and we need to convert it into an improper fraction. Let us follow the steps given below to convert the mixed number to improper fraction:
- Step 1: Multiply 3 by 5 ⇒ 3 × 5 = 15.
- Step 2: Add 2 to 15 ⇒ 2 + 15 = 17.
- Step 3: Write 17 over 3. So, 17/3 is the answer.
Therefore, \(5\dfrac{2}{3}\) = 17/3.
-
Example 2: What improper fraction is equal to the mixed number \(4\dfrac{7}{9}\)?
Solution: To convert the given mixed number to an improper fraction, let us first multiply the denominator with the whole number. This means, 9 × 4 = 36. Then, let us add this product to the numerator, which is, 36 + 7 = 43. So, this will be the new numerator and the improper fraction will be = 43/9
Therefore, \(4\dfrac{7}{9}\) = 43/9.
-
Example 3: Which improper fraction is equal to the mixed number \(6\dfrac{4}{7}\)?
Solution: To convert the given mixed number to an improper fraction, let us follow the steps given below:
Step 1: Multiply 7 by 6 ⇒ 7 × 6 = 42.
Step 2: Add 4 to 42 ⇒ 4 + 42 = 46.
Step 3: Write 46 over 7. So, 46/7 is the answer.
Therefore, \(6\dfrac{4}{7}\) = 46/7.

FAQs on Mixed Number to Improper Fraction
What is the First Step in Changing a Mixed Number to an Improper Fraction?
The first step in changing a mixed number to an improper fraction is the multiplication of the whole number and the denominator of the given mixed number. Then, we add the numerator to the product.
What are the Steps to Convert a Mixed Number to an Improper Fraction?
The steps to convert a mixed fraction to improper fraction are given below. Let us convert \(3\dfrac{3}{7}\) into an improper fraction.
- Step 1: Find the product of the whole number and the denominator of the given mixed fraction. Here, 3 × 7 = 21.
- Step 2: Add the product to the numerator. So, 21 + 3 = 24.
- Step 3: Write that value over the denominator to express the answer. So, 24/7 is the improper fraction.
How to Change a Mixed Number to an Improper Fraction?
A mixed number consists of two parts - a whole number and a proper fraction. To change a mixed number to an improper fraction, we multiply the whole number by the denominator and then add this product with the numerator. This number becomes the numerator of the improper fraction and the denominator remains the same. For example, let us change the given mixed number to an improper fraction: \(3\dfrac{1}{2}\). We will first multiply the denominator (2) by the whole number (3) and the product is 2 × 3 = 6. To this product, we will add 1 which is the numerator. This will make it 6 + 1 = 7. So, 7 will become the numerator of the improper fraction and 2 will be the denominator. Therefore, \(3\dfrac{1}{2}\) is converted to an improper fraction and is written as 7/2.
How to Multiply a Mixed Number to an Improper Fraction?
To multiply a mixed number with an improper fraction, we first need to change the mixed fraction into an improper fraction. After this, we can multiply both the fractions in the usual way. For example, to multiply \(2\dfrac{3}{4}\) to 7/5, the first step is to convert \(2\dfrac{3}{4}\) to an improper fraction, which will be 11/4. Now, we can multiply 11/4 and 7/5 which is 11/4 × 7/5 = 77/20 = \(3\dfrac{17}{20}\).
How to Add Mixed Numbers to Improper Fractions?
In order to add mixed numbers to improper fractions, we first need to convert the mixed number to an improper fraction and then add them using the usual method of addition of fractions. For example, let us add \(5\dfrac{3}{4}\) + 15/4. We will convert \(5\dfrac{3}{4}\) to an improper fraction which will be, 23/4. Now 23/4 + 15/4 = 38/4 = 19/2 = \(9\dfrac{1}{2}\). Therefore, the sum is \(9\dfrac{1}{2}\)
visual curriculum
