Less Than or Equal To
Less than or equal to relation is one of the inequalities used to represent the relation between two numbers or two mathematical expressions. We know that the 'less than' symbol is used to show that one quantity is lesser than the other quantity, and the 'is equal to' symbol is used to show that two quantities are equal. Similarly, there is a symbol of less than or equal to in math which is used to show that one quantity can be less than the other quantity or equal to the other quantity.
| 1. | What is Less Than or Equal To? |
| 2. | Less Than or Equal to Symbol in Words |
| 3. | Less Than or Equal to Meaning |
| 4. | Less Than or Equal to on a Number Line |
| 5. | FAQs on Less Than or Equal to |
What is Less Than or Equal To?
'Less than or equal to', as the name suggests, means a variable is either less than or equal to another number, variable, or quantity. 'Less than or equal to' is used to represent the following phrases while solving math problems:
- at most
- no more than
- a maximum of
- not exceeding
Less than or equal to is represented by the sign "≤". Observe the following figure to see the symbol that shows a 'less than' sign with a sleeping line under it.
Less Than or Equal To Symbol
Less than or equal to is represented by the symbol "≤". Let us understand the usage of this symbol with an example. James works at a departmental store, and he is paid on an hourly basis. He can work for a maximum of 8 hours per day. Do you know what’s meant by the term maximum, here? This means James can work for either less than or equal to 8 hours per day in the store. Let us represent the number of hours James worked as x hours. Then we can write the given example mathematically as, x ≤ 8.
Less Than or Equal To and Greater Than or Equal To
An inequality symbol that is similar to "less than or equal to" is "greater than or equal to". It is represented by the symbol '≥'. We come across certain statements involving the signs '≤' and '≥' which are called inequalities. Both inequality signs have different meanings. We can easily understand them by comparison. Here are some comparisons of these symbols and their examples along with their meanings.
| Less Than or Equal To | Greater Than or Equal To | |
|---|---|---|
| Definition | A comparison that is true when the value on the left is less than or equal to the value on the right. | A comparison that is true when the value on the left is greater than or equal to the value on the right. |
| Example 1: | x ≤ 7 means the value of x is less than or equal to 7. | x ≥ 2 means the value of x is greater than or equal to 2. |
| Example 2: | − 5 ≤ x ≤ 3 means the value of x should lie between − 5 and 3, inclusive of both values. | 2 ≥ x ≥ − 1 means the value of x should lie between − 1 and 2, inclusive of both values. |
Less Than or Equal to on a Number Line
Inequalities like less than or equal to and greater than or equal to are represented in a different way on a number line. To denote these, we use the closed circle to mark the limit value and point the arrow (either to the left side or to the right side of the limit value) towards the given condition of inequality. Let us see this on a number line given below:
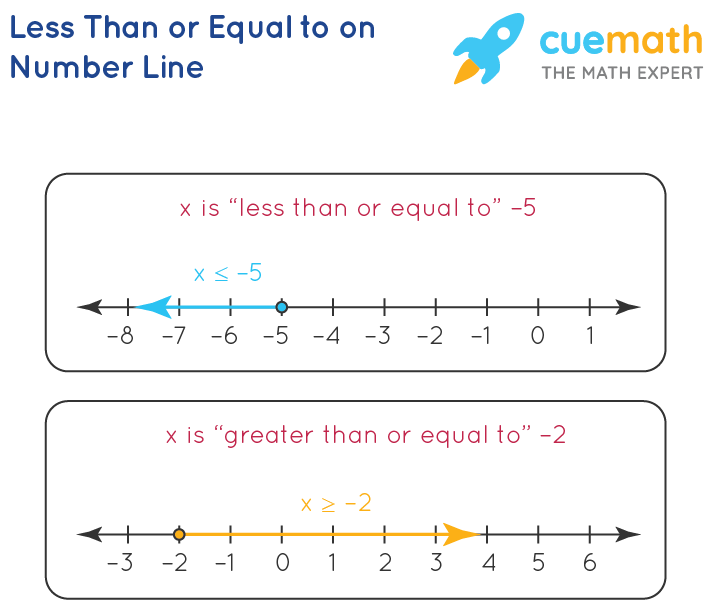
Here, the closed circles in both figures represent that "x can be equal to the limiting value also".
We can see that when we want to denote 'x less than or equal to - 5', we marked a circle at - 5 and pointed an arrow towards the values less than - 5, as suggested in the condition of inequality. Similarly, when we want to denote 'x greater than or equal to - 2', we marked a circle at - 2 and pointed an arrow towards the values greater than - 2, as suggested in the condition of inequality.
☛Related Topics:
- Greater than Less than Calculator
- Greater Than Calculator
- How To Make a Greater Than or Equal to Sign
Important Notes on Less Than or Equal To:
- Same numbers can either be added or subtracted on both sides of an inequality without changing the sign of inequality.
- Same positive numbers can either be multiplied or divided into both sides of an inequality without changing the sign of inequality.
- Same negative numbers can either be multiplied or divided on both sides of an inequality and the sign of inequality gets reversed.
Less Than or Equal To Examples
-
Example 3: Jolly's teacher asked her to find the numbers that belong to the set as shown on the blackboard.
Can you help her?
Solution:
We know that Z is the set of integers.
The given set is:
{x ∈ Z | x ≤ 4}
This means that we have to find all the integers that are less than or equal to 4.
Since the set of integers is infinite, the numbers that belong to the given set are:
..., −3, −2, −1, 0, 1, 2, 3, 4
Answer: The numbers in the given set are ..., −3, −2, −1, 0, 1, 2, 3, 4.
FAQs on Less than or Equal to
What is Less Than or Equal to in Math?
Less than or equal to is represented by the symbol ≤. So if we want to write x is less than or equal to 6 mathematically, we write it as x ≤ 6. It means that x can have any value from negative infinity to 6 but not more than 6.
What Does Less Than or Equal To Mean?
Less than or equal to in math means that you can't have more than something, you must have either less than or equal to the given limit. 'Less than or equal to', as the name suggests, means a number is either less than or equal to another number. It can also be expressed as at most, no more than, a maximum of, and not exceeding.
What is the Difference Between Less Than and Less Than or Equal To?
The inequality 'less than' is represented by the symbol < whereas the inequality 'less than or equal to' is represented as ≤. The inequality 'less than' means that some variable or number can have any value that is less than the given limit, not more than that or equal to that limit, but the inequality 'less than or equal to' states that the number or variable can be equal or less than the given limit. Here, the "inclusion of the limit" is the difference.
How Do You Use Less Than or Equal To?
Less than or equal to is used to express an inequality where a variable or an expression could be less than or equal to a number, variable, or expression. For example, a ≤ 2, x + y ≤ 6, p ≤ 6q + 8, etc.
What is Another Word for Less Than or Equal To?
In math, some phrases like "maximum", "not exceed", "at most", etc are used to represent "less than or equal to". This understanding helps in framing the correct inequality according to the given math problem.
What is an Example of Less Than or Equal To?
Consider the inequality a ≤ 5, where 'a' is a natural number. We know that the set of natural numbers is {1, 2, 3, 4, 5, 6, 7,....}. Since the inequality says a ≤ 5, the solutions of this inequality are 1, 2, 3, 4, and 5 as these are the only natural numbers that satisfy the inequality.