First 10 Natural Numbers
First ten natural numbers are a set of consecutive natural numbers from 1 to 10, with 1 as the natural number and 10 as the largest one. Natural numbers are also known as counting numbers and include all the positive integers from 1 to infinity. The set of natural numbers, as a whole, is a classification under the bigger set of real numbers not including zero, fractions, decimals, and negative numbers.
In this article, let's learn the first ten natural numbers, their sum, lcm, and average with formula and solved examples.
| 1. | List of First Ten Natural Numbers |
| 2. | Sum of First Ten Natural Numbers |
| 3. | First Ten Natural Numbers Average |
| 4. | LCM of First Ten Natural Numbers |
| 5. | FAQs on First Ten Natural Numbers |
List of First Ten Natural Numbers
List of first ten natural numbers refers to the list of consecutive natural numbers starting from 1 to 10 which is formed with the help of a simple formula, we need to add 1 to the previous number to obtain the next number. Thus, we can conclude that 10 is the last natural number in the list of the first ten natural numbers, that is from 1 to 10, and 1 is the smallest natural number in the list.
Natural numbers 1 to 10 are all those numbers, within this range, that are all consecutive numbers starting from 1 till 10. The list of natural numbers from 1 to 10 includes 1, 2, 3, 4, 5, 6, 7, 8, 9, and 10.
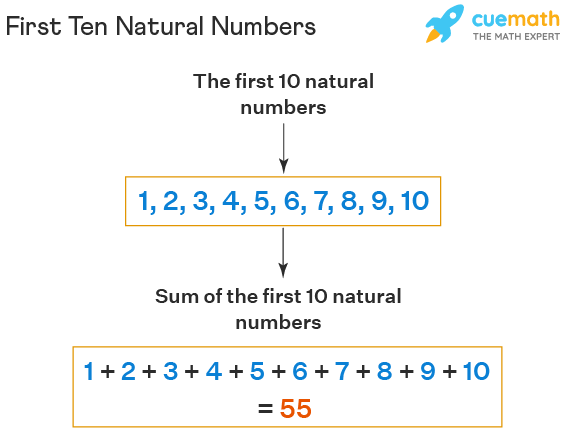
Sum of First Ten Natural Numbers
The first ten natural numbers can be written as 1, 2, 3, 4, 5…….10. Clearly, this forms an arithmetic progression (A.P). Applying the arithmetic progression formula of the sum of a.p., the sum of all natural numbers 1 to 10 can be calculated using the formula, S= n/2[2a + (n − 1) × d], where, a is the first term, d is the difference between the two consecutive terms, and n is the total number of natural numbers from 1 to 10. There are a total of 10 natural numbers in the list or arithmetic progression, so n = 10.
Thus, a = 1, d = 1 and n = 10
Let's calculate the sum of the first ten natural numbers.
The sum of an A.P: 1, 2, 3, 4,5…….10.
S = n/2[2a + (n − 1) × d]
S = 10/2[2.1 + (10 − 1) × 1]
S = 10/2[2 + 9]
S = 5[11] = 55.
Therefore, the sum of the first ten natural numbers is 55.
First Ten Natural Numbers Average
First ten natural numbers average corresponds to the arithmetic mean of the numbers from 1 to 10. The average is the sum of all numbers in a collection, divided by the count of the numbers present in the collection. In other words, it is the ratio of the sum of all given observations to the total number of observations.
- Step 1: Sum of the Numbers: 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10 = 55
- Step 2: Number of Observations: 10
- Step 3: Average Calculation, Average = Sum of the Observations/Number of Observations = 55/10 = 5.5
Therefore, the average of the first ten natural numbers is 5.5.
LCM of First Ten Natural Numbers
The LCM of the first 10 natural numbers is the least number which is exactly divisible by all the 10 numbers. Since, the first ten natural numbers are 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, the LCM of all these numbers is the product of all the prime factors so obtained after taking lcm. Now, finding the LCM of (1, 2, 3, 4, 5, 6, 7, 8, 9, 10) by the division method
In the division method, we first arrange the numbers in the grid and then divide the given numbers with their prime factors till we cannot find any prime number to further divide the given numbers without leaving any remainder. The LCM is the product of all such common prime factors.
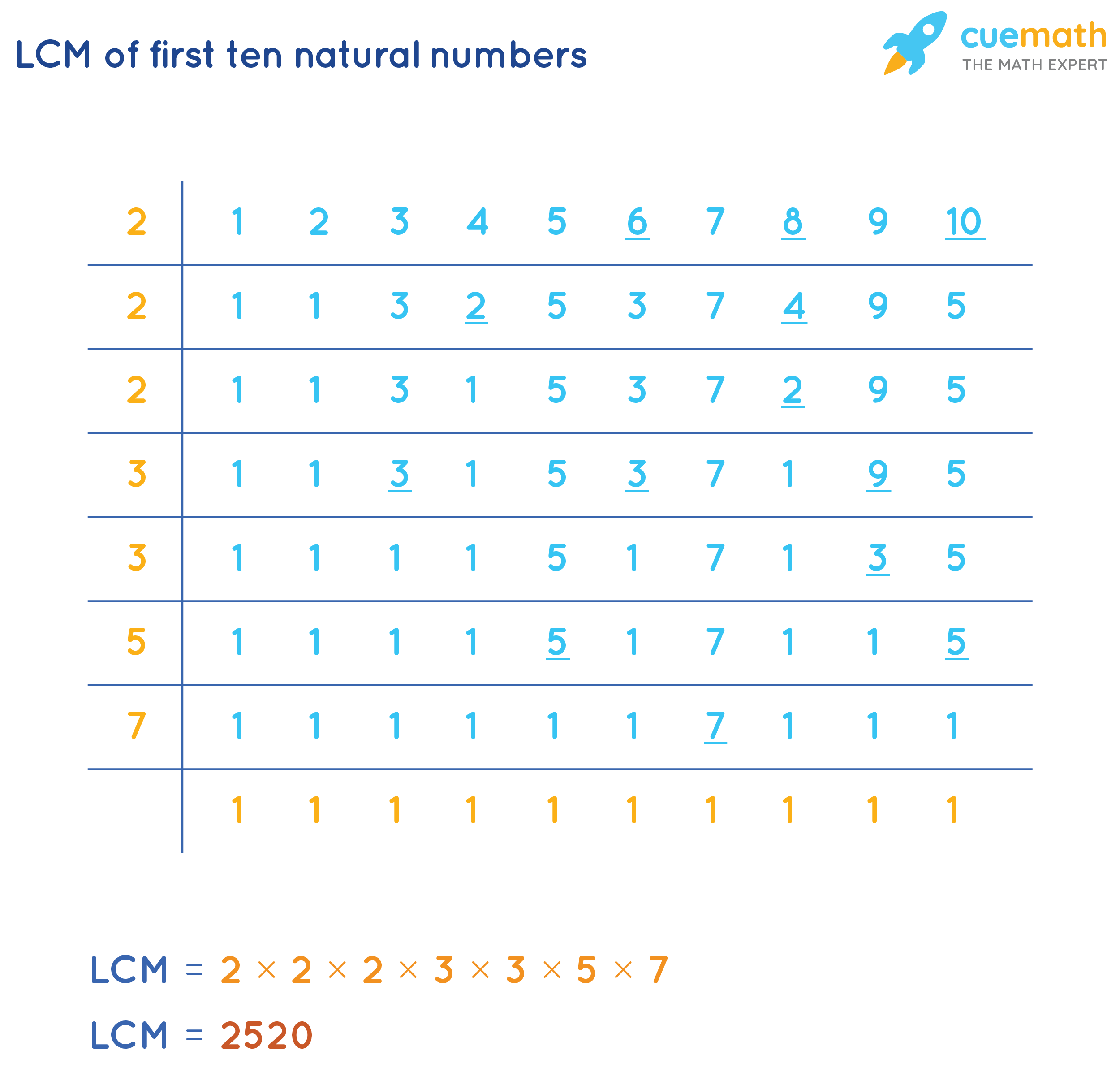
Thus, the LCM of the first ten natural numbers is 2520.
Important Notes:
- 1 is the smallest amongst the list of the first ten natural numbers.
- There are a total of 10 natural numbers in the list from 1 to 10.
- The sum of the first ten natural numbers, that is from 1 to 10 is 55.
- The average or mean of the numbers from 1 to 10 is 5.5.
Topics Related to First Ten Natural Numbers
Check these articles related to the concept of first ten natural numbers.
Examples on First Ten Natural Numbers
-
Example 1: Determine the sum of the largest and the smallest natural numbers from the list of the first ten natural numbers.
Solution:
As per the list of the first ten natural numbers, which includes numbers from 1 to 10, the largest even number is 10 and the smallest even number is 1. Thus, the required sum is 10+1 = 101. Therefore, the sum of the largest and the smallest natural numbers from the list of the first ten natural numbers is 101.
-
Example 2: How many odd numbers are there in the list of the first ten natural numbers?
Solution:
We know that the odd numbers are the ones that are not divisible by 2 and end up with 1, 3, 5, 7, and 9 as the last digits (or digit at unit place) of the given numbers. From the list of the first ten natural numbers, which includes numbers from 1 to 10, the odd numbers are 1, 3, 5, 7, and 9. Thus, there are 5 odd odd numbers in the list of first ten natural numbers.

FAQs on First Ten Natural Numbers
What Are First Ten Natural Numbers?
The first ten natural numbers refer to the list of natural numbers starting from 1 to 10. The first ten natural numbers are 1, 2, 3, 4, 5, 6, 7, 8, 9, and 10. Each of the two consecutive numbers have a difference of 1.
What Is the Sum of First Ten Natural Numbers?
The first ten natural numbers can be written as an arithmetic sequence - 1, 2, 3, 4, 5…….10. Thus, the sum of all natural numbers 1 to 10 can be calculated using the formula, S= n/2[2a + (n − 1) × d], where, a is the first term, d is the difference between the two consecutive terms, and n is the total number of natural numbers from 1 to 10. Therefore, the sum of the first ten natural numbers is 55.
What Is the Mean of First Ten Natural Numbers?
The mean of the first ten natural numbers is the sum of all numbers in the list (1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10), divided by the count of the numbers (10). Here, the sum of the numbers = 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10 = 55
and the number of observations is 10. Thus, the mean equals to the ratio 55/10 or is simply 5.5.
What Is the Variance of First Ten Natural Numbers?
The variance of the first ten natural numbers can be calculated using the variance formula, \(\sigma^{2}=\frac{\sum\left(x_{i}-\mu\right)^{2}}{N}\). We know that the mean of the first ten natural numbers is 5.5. Now, substituting the values in the formula, we have var = [(−4.5)2+(−3.5)2++(−2.5)2+(−1.5)2+(−.5)2+(.5)2+(1.5)2+(2.5)2+(3.5)2+(4.5)2] / 10⇒ var = [20.25+12.25+6.252.25+0.25+0.25+2.25+6.2512.25+20.25]/10 = 82.5/10 = 8.25.
What Is the Median of First Ten Natural Numbers?
The median of first ten natural numbers can be calculated using the median formula for even number of observations as n = 10, (\text { Median }=\frac{\left(\frac{n}{2}+1\right)^{t h} \text { term }+\left(\frac{n}{2}\right)^{t h} \text { term }}{2}\). Thus, substituting the values in the formula, we have median = (6 + 5) / 2 = 11/2 = 5.5.
What Is the Standard Deviation of First Ten Natural Numbers?
The standard deviation of the first ten natural numbers can be calculated using the standard deviation formula, σ = √var. We know that the variance of the first ten natural numbers is 8.25. Thus, standard deviation, σ = √var = √8.25 = 2.87.
What Percent of the First 10 Natural Numbers Are Prime Numbers?
There are a total of 4 prime numbers, which includes 2, 3, 5, and 7, in the list of the first ten natural numbers and there are a total of 10 numbers in the list. Thus, the percent of the prime numbers in the first ten natural numbers equals (4/10) ×100 = 40%.
visual curriculum