Factors of 65
Factors of 65 are the numbers which when multiplied in pairs give the product as 65. These factors can be negative as well. 65 is a special two-digit number because apart from 1 and 65, it has only one other pair factor, i.e. 5 and 13. In this lesson, we will learn the factors of 65, the prime factors of 65, and the factors of 65 in pairs along with solved examples.
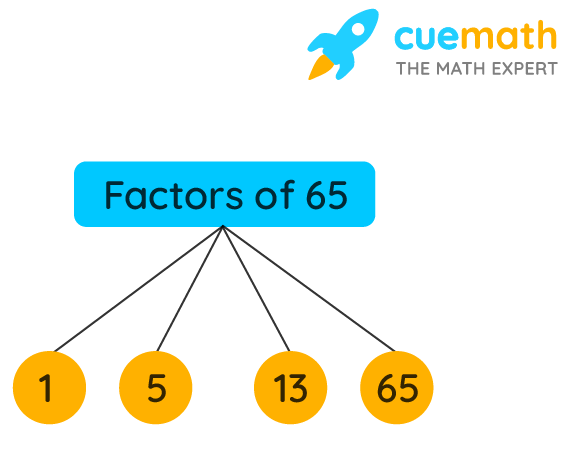
- Factors of 65: 1, 5, 13, and 65
- Prime Factorization of 65: 65 = 5 × 13
| 1. | What Are the Factors of 65? |
| 2. | How to Calculate the Factors of 65? |
| 3. | Factors of 65 by Prime Factorization |
| 4. | Factors of 65 in Pairs |
| 5. | FAQs on Factors of 65 |
What Are the Factors of 65?
Let us first understand the meaning of factors. A factor is that number which divides any given number without leaving a remainder at the end. The number 65 is said to be an odd composite number. A composite number is a number that consists of more than two factors. For instance, consider the number 65. The factors of 65 are 1, 5, 13, and 65.
How to Calculate the Factors of 65?
Let us begin calculating the factors of 65.
We start with the number 1.
Divide 65 with 1. Is the remainder zero?
Yes! By factor definition, the number 65 is divided equally by 1 without leaving a remainder.
- 65 ÷ 1 = 65
- 65 × 1 = 65
Next, let's try the number 2. Since 65 is an odd number, it cannot be divided by 2 or multiples of 2. Hence, we need to check odd numbers only. Let's try with number 3.
65 ÷ 3 = 21.66
Therefore, 3 is not a factor of 65.
Now, let's try with number 5.
- 65 ÷ 5 = 13
- 5 × 13 = 65
Factors of 65 By Prime Factorization
Prime factorization is the process of breaking down a composite number into its prime factors.
To get the prime factorization of 65, we divide it by its smallest prime factor which is 5.
- 65 ÷ 5 = 13
- 13 is a prime number whose factors are 1 and 13.
- The process of prime factorization goes on till we get the quotient as 1.
- The prime factorization of 65 is shown below:

Prime factorization of 65 can also be represented as follows:
- 65 = 5 × 13 × 1
Now that we have done the prime factorization of our number, we can multiply them and get the other factors. Can you try and find out if all the factors are covered or not?
And as you might have already guessed, for prime numbers, there are no other factors.
Explore factors using illustrations and interactive examples
- Factors of 62 - The factors of 62 are, 1, 2, 31, and 62
- Factors of 63 - The factors of 63 are 1, 3, 7, 9, 21 and 63
- Factors of 69 - The factors of 69 are 1, 3, 23 and 69.
- Factors of 66 - The factors of 66 are 1, 2, 3, 6, 11, and 66
- Factors of 68 - The factors of 68 are 1, 2, 4, 17, 34, and 68
Challenging Question:
- Mike has to divide 65 students in his class into different groups with the same number of students in all the groups. Each group must have more than one student and not all students can be in one group. In how many ways can Mike form these groups?
Factors of 65 in Pairs
- The pair of numbers that give 65 when multiplied with each other is called the pair factors of 65.
- Therefore, the pair factors of 65 are (1,65) and (5,13).
- Since the product of two negative numbers is positive, i.e. (-) × (-) = (+), (-1,-65), (-5,-13) are also factor pairs of 65.
Important Notes:
- The numbers which we multiply to get 65 are the factors of 65.
- The factors of 65 are 1, 5, 13, and 65.
- As the number 65 is an odd composite number, all of its factors will also be odd.
- The number 65 is neither a perfect square nor a perfect cube.
Factors of 65 Solved Examples
-
Example 1: Anna has 65 pencils in 5 different colors. She must divide the pencils and put them in several pouches. The pencils must be divided in such a way that the pouch does not have any repeated colors. How should Anna divide the pencils equally and how many pouches will she need?
Solution:
There are 65 pencils in total.
She can divide them into 13 sets with 5 pencils in each set so that the same colors are not repeated.
Since there are 13 sets, she will need 13 pouches. -
Example 2: Can you help Andrea list the factors of 65?
Solution:
Factors of 65 are the numbers that divide 65 exactly without any remainder.
65 ÷ 5 = 13
5 × 13 = 65
Hence, the factors of 65 are 1, 5, 13, and 65. -
Example 3: Miranda has 65 pairs of sandals. How many big or small cartons are needed so that the sandal pairs can be packed equally?
Solution:
Miranda has two possible ways to pack her sandals.
She can opt for either 65 ÷ 5 = 13 small cartons or 65 ÷ 13 = 5 big cartons
Miranda needs either 13 small cartons or 5 big cartons.
go to slidego to slidego to slide

FAQs on Factors of 65
What are the factors of 65?
The factors of 65 are 1, 5, 13, and 65.
Is 65 prime or composite?
The number 65 is an odd composite number since the number has more than two factors.
Which is the smallest factor of 65?
The smallest factor of 65 is 1.
Which is the smallest prime factor of 65?
The smallest prime factor of 65 is 5.
What are the prime factors of 65?
The prime factors of 65 are 5 and 13.
visual curriculum
