Factors of 132
Did you know from the number 132, if you take the sum of all the 2-digit numbers, you get 12 + 13 + 21 + 23 + 31 + 32 = 132 making it the smallest number among 264, 396, and 35964 with this property? In this lesson, we will find the factors of 132, prime factors of 132, and factors of 132 in pairs along with solved examples for a better understanding.
- Factors of 132: 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132
- Prime Factorization of 132: 132 = 2 × 2 × 3 × 11
| 1. | What Are the Factors of 132? |
| 2. | How to Calculate Factors of 132? |
| 3. | Factors of 132 by Prime Factorization |
| 4. | Factors of 132 in Pairs |
| 5. | FAQs on Factors of 132 |
What are the Factors of 132?
Factors of a number are the numbers that divide the given number exactly without any remainder. Factors of 132 are 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132.
So,132 is a composite number as it has more factors other than 1 and itself.
How to Calculate the Factors of 132?
We can use different methods like divisibility test, prime factorization, and the upside-down division method to calculate the factors of 132. We express 132 as a product of its prime factors in the prime factorization method as well as we divide 132 with its divisors in the division method. Let us see which numbers divide 132 exactly without a remainder.
Hence, the factors of 132 are 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132.
Explore factors using illustrations and interactive examples.
- Factors of 12 - The factors of 12 are 1, 2, 3, 4, 6, 12
- Factors of 150 - The factors of 150 are 1, 2, 3, 5, 6, 10, 15, 25, 30, 50, 75, 150
- Factors of 33 - The factors of 33 are 1, 3, 11, 33
- Factors of 32 - The factors of 32 are 1, 2, 4, 8, 16, 32
- Factors of 24 - The factors of 24 are 1, 2, 3, 4, 6, 8, 12, 24
- Factors of 66 - The factors of 66 are 1, 2, 3, 6, 11, 66
Factors of 132 by Prime Factorization
We can do prime factorization of any number by:
- Upside-down division method
- Factor tree method
Prime Factorization by Division Method
The upside-down division got its name because the division symbol is flipped upside down.
- STEP 1: By using divisibility rules, we find out the smallest exact prime divisor (factor) of the given number. Here, 132 is an even number. So it is divisible by 2. In other words, 2 divides 132 with no remainder. Therefore 2 is the smallest prime factor of 132.
- STEP 2: We divide the given number by its smallest factor other than 1 (prime factor), 132/2 = 66
- STEP 3: We then find the prime factors of the obtained quotient.Repeat Step 1 and Step 2 till we get a prime number as a quotient. In the last step, 33 is the quotient, 33/3= 11. 11 is the quotient here, so we stop the process as 11 is a prime number.
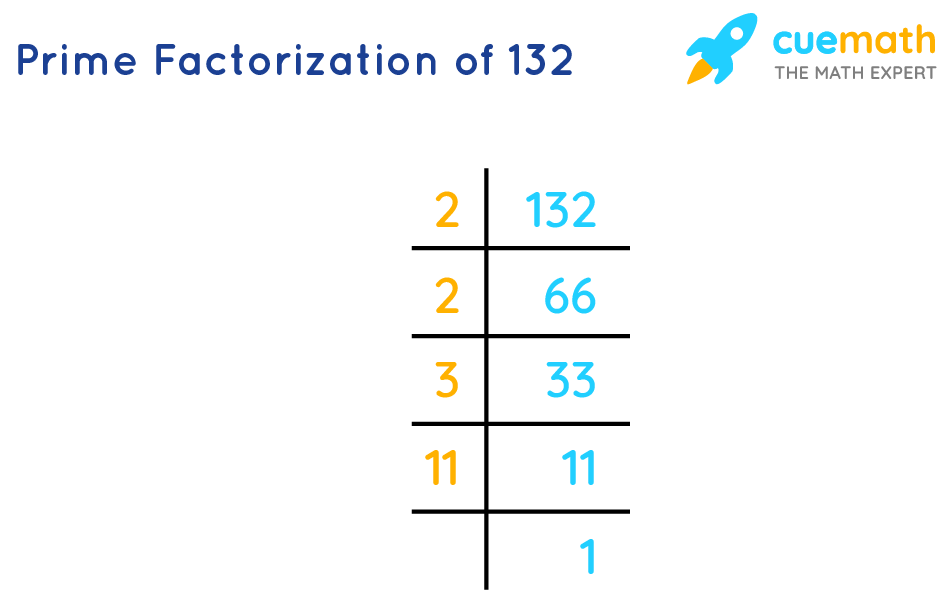
Therefore 132 = 2 × 2 × 3 × 11.
Prime Factorization by Factor Tree Method
First, we identify the two factors that give 132. 132 is the root of this factor tree.
132 = 2 × 66
Here, 66 is an even composite number, so it can be further factorized.
66 = 2 × 66
We continue this process until we are left with only prime numbers, i.e., till we cannot further factor the obtained numbers.
Basically, we branch out 132 into its prime factors. Factor tree is not unique for a given number.
Instead of expressing 132 as 2 × 66, we can express 132 as 4 × 33 or 3 × 44
Here is a simple activity to try it on your own, instead of 2 × 66, if we had used 3 × 44, do you think we will get the same factor tree?
Can you draw the factor tree with 3 and 44 as the branches?
Factors of 132 in Pairs
Factor pairs are the two numbers that, when multiplied, give the number 132.
| Factor Pairs of 132 | Notation |
|---|---|
| 1 ×132 | (1, 132) |
| 2 × 66 | (2, 66) |
| 3 × 44 | (3, 44) |
| 4 × 33 | (4, 33) |
| 6 × 22 | (6, 22) |
| 11 × 12 | (11, 12) |
- Therefore, factors of 132 in pairs are (1, 132), (2, 66), (3, 44), (4, 33), (6, 22), and (11, 12).
- We can have negative factors also for a given number.
- As the product of two negative numbers is positive (- × - = +), hence (-1, -132), (-2, -66), and (-3, -44), etc., are also factor pairs of 132
But, for now, let us focus on the positive factors in this article. With factors, we are only looking for whole numbers that are equal to or less than the original number.
Important Notes:
- 132 is composite number with factors 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132
- It can be expressed as the product of two consecutive numbers: 132 = 11 × 12
- Prime factorization of 132: 2 × 2 × 3 × 11
Think Tank:
- At a jamboree, there are 132 girl guides and 150 boy scouts. The leaders want to make teams of 12 for the raft race. Each team must have the same number of boys and girls. Can this be done? How?
FAQs on Factors of 132
What are the factors of 132?
The factors of 132 are, 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132.
What are the prime factors of 132?
The prime factors of 132 are 2, 3, and 11
Is 132 a perfect square?
No, 132 is not a perfect square. It cannot be expressed as a product of two equal numbers.
How many positive factors of 132 are there?
The factors of 132 are 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132.
In total, there are 12 positive factors of 132.
What are the common factors of 132 and 121?
The factors of 132 are 1, 2, 3, 4, 6, 11, 12, 22, 33, 44, 66, and 132. The factors of 121 are 1, 11, 121.
Hence, the common factors of 132 and 121 are 1 and 11.