Factors of 1080 are the list of integers that we can split evenly into 1080. There are 32 factors of 1080, of which the following are its prime factors 2, 3, 5. The Prime Factorization of 1080 is 2³ × 3³ × 5¹.

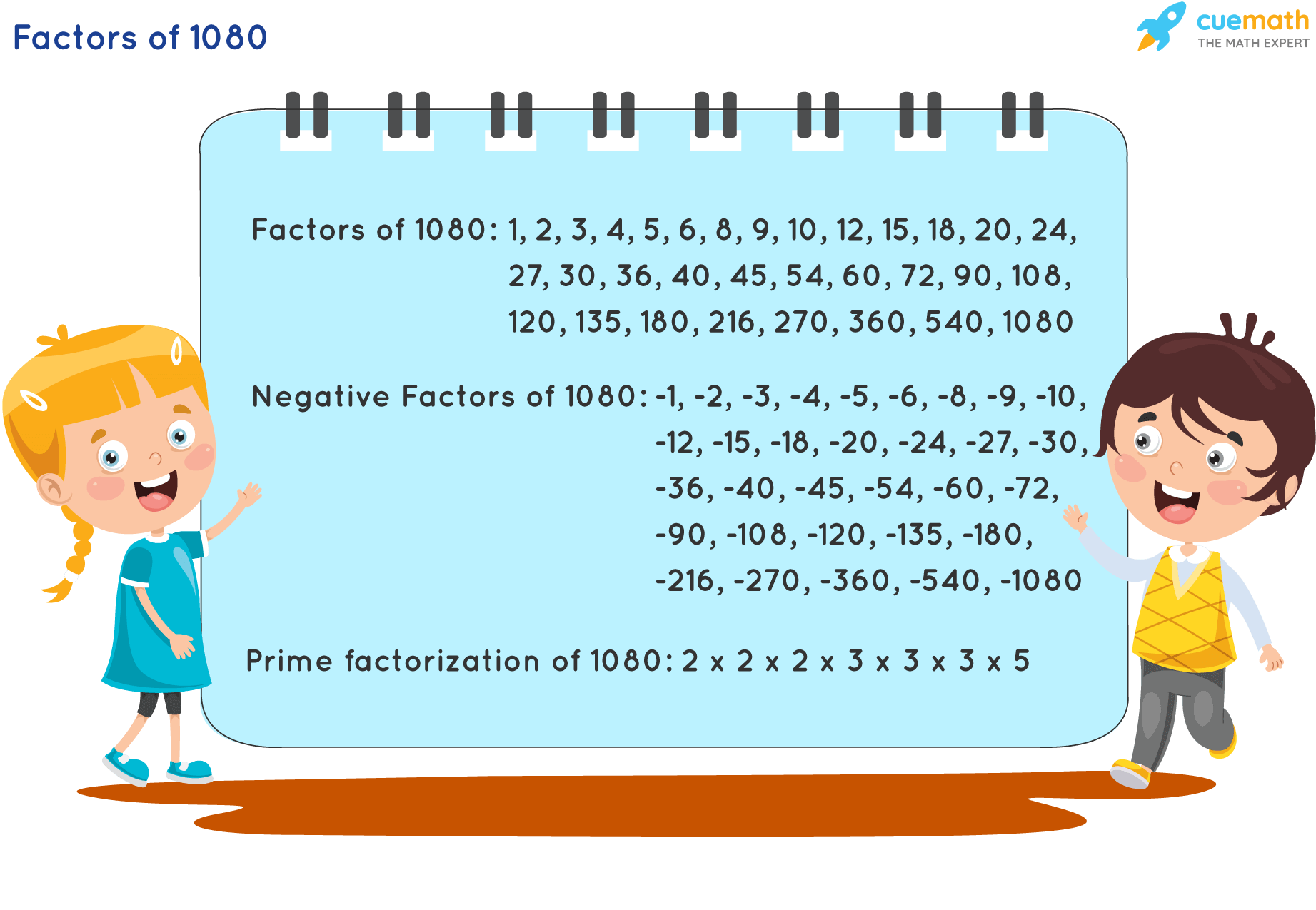
• All Factors of 1080: 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 27, 30, 36, 40, 45, 54, 60, 72, 90, 108, 120, 135, 180, 216, 270, 360, 540 and 1080
• Prime Factors of 1080: 2, 3, 5
• Prime Factorization of 1080: 2³ × 3³ × 5¹
• Sum of Factors of 1080: 3600

1.	What Are the Factors of 1080?
2.	Factors of 1080 by Prime Factorization
3.	Factors of 1080 in Pairs
4.	FAQs on Factors of 1080

Factors of 1080 are pairs of those numbers whose products result in 1080. These factors are either prime numbers or composite numbers.

How to Find the Factors of 1080?

To find the factors of 1080, we will have to find the list of numbers that would divide 1080 without leaving any remainder.

• 1080/9 = 120; therefore, 9 is a factor of 1080 and 120 is also a factor of 1080.
• 1080/360 = 3; therefore, 360 is a factor of 1080 and 3 is also a factor of 1080.

Similarly we can find other factors. Hence, the factors of 1080 are 1, 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 27, 30, 36, 40, 45, 54, 60, 72, 90, 108, 120, 135, 180, 216, 270, 360, 540, 1080.

☛ Also Check:

• Factors of 30 - The factors of 30 are 1, 2, 3, 5, 6, 10, 15, 30
• Factors of 40 - The factors of 40 are 1, 2, 4, 5, 8, 10, 20, 40
• Factors of 70 - The factors of 70 are 1, 2, 5, 7, 10, 14, 35, 70
• Factors of 13 - The factors of 13 are 1, 13
• Factors of 31 - The factors of 31 are 1, 31

The number 1080 is composite and therefore it will have prime factors. Now let us learn how to calculate the prime factors of 1080. The first step is to divide the number 1080 with the smallest prime factor, here it is 2. We keep dividing until it gives a non-zero remainder.

• 1080 ÷ 2 = 540
• 540 ÷ 2 = 270
• 270 ÷ 2 = 135

Further dividing 135 by 2 gives a non-zero remainder. So we stop the process and continue dividing the number 135 by the next smallest prime factor. We stop ultimately if the next prime factor doesn't exist or when we can't divide any further.

So, the prime factorization of 1080 can be written as 2³ × 3³ × 5¹ where 2, 3, 5 are prime.

Pair factors of 1080 are the pairs of numbers that when multiplied give the product 1080. The factors of 1080 in pairs are:

• 1 × 1080 = (1, 1080)
• 2 × 540 = (2, 540)
• 3 × 360 = (3, 360)
• 4 × 270 = (4, 270)
• 5 × 216 = (5, 216)
• 6 × 180 = (6, 180)
• 8 × 135 = (8, 135)
• 9 × 120 = (9, 120)
• 10 × 108 = (10, 108)
• 12 × 90 = (12, 90)
• 15 × 72 = (15, 72)
• 18 × 60 = (18, 60)
• 20 × 54 = (20, 54)
• 24 × 45 = (24, 45)
• 27 × 40 = (27, 40)
• 30 × 36 = (30, 36)

Negative pair factors of 1080 are:

• -1 × -1080 = (-1, -1080)
• -2 × -540 = (-2, -540)
• -3 × -360 = (-3, -360)
• -4 × -270 = (-4, -270)
• -5 × -216 = (-5, -216)
• -6 × -180 = (-6, -180)
• -8 × -135 = (-8, -135)
• -9 × -120 = (-9, -120)
• -10 × -108 = (-10, -108)
• -12 × -90 = (-12, -90)
• -15 × -72 = (-15, -72)
• -18 × -60 = (-18, -60)
• -20 × -54 = (-20, -54)
• -24 × -45 = (-24, -45)
• -27 × -40 = (-27, -40)
• -30 × -36 = (-30, -36)

NOTE: If (a, b) is a pair factor of a number then (b, a) is also a pair factor of that number.