Common Factors
Common factors of two or more numbers are those that can divide exactly without leaving any remainder. Factors are numbers that can divide another number exactly leaving zero as the remainder. When we compare the factors of two or more numbers we observe that some factors are the same or common. Such factors are known as common factors. Let us learn how to find common factors and solve a few examples to understand the concept better.
| 1. | What are Common Factors? |
| 2. | How to Find Common Factors? |
| 3. | Greatest Common Factor |
| 4. | FAQs on Common Factors |
What are Common Factors?
A factor of a number is an exact divisor of the given number or when a number is exactly divided by a divisor that divisor is known as a factor of that number. Factors of a number cannot be greater than the given number, they are either less than or equal to the given number. 1 is a common factor of all numbers and every number is a factor of itself. When we find the factors of two or more numbers we notice that some factors are common, this is because a number can be exactly divided by many divisors if the number is a multiple of that divisor. For example, the factors of 35 are {1,5,7,35} and factors of 45 are {1,3,5,9,15,45}. 1 and 5 are the numbers that are exactly dividing both the numbers. Thus the common factors of 35 and 45 are 1 and 5.
In math, the definition of the common factors states that "when two or more numbers are exactly divided by the same number(s), then those common divisors are known as common factors of the given numbers. Or we can say a common factor is a number that divides a pair of two or more numbers exactly without leaving any remainder.
How to Find Common Factors?
As we already discussed above, the factors are the numbers that are the exact divisors of a number. Let's understand the steps to find the common factors.
- Step 1: Write down all the factors of given numbers in separate rows.
- Step 2: Now check out the factors that are common in the given numbers and then write down all the common factors in a separate row.
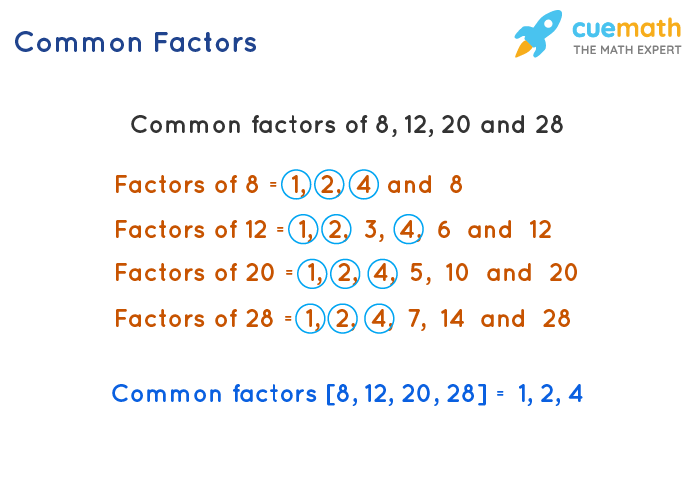
For example, let's find out the common factors of the given numbers- 8, 12, 20, and 28.
- Step 1: Writing down all the factors of given numbers.
Factors of 8 = 1, 2, 4, and 8.
Factors of 12 = 1, 2, 3, 4, 6, and 12.
Factors of 20 = 1, 2, 4, 5, 10, and 20.
Factors of 28 = 1, 2, 4, 7, 14, and 28.
- Step 2: Common factors (8, 12, 20, 28) = 1, 2 and 4.
Greatest Common Factor
The GCF-Greatest Common Factor is the largest common factor of two or more numbers. Once all the factors of the two or more numbers are taken out or written down we will find out the few factors that are common in given numbers. The largest number that is found in the common factors is the greatest common factor. Suppose p and q are the natural numbers. The GCF of two natural numbers p and q is the largest possible number that divides p and q. The greatest common factor is also known as the HCF-Highest Common Factor or greatest common divisor.
Let's understand GCF with help of an example. Taking two numbers 68 and 88 for finding out the greatest common factor between them. First list the factors of 68 and 88 and then we find out the common factors.
Factors of 68: 1, 2, 4, 17, 34 and 68.
Factors of 88: 1, 2, 4, 8, 11, 22, 44, and 88.
Thus, the common factors of 68 and 88 are 1, 2, and 4. Among these factors, 4 is the greatest factor. Thus, the GCF of 68 and 88 is 4. It is written as GCF(68, 88) = 4.
Related Articles
Check these interesting articles to know more about common factors.
FAQs on Common Factors
How do you Find the Common Factors?
The below-given steps are the way to find the common factors of two or more numbers:
- Step 1: Write down the factors of all given numbers.
- Step 2: Now check all the common factors of the given numbers and write down the common factors.
For example, common factors of 3 and 4 are,
Step 1: Factors of 3 = 1 and 3 and Factors of 4 = 1, 2, and 4.
Step 2: Checking all the common factors of 3 and 4. We can see, 1 is the only common factor of 3 and 4.
What are Common Factors in Math?
A factor is a number that divides the given number and leaves zero as the remainder. A single number can be a factor of many numbers like 4 is a factor of number 8 as well as 16 and so on, as 4 can divide both numbers exactly. When the factors of one number are also the factors of another number then those are called common factors of those two numbers. For example, common factors of 6 (factors: 1, 2, 3, 6) and 3 (factors: 1 and 3) are 1 and 3.
What are the Common Factors of 10 and 5?
The factors of 10 are 1, 2, 5, and 10. The factors of 5 are 1 and 5. So, the common factors of 10 and 5 are 1 and 5.
What are the Common Factors of 20 and 35?
The factors of 20 are 1, 2, 4, 5, 10, and 20. The factors of 35 are 1, 5, 7, and 35. So, the common factors of 20 and 35 are 1 and 5.
What are the Common Factors of 22 and 11?
The factors of 22 are 1, 2, 11, and 22. The factors of 11 are 1 and 11. So, the common factors of 22 and 11 are 1 and 11.
What is the Greatest Common Factor?
The greatest common factor is the largest factor which is common to two or more numbers. For example, the factors of 4 are 1, 2, and 4, and factors of 16 are 1, 2, 4, 8, and 16. We can see that 1, 2, and 4 are the common factors and in these 4 is the largest common factor as compared to 1 and 2. Therefore, 4 is the greatest common factor of 4 and 16.
What is the Lowest Common Factor?
The lowest common factor will always be 1 for any set of numbers, as 1 is the factor of all numbers. For example, the factors of 8 are 1, 2, 4, and 8, and factors of 16 are 1, 2, 4, 8, and 16. We can see that 1, 2, 4, and 8 are the common factors and 1 is the smallest common factor.
What is the Greatest Common Factor of 12 and 14?
The factors of 12 are 1, 2, 3, 4, 6, and 12. The factors of 14 are 1, 2, 7, and 14. So, the greatest common factor of 12 and 14 is 2.