Two water taps together can fill a tank in 9 3/8 hours. The tap of larger diameter takes 10 hours less than the smaller one to fill the tank separately. Find the time in which each tap can separately fill the tank.
Solution:
Let the tap of smaller diameter fill the tank in x hours.
The tap of larger diameter takes (x - 10) hours.
Given, the two water taps can fill the tank together in 9 3/8 hours = 75/8 hours
The smaller tap fills the tank in x hours.
Therefore, in one hour, the part of the tank filled by the smaller tap = 1/x
Also, the larger tap fills the tank in (x - 10) hours.
Thus, in one hour, the part of the tank filled by the larger tap = 1/(x - 10)
Now, considering both the pipes working together we have,
In 1 hour, the part of the tank filled by the smaller and larger tap together is,
1/x + 1/(x - 10) = 8/75 [ Since, the two water taps can fill the tank together in 75/8 hours]
By taking LCM and solving,
(x - 10 + x) / x(x -10) = 8/75
(2x - 10) / (x2 - 10x) = 8/75
75(2x - 10) = 8(x2 - 10x)
150x - 750 = 8x2 - 80x
8x2 - 80x - 150x + 750 = 0
8x2 - 230x + 750 = 0
4x2 - 115x + 375 = 0
We will solve this quadratic equation using the quadratic formula.
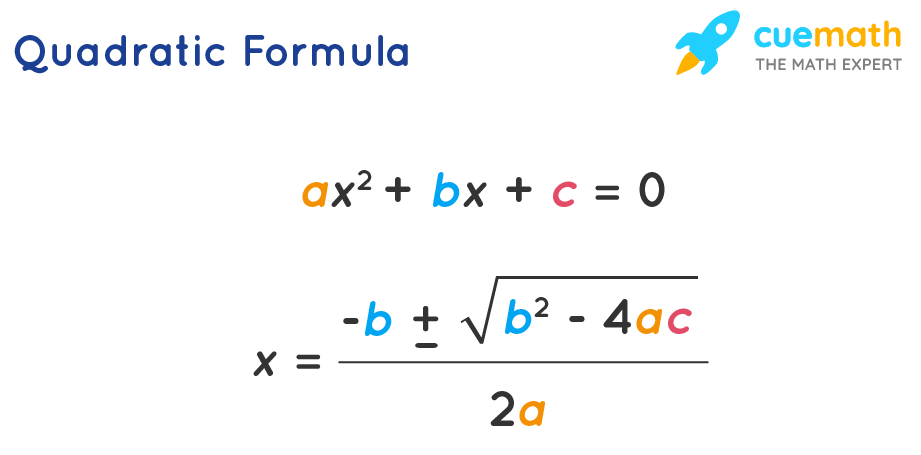
Comparing 4x2 - 115x + 375 = 0 with ax2 + bx + c = 0, we get a = 4, b = - 115, c = 375
b² - 4ac = (- 115)2 - 4(4)(375)
= 13225 - 6000
= 7225
b² - 4ac > 0
Hence, real roots exist.
x = [- b ± √(b2 - 4ac)] / 2a
x = (115 ± √7225) / 8
x = (115 + 85) / 8 and x = (115 - 85) / 8
x = 200/8 and x = 30/8
x = 25 and x = 15/4
x cannot be 15/4 hours because the larger tap takes 10 hours less than x and this would give us a negative value as 15/4 <10.
Thus, x = 25
Time taken by smaller tap x = 25 hours
Time taken by larger tap (x - 10) = 15 hours
☛ Check: NCERT Solutions for Class 10 Maths Chapter 4
Video Solution:
Two water taps together can fill a tank in 9 3/8 hours. The tap of larger diameter takes 10 hours less than the smaller one to fill the tank separately. Find the time in which each tap can separately fill the tank
Class 10 Maths NCERT Solutions Chapter 4 Exercise 4.3 Question 9
Summary:
If two water taps together can fill a tank in 9 3/8 hours and the tap of larger diameter takes 10 hours less than the smaller one to fill the tank separately, then the time taken by the smaller tap is 25 hours and the time taken by the larger tap is 15 hours to separately fill the tank.
☛ Related Questions:
- Find the roots of the quadratic equations given in Q.1 above by applying the quadratic formula.
- Find the roots of the following equations:(i) x - 1/x = 3, x ≠ 0(ii) 1/(x + 4) - 1/(x - 7) = 11/30, x ≠ - 4, 7
- The sum of the reciprocals of Rehman’s age (in years) 3 years ago and 5 years from now is 1/3. Find his present age.
- In a class test, the sum of Shefali’s marks in Mathematics and English is 30. Had She got 2 marks more in Mathematics and 3 marks less in English, the product of their marks would have been 210. Find her marks in the two subjects.
visual curriculum