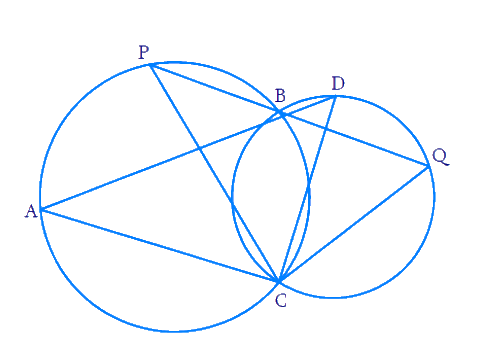
Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D, P and Q respectively (see Fig. 10.40). Prove that ∠ACP = ∠QCD.

Solution:

∠ACP and ∠ABP lie in the same segment. Similarly, ∠DCQ and ∠DBQ lie in the same segment. 

We know that angles in the same segment of a circle are equal.

So, we get ∠ACP = ∠ABP and ∠QCD = ∠QBD

Also, ∠QBD = ∠ABP (Vertically opposite angles)

Therefore, ∠ACP = ∠QCD.

☛ Check: NCERT Solutions for Class 9 Maths Chapter 10

Video Solution:

Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D, P and Q respectively (see Fig. 10.40). Prove that ∠ACP = ∠QCD

Maths NCERT Solutions Class 9 Chapter 10 Exercise 10.5 Question 9

Summary:

If two circles intersect at two points B and C, through B, two line segments ABD and PBQ, are drawn to intersect the circles at A, D, P, and Q respectively, then ∠ACP = ∠QCD.

☛ Related Questions:

• If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
• ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD
• Prove that a cyclic parallelogram is a rectangle.
• Prove that the line of centers of two intersecting circles subtends equal angles at the two points of intersection.