NCERT Solutions Class 12 Maths Chapter 10 Exercise 10.2 Vector Algebra
NCERT solutions for class 12 maths chapter 10 exercise 10.2 vector algebra covers several subparts of this lesson such as the addition of vectors, their properties, multiplication by a scalar, components of a vector, section formula, and how to find the position as well as the magnitude of a vector joining two points. To add any two vectors we either use the triangle or parallelogram law of vector addition. The vector sum of the three sides of a triangle taken in order is 0 and the vector sum of two coinitial vectors is the diagonal of the parallelogram whose adjacent sides are the given vectors. Further, if we multiply a scalar λ with a vector, only the magnitude gets changed and not the direction. The final crucial concept that kids learn in the NCERT Solutions Class 12 maths chapter 10 exercise 10.2 is how to divide a line in a given ratio externally or internally.
There are a total of 19 questions that range from a medium to tough level covering all the aforementioned topics in depth. As there are many formulas associated with these sums it is advised to keep a formula sheet close when attempting the Class 12 maths NCERT solutions chapter 10 exercise 10.2 vector algebra. Given below is the PDF version of these solutions that kids can use as a supplement for the exercise.
☛ Download NCERT Solutions Class 12 Maths Chapter 10 Exercise 10.2
Exercise 10.2 Class 12 Chapter 10




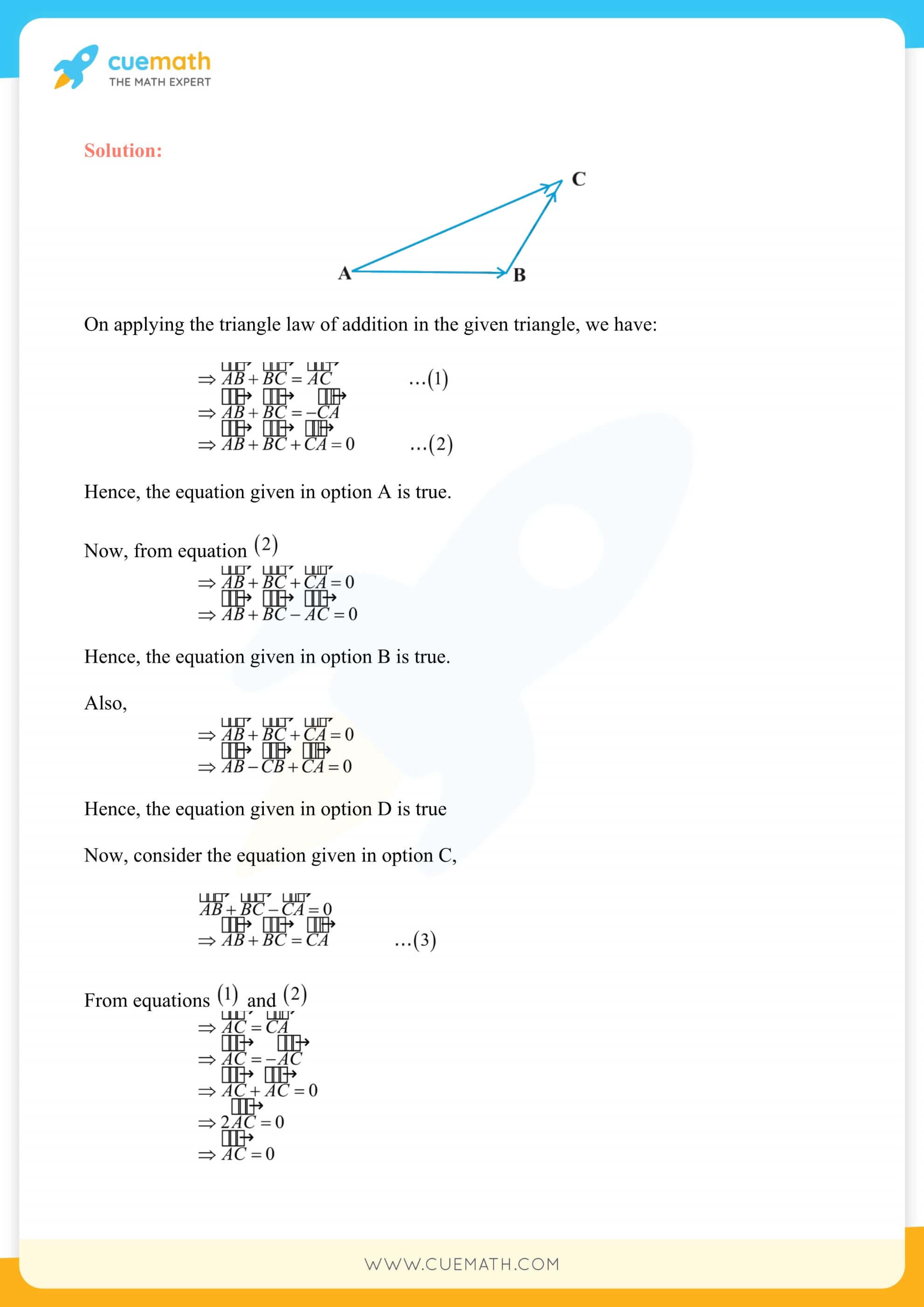

More Exercises in Class 12 Maths Chapter 10
- NCERT Solutions Class 12 Maths Chapter 10 Ex 10.1
- NCERT Solutions Class 12 Maths Chapter 10 Ex 10.3
- NCERT Solutions Class 12 Maths Chapter 10 Ex 10.4
- NCERT Solutions Class 12 Maths Chapter 10 Miscellaneous Ex
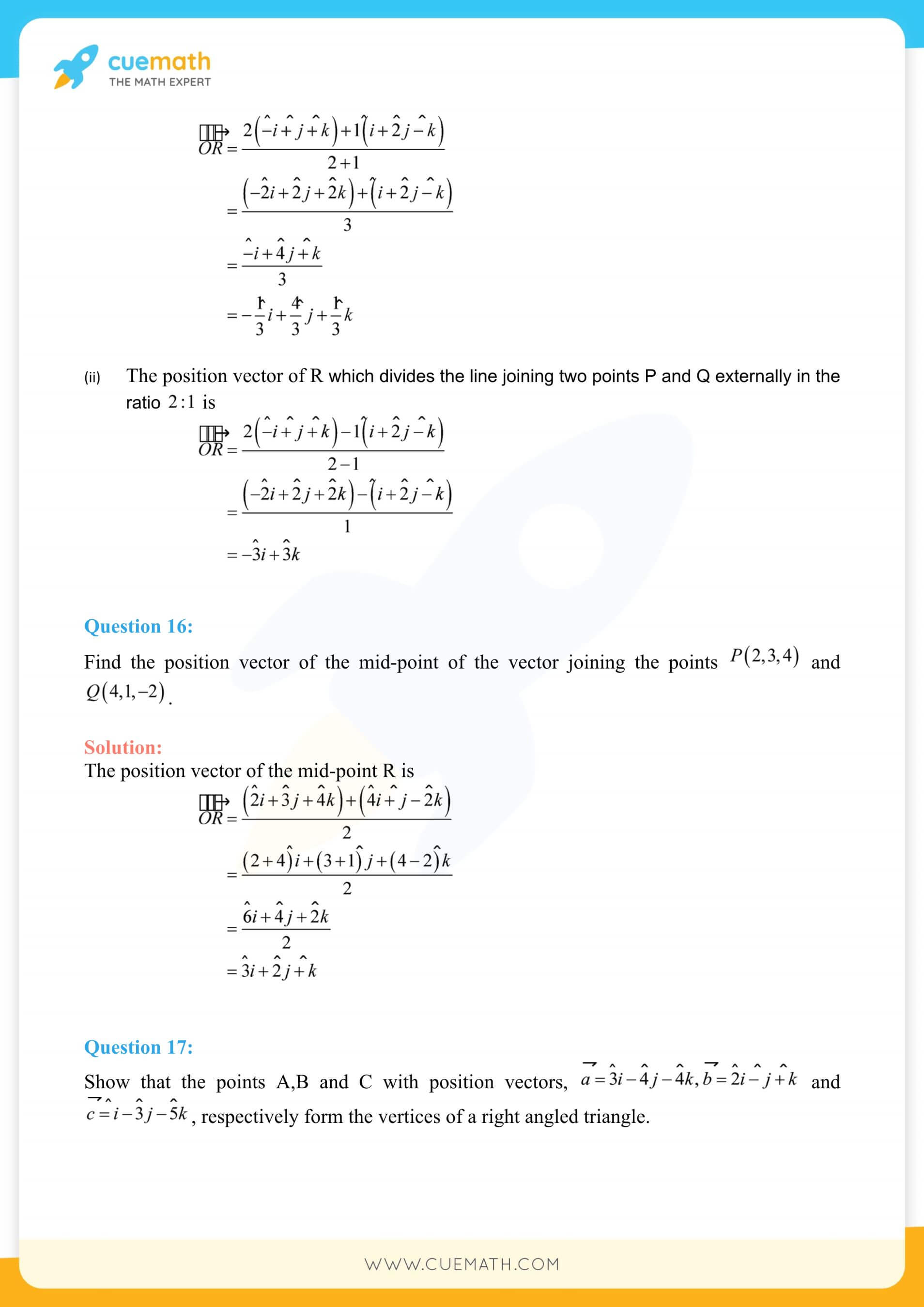
NCERT Solutions Class 12 Maths Chapter 10 Exercise 10.2 Formulas
NCERT solutions class 12 maths chapter 10 vector algebra has certain properties that are followed by the addition and scalar multiplication of vectors. They are given below as:
If we have three vectors a, b and c then
- Commutative Property: a + b = b + a. This means that the order of adding the vectors does not matter, the resultant will be the same
- Associative Property: (a + b) + c = a + (b + c). This implies that the sum of three vectors does not depend on which pair of vectors is added first.
Distributive Properties for Multiplication if k and m are scalars while a is a vector:
- ka + ma = (k + m) a
- (km)a = k(ma)
- k (a + b) = ka + kb
Download Cuemath NCERT Solutions PDF for free and start learning!
visual curriculum
