In the following figure, FD||BC||AE and AC||ED. Find the value of x.
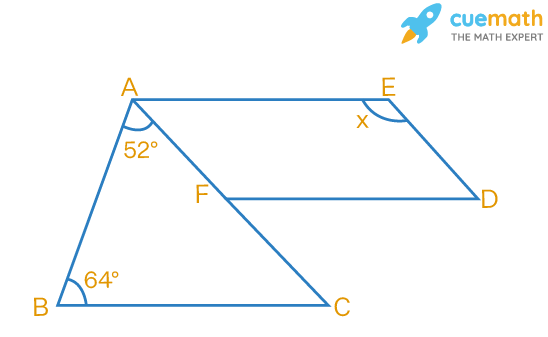
Solution:
Given, FD||BC||AE and AC||ED.
We have to find the value of x.
Extend DF such that it intersects AB at G.

Consider triangle ABC,
By angle sum property of a triangle,
∠A + ∠B + ∠C = 180°
52° + 64° + ∠C = 180°
116° + ∠C = 180°
∠C = 180° - 116°
∠C = 64°
We observe that DG || BC and DG || AE.
Since FG || BC with FC as transversal, the corresponding angles are equal.
So, ∠ACB = ∠AFG
∠AFG = 64°
We know that the linear pair of angles is supplementary.
∠GFA + ∠AFD = 180°
64° + ∠AFD = 180°
∠AFD = 180° - 64°
∠AFD = 116°
We observe that FD || AE and AF || ED
Therefore, AEDF is a parallelogram.
We know that the opposite angles of a parallelogram are equal.
So, ∠AFD = ∠AED
∠AFD = x
Therefore, x = 116°
✦ Try This: In a parallelogram ABCD. Its diagonals AC and BD intersect each other at point O. If AC = 12 cm and BD = 9 cm; find lengths of OA and OB.
☛ Also Check: NCERT Solutions for Class 8 Maths
NCERT Exemplar Class 8 Maths Chapter 5 Problem 184
In the following figure, FD||BC||AE and AC||ED. Find the value of x.
Summary:
In the given figure, FD||BC||AE and AC||ED. The value of x is 116 degrees.
☛ Related Questions:
visual curriculum
