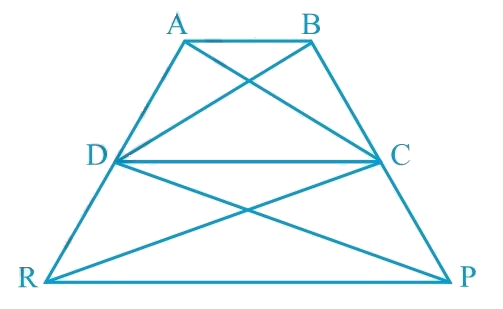
In Fig.9.29, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums
Solution:
We have to prove quadrilaterals ABCD and DCPR are trapeziums.
It is given that Area (ΔDRC) = Area (ΔDPC)
As ΔDRC and ΔDPC are lying on the same base DC having equal areas, therefore, they must lie between the same parallel lines.
According to Theorem 9.3: Two triangles having the same base (or equal bases) and equal areas lie between the same parallels.
∴ DC || RP
Therefore, DCPR is a trapezium.
It is also given that Area (ΔBDP) = Area (ΔARC)
Now, subtract ar (ΔDPC) form ar (ΔBDP) and ar (ΔDRC) from ar (ΔARC)
ar (ΔBDP) - ar (ΔDPC) = ar (ΔARC) - ar (ΔDRC) [Since, ar (ΔDPC) = ar (ΔDRC)]
ar (ΔBDC) = ar (ΔADC)
Since ΔBDC and ΔADC are on the same base CD having equal areas, they must lie between the same parallel lines. (According to Theorem 9.3)
∴ AB || CD
Therefore, ABCD is a trapezium.
☛ Check: NCERT Solutions for Class 9 Maths Chapter 9
Video Solution:
In Fig.9.29, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums
Maths NCERT Solutions Class 9 - Chapter 9 Exercise 9.3 Question 16:
Summary:
In the given figure, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC), then we have proved that ABCD and DCPR are trapeziums.
☛ Related Questions:
- In Fig.9.23, E is any point on median AD of a ∆ ABC. Show that ar (ABE) = ar (ACE).
- In a triangle ABC, E is the mid-point of median AD. Show that ar (BED) = 1/4 ar(ABC).
- Show that the diagonals of a parallelogram divide it into four triangles of equal area.
- In Fig. 9.24, ABC and ABD are two triangles on the same base AB. If line- segment CD is bisected by AB at O, show that ar(ABC) = ar (ABD).
visual curriculum
