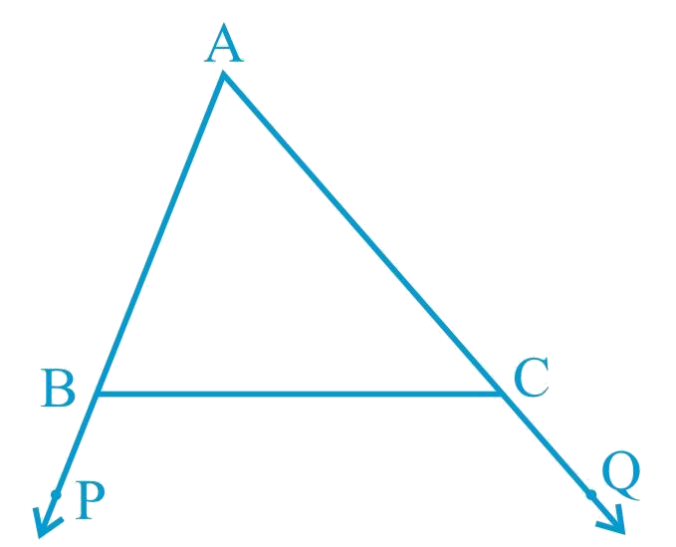
In Fig. 7.48, sides AB and AC of Δ ABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.

Solution:

Let's look into the solution below.

In the given figure,

∠ABC + ∠PBC = 180° [Linear pair of angles]

Also, ∠ABC = 180° - ∠PBC   ... (1)

∠ACB + ∠QCB = 180° [Linear pair]

∠ACB = 180° - ∠QCB  ... (2)

As ∠PBC < ∠QCB (given),

180^o - ∠PBC > 180^o - ∠QCB

∠ABC > ∠ACB [From Equations (1) and (2)]

Thus, AC > AB (Side opposite to the larger angle is larger).

Hence proved, AC > AB.

☛ Check: NCERT Solutions for Class 9 Maths Chapter 7

Video Solution:

In Fig. 7.48, sides AB and AC of Δ ABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.

NCERT Maths Solutions Class 9 Chapter 7 Exercise 7.4 Question 2:

Summary:

If sides AB and AC of ΔABC are extended to points P and Q respectively, ∠PBC < ∠QCB, we have proved that AC > AB

☛ Related Questions:

• In the given figure, ∠B < ∠A and ∠C < ∠D. Show that AD < BC.
• AB and CD are respectively the smallest and longest sides of a quadrilateral ABCD (see Fig. 7.50). Show that ∠A > ∠C and ∠B > ∠D.
• In Fig 7.51, PR > PQ and PS bisects ∠QPR. Prove that ∠PSR > ∠PSQ.
• Show that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.