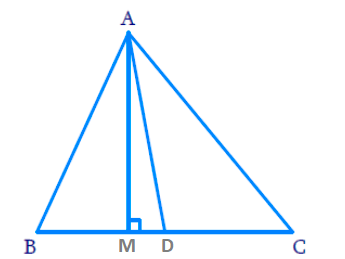
In Fig. 6.60, AD is a median of a triangle ABC and AM ⊥ BC. Prove that :
(i) AC² = AD² + BC.DM + (BC/2)²
(ii) AB² = AD² - BC.DM + (BC/2)²
(iii) AC² + AB² = 2AD² + 1/2 BC²
Solution:
According to Pythagoras theorem, in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
(i) In ΔAMC
∠AMC = 90°
AC² = AM² + CM²
AC² = AM² + (DM + CD)²
AC² = AM² + DM² + CD² + 2DM.CD [Since (a + b)² = a² + b² + 2ab]
AC² = AD² + (BC/2)² + 2DM (BC/2) [Since, in ΔAMD, AD² = AM² + DM² and D is the midpoint of BC means BD = CD = BC/2]
AC² = AD² + BC.DM + (BC/2)² ......(i)
(ii) In ΔAMB
∠AMB = 90°
AB² = AM² + BM²
AB² = AM² + (BD - DM)²
AB² = AM² + BD² + DM² - 2BD.DM [Since (a - b)² = a² + b² - 2ab]
AB² = AM² + DM² + (BC/2)² - 2 (BC/2)DM [Since, D is the midpoint of BC means BD = CD = BC/2]
AB² = AD² - BC.DM + (BC/2)² .....(ii) [Since, in ΔAMD, AD² = AM² + DM² and D is the midpoint of BC means BD = CD = BC/2]
(iii) Adding (i) and (ii)
AC² + AB² = AD² + (BC/2)² + BC.DM + AD² + (BC/2)² - BC.DM
AC² + AB² = 2AD² + 2(BC/2)²
AC² + AB² = 2AD² + 1/2 BC²
☛ Check: NCERT Solutions for Class 10 Maths Chapter 6
Video Solution:
In Fig. 6.60, AD is a median of a triangle ABC and AM ⊥ BC. Prove that : (i) AC² = AD² + BC.DM + (BC/2)² (ii) AB² = AD² - BC.DM + (BC/2)² (iii) AC² + AB² = 2AD² + 1/2BC²
NCERT Class 10 Maths Solutions Chapter 6 Exercise 6.6 Question 5
Summary:
In the above figure, AD is a median of a triangle ABC and AM ⊥ BC. Hence proved that AC² = AD² + BC.DM + (BC/2)² , AB² = AD² - BC.DM + (BC/2)² and AC² + AB² = 2AD² + 1/2BC²
☛ Related Questions:
- Prove that the sum of the squares of the diagonals of parallelogram is equal to the sum of the squares of its sides.
- In Fig. 6.61, two chords AB and CD intersect each other at the point P. Prove that: (i) ΔAPC ~ ΔDPB (ii) AP.PB = CP.DP.
- In Fig. 6.62, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that (i) ΔPAC ~ ΔPDB (ii) PA.PB = PC.PD.
- In Fig. 6.63, D is a point on side BC of ∆ABC such that BD/CD = BA/CA. Prove that: AD is the bisector of ∠BAC.
visual curriculum
