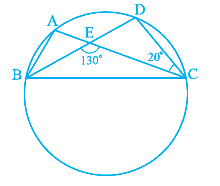
In Fig. 10.39, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC

Solution:

We will use the following concepts to answer the question.

• The sum of angles in a triangle is 180°.

• Angles in the same segment are equal.

Consider the straight-line BD. As the line AC intersects with the line BD, the sum of two adjacent angles so formed is 180°.

Therefore, ∠BEC + ∠DEC = 180°

130° + ∠DEC = 180°

∠DEC =180° - 130° = 50°

Consider the ∆DEC, the sum of all angles will be 180º.

∠DEC + ∠EDC + ∠ECD = 180°

50° + ∠EDC + 20° = 180°

∠EDC = 180° - 70° = 110°

∴ ∠BDC = ∠EDC = 110°

We know that angles in the same segment of a circle are equal.

∴ ∠BAC = ∠BDC = 110°

☛ Check: NCERT Solutions for Class 9 Maths Chapter 10

Video Solution:

In Fig. 10.39, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC

Maths NCERT Solutions Class 9 Chapter 10 Exercise 10.5 Question 5

Summary:

If in the given figure A, B, C, and D are four points on a circle, AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°, then ∠BAC=110°.

☛ Related Questions:

• ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70°, ∠BAC is 30° find ∠BCD. Further if AB = BC, find ∠ECD.
• If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
• If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
• Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D, P and Q respectively (see Fig. 10.40). Prove that ∠ACP = ∠QCD.