Refer to Example 13. (i) Complete the following table:

(ii) A student argues that ‘there are 11 possible outcomes 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 and 12. Therefore, each of them has a probability 1/11. Do you agree with this argument? Justify your answer

Solution:

We use the basic concepts of probability to solve the problem.

(i) Number of possible outcomes to get the sum as 2 = (1,1) = 2

Number of possible outcomes to get the sum as 3 = (2,1), (1, 2) = 2

Number of possible outcomes to get the sum as 4 = (2, 2), (1, 3), (3,1) = 3

Number of possible outcomes to get the sum as 5 = (3, 2), (2, 3), (4,1), (1, 4) = 4

Number of possible outcomes to get the sum as 6 = (5,1), (1, 5), (3, 3), (4, 2), (2, 4) = 5

Number of possible outcomes to get the sum as 7 = (4, 3), (3, 4), (6,1), (1, 6), (5, 2), (2, 5) = 6

Number of possible outcomes to get the sum as 8 = (4, 4), (6, 2), (2, 6), (5, 3), (3, 5) = 5

Number of possible outcomes to get the sum as 9 = (5, 4), (4, 5), (6, 3), (3, 6) = 4

Number of possible outcomes to get the sum as 10 = (5, 5), (6, 4), (4, 6) = 3

Number of possible outcomes to get the sum as 11 = (6, 5), (5, 6) = 11

Number of possible outcomes to get the sum as 12 = (6, 6) = 1

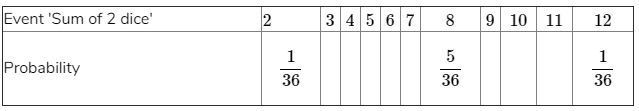
Thus, the table is:

(ii) Probability of each of them is not 1/11 as these are not equally likely.

Check out more about terms of probability.

☛ Check: NCERT Solutions for Class 10 Maths Chapter 15

Video Solution:

Refer to Example 13. (i) Complete the following table:
(ii) A student argues that ‘there are 11 possible outcomes 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 and 12. Therefore, each of them has a probability 1/11. Do you agree with this argument? Justify your answer.

NCERT Solutions for Class 10 Maths Chapter 15 Exercise 15.1 Question 22

Summary:

A student argues that ‘there are 11 number of outcomes 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 and 12. (i) P(3) = 2/36, P(4) = 3/36, P(5) = 4/36, P(6) = 5/36, P(7) = 6/36, P(9) = 4/36, P(10) = 3/36, and P(11) = 2/36; (ii) Probability of each of them is not 1/11 as these are not equally likely.

☛ Related Questions:

• A game consists of tossing a one-rupee coin 3 times and noting its outcome each time. Hanif wins if all the tosses give the same result i.e., three heads or three tails, and loses otherwise. Calculate the probability that Hanif will lose the game.
• A die is thrown twice. What is the probability that(i) 5 will not come up either time?(ii) 5 will come up at least once?[Hint : Throwing a die twice and throwing two dice simultaneously are treated as the same experiment]
• Which of the following arguments are correct and which are not correct? Give reasons for your answer. (i) If two coins are tossed simultaneously there are three possible outcomes—two heads, two tails or one of each. Therefore, for each of these outcomes, the probability is 1/3⋅ (ii) If a die is thrown, there are two possible outcomes—an odd number or an even number. Therefore, the probability of getting an odd number is 1/2.