Find the values of the angles x, y and z in each of the following:

Solution:
We use the geometry concepts like supplementary angles, vertically opposite angles and adjacent angles to solve the problem.
There are two operations done in sequence. First, if one angle is 55° then the angle opposite to it will also be 55° as vertically opposite angles are equal.
Also sum of ∠x + ∠y = 180° and ∠z + 55° = 180°.
Solve for ∠x, ∠y, ∠z:
(i) ∠x = 55° (Vertically opposite angle)
∠x + ∠y = 180°(Linear pair)
55 ° + ∠y = 180°
∠y = 180° - 55 °
∠y = 125 °
Therefore, ∠y = ∠𝑧 = 125° (Vertically opposite angle)
Hence, ∠x = 55° , ∠y= 125° and ∠z = 125°
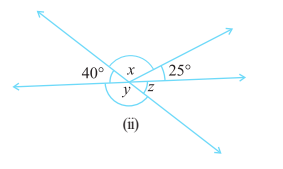
(ii) 40° + 𝑥 + 25° = 180°(Angles on straight line)
x + 65° = 180°
x = 180° - 65° = 115°
Also, 40° + 𝑦 = 180°(Linear pair)
𝑦 = 180° − 40°
𝑦 = 140°
𝑦 + 𝑧 = 180°(Linear pair)
140° + 𝑧 = 180° (Since, 𝑦 = 140°)
𝑧 = 180°- 140°
𝑧 = 40°
Thus, 𝑥 = 115°, 𝑦 = 140° and 𝑧 = 40°
☛ Check: NCERT Solutions for Class 7 Maths Chapter 5
Video Solution:
Find the values of the angles x, y and z in each of the following:
NCERT Solutions for Class 7 Maths Chapter 5 Exercise 5.1 Question 12
Summary:
The values of the angles x, y and z in each of the following is: (i) ∠x = 55° , ∠y= 125° and ∠z = 125° and (ii) 𝑥 = 115°, 𝑦 = 140° and 𝑧 = 40°
☛ Related Questions:
- In The Given Figure 1 And 2 Are Supplementary Angles If 1 Is Decreased What Changes Should Take Place In 2 So That Both The Angles Remain Supplementary
- Can Two Angles Be Supplementary If Both Of Them Are I Acute Ii Obtuse Iii Right
- An Angle Is Greater Than 45 Is Its Complementary Angle Greater Than 45 Or Equal To 45 Or Less Than 45
- In The Adjoining Figure Iis 1 Adjacent To 2 Iiis Aoc Adjacent To Aoe Iiido Coe And Eod Form A Linear Pair Ivare Bod And Doa Supplementary
visual curriculum
