

Calculate the area of the designed region in Fig. 12.34 common between the two quadrants of circles of radius 8 cm each
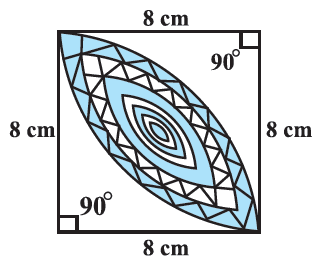
Solution:
We use the formula for the area of sectors of the circle and the area of the square to solve the problem.
In a circle with radius r and the angle at the centre with degree measure θ,
Area of a sector = θ/360° × πr2
Area of a quadrant = 90°/360° × πr2 = 1/4 πr2
Area of plain or unshaded part of the square = Area of square - Area of quadrant
= side2 - (1/4 × πr2)
= (8 cm × 8 cm) - (1/4 × 22/7 × 8 cm × 8 cm)
= 64 cm2 - 352/7 cm2
= 96/7 cm2
Area of the designed region = Area of the quadrant - Area of plain or unshaded part of the square
= (1/4 × 22/7 × 8 cm × 8 cm) - 96/7 cm2
= 352/7 cm2 - 96/7 cm2
= 256/7 cm2
☛ Check: NCERT Solutions for Class 10 Maths Chapter 12
Video Solution:
Calculate the area of the designed region in Fig. 12.34 common between the two quadrants of circles of radius 8 cm each
NCERT Solutions Class 10 Maths Chapter 12 Exercise 12.3 Question 16
Summary:
The area of the designed region common between the two quadrants of circles of radius 8 cm each is 256/7 cm2.
☛ Related Questions:
- In a circular table cover of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as shown in Fig. 12.24. Find the area of the design.
- In Fig. 12.25, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touch externally two of the remaining three circles. Find the area of the shaded region.
- Fig. 12.26 depicts a racing track whose left and right ends are semicircular. Fig. 12.26 The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find :(i) the distance around the track along its inner edge(ii) the area of the track.
- In Fig. 12.27, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.
visual curriculum