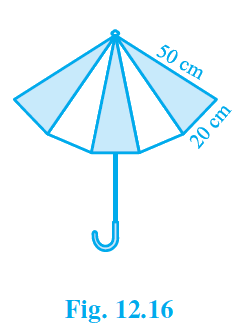
An umbrella is made by stitching 10 triangular pieces of cloth of two different colours (See Fig. 12.16), each piece measuring 20 cm, 50 cm, and 50 cm. How much cloth of each colour is required for the umbrella?
Solution:
Given: Dimensions of each triangular piece used in umbrella.
Reasoning: By using Heron’s formula, we can calculate the area of a triangle.
Heron's formula for the area of a triangle, Area = √s(s - a)(s - b)(s - c)
Where a, b, and c are the sides of the triangle, and
s = Semi-perimeter = Half the Perimeter of the triangle = (a + b + c)/2
We know that umbrella is made of 10 triangular pieces of cloth of two different colours.
Let us calculate the area of one triangle.
For each triangle, a = b = 50 cm, c = 20 cm
Semi Perimeter
s = (a + b + c)/2
= (50 + 50 + 20)/2
= 120/2
= 60 cm
By using Heron’s formula,
Area of triangle = √s(s - a)(s - b)(s - c)
= √s(s - a)(s - b)(s - c)
= √60(60 - 50)(60 - 50)(60 - 20)
= √60 × 10 × 10 × 40
= 200√6 cm2
Therefore,
Area of 10 triangular pieces = 10 × 200√6 cm2 = 2000√6 cm2
Hence, cloth required for each colour = (Total area of the cloth)/2
= (2000√6)/2
= 1000√6 cm2
Thus, 1000√6 cm2 cloth of each colour is required for the umbrella.
☛ Check: NCERT Solutions Class 9 Maths Chapter 12
Video Solution:
An umbrella is made by stitching 10 triangular pieces of cloth of two different colours (See Fig. 12.16), each piece measuring 20 cm, 50 cm and 50 cm. How much cloth of each colour is required for the umbrella?
Class 9 Maths NCERT Solutions Chapter 12 Exercise 12.2 Question 6
Summary:
It is given that an umbrella is made by stitching 10 triangular pieces of cloth of two different colours. If each piece measuring 20 cm, 50 cm and 50 cm, we see that the cloth required for each colour is 1000√6 cm2.
☛ Related Questions:
- A park, in the shape of a quadrilateral ABCD, has ∠C = 90°, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m. How much area does it occupy?
- Find the area of a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 4 cm, DA = 5 cm and AC = 5 cm.
- Radha made a picture of an aeroplane with coloured paper as shown in Fig 12.15. Find the total area of the paper used.
- A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 26 cm, 28 cm, and 30 cm, and the parallelogram stands on the base 28 cm, find the height of the parallelogram.
visual curriculum
