A day full of math games & activities. Find one near you.

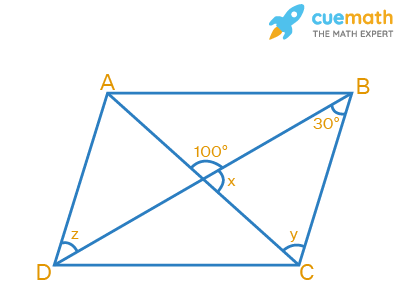
ABCD is a parallelogram. Find the value of x, y and z.

Solution:

Given, ABCD is a parallelogram.

We have to find the value of x, y and z.

Consider triangle OBC,

By exterior angle property of a triangle,

y + 30° = 100°

y = 100° - 30°

y = 70°

By angle sum property of a triangle,

x + y + 30° = 180°

x + 70° + 30° = 180°

x + 100° = 180°

x = 180° - 100°

x = 80°

Since AD || BC with BD as transversal, the alternate interior angles are equal.

So, ∠ADO = ∠OBD

z = 30°

Therefore, the values of x, y and z are 80°, 70° and 30°.

✦ Try This: ABCD is a parallelogram. ∠A = 80°. The internal bisectors of ∠B and ∠C meet at O. find the measure of the three angles of the triangle BCO.

☛ Also Check: NCERT Solutions for Class 8 Maths

NCERT Exemplar Class 8 Maths Chapter 5 Problem 172

ABCD is a parallelogram. Find the value of x, y and z.

Summary:

ABCD is a parallelogram. The values of x, y and z are 80°, 70° and 30°.

☛ Related Questions: