ABC is an equilateral triangle of side 2a. Find each of its altitudes
Solution:
We know that in an equilateral triangle, the perpendicular drawn from its vertex to the opposite side bisects the opposite side.
Let us analyze using the figure shown below.
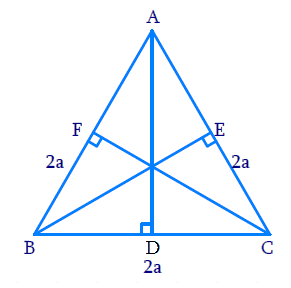
We know that, in a right triangle, the square of the hypotenuse is equal to the sum of the square of the other two sides.
In the equilateral ΔABC, we see that AB = BC = CA = 2a [From the figure shown above]
AD ⊥ BC [Construction]
⇒ BD = CD = 1/2 BC = a [Since the perpendicular drawn from a vertex to the opposite side bisects the opposite side in an equilateral triangle]
In ΔADB, using pythagoras theorem,
AB2 = AD2 + BD2
AD2 = AB2 - BD2
AD2 = (2a)2 - a2
AD2 = 4a2 - a2
AD2 = 3a2
AD = 3a
⇒ AD = √3a units
Similarly, we can prove that, BE = CF = √3a units
☛ Check: NCERT Solutions for Class 10 Maths Chapter 6
Video Solution:
ABC is an equilateral triangle of side 2a. Find each of its altitudes
NCERT Class 10 Maths Solutions Chapter 6 Exercise 6.5 Question 6
Summary:
If ABC is an equilateral triangle of side 2a, then each of its altitudes are AD = BE = CF = √3a units.
☛ Related Questions:
- Prove that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals.
- In Figure 6.54, O is a point in the interior of a triangle ABC, OD ⊥ BC, OE ⊥ AC and OF ⊥ AB. Show that(i) OA2 + OB2 + OC2 - OD2 - OE2 - OF2 = AF2 + BD2 + CE2(ii) AF2 + BD2 + CE2 = AE2 + CD2 + BF2
- A ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from base of the wall.
- A guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
visual curriculum