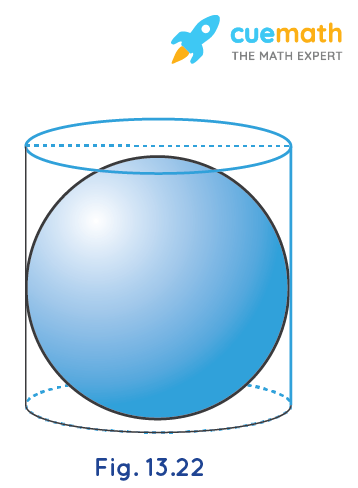
A right circular cylinder just encloses a sphere of radius r (see Fig. 13.22). Find
i) surface area of the sphere,
ii) curved surface area of the cylinder,
iii) ratio of the areas obtained in (i) and (ii).
Solution:
Since the cylinder encloses the sphere as we can see in the figure, the radius of the cylinder will be equal to the radius of the sphere and the height of the cylinder will be equal to the diameter of the sphere.
The radius of the sphere = Radius of the cylinder = r
Height of the cylinder, h = diameter of the sphere = 2r
Thus, h = 2r
The surface area of a sphere with radius r = 4πr2
Curved surface area of a cylinder = 2πrh
(i) Surface area of the sphere = 4πr2
(ii) Curved surface area of the cylinder = 2πrh
= 2πr × 2r [Since, h = 2r]
= 4πr2
(iii) The ratio of the areas obtained in (i) and (ii) is:
4πr2/4πr2 = 1/1
Thus, the surface area of the sphere and the curved surface area of the cylinder is 4πr2 and the ratio between these areas is 1:1.
☛ Check: NCERT Solutions for Class 9 Maths Chapter 13
Video Solution:
A right circular cylinder just encloses a sphere of radius r. Find i) surface area of the sphere, ii) curved surface area of the cylinder, iii) ratio of the areas obtained in (i) and (ii).
Class 9 Maths NCERT Solutions Chapter 13 Exercise 13.4 Question 9
Summary:
It is given that there is a right circular cylinder encloses a sphere of radius r. We have found that the surface area of the sphere is 4πr2, curved surface area of the cylinder is 4πr2 and the ratio between their area is 1:1.
☛ Related Questions:
- A hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin-plating it on the inside at the rate of ₹16 per 100 cm².
- Find the radius of a sphere whose surface area is 154 cm².
- The diameter of the moon is approximately one fourth of the diameter of the earth. Find the ratio of their surface areas.
- A hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
visual curriculum