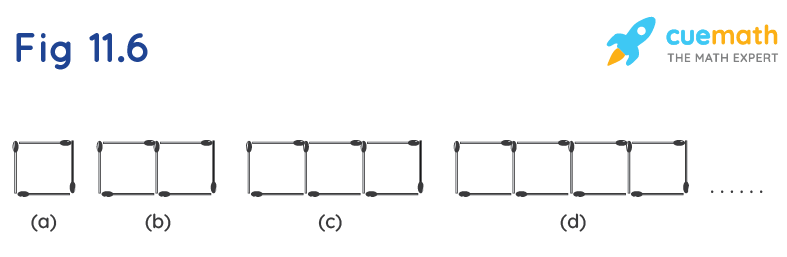
(a) Look at the following matchstick pattern of squares (Fig 11.6). The squares are not separate. Two neighbouring squares have a common matchstick. Observe the patterns and find the rule that gives the number of matchsticks in terms of the number of squares. (Hint : If you remove the vertical stick at the end, you will get a pattern of Cs.)
(b) Fig 11.7 gives a matchstick pattern of triangles. As in Exercise 11 (a) above, find the general rule that gives the number of matchsticks in terms of the number of triangles.

Solution:
a) By observing the pattern carefully, it can be concluded that the number of matchsticks is 4, 7, 10, and 13 which is 1 more than thrice the number of squares present.
i.e, 1 square = 3 × 1 + 1 = 4 matchsticks, 2 squares = 3 × 2 + 1 = 7 matchsticks and so on..
Thus, the rule for this pattern = 3n + 1 where, n = number of squares
b) Similarly, by observing the above matchstick pattern of triangles, we can conclude that every consecutive triangle shares a common matchstick.
The number of matchsticks in the first pattern is 3, i.e, 2 × 1 + 1 = 3
The number of matchsticks in the second pattern is 5, i.e, 2 × 2 + 1 = 5
The number of matchsticks in the third pattern is 7, i.e, 2 × 3 + 1 = 7
Thus, the pattern generated is adding 1 matchstick to twice the number of triangles present
Hence, the general rule for the pattern of triangles is 2n + 1 where, n = number of triangles
NCERT Solutions for Class 6 Maths Chapter 11 Exercise 11.1 Question 11
(a) Look at the following matchstick pattern of squares (Fig 11.6). The squares are not separate. Two neighbouring squares have a common matchstick. Observe the patterns and find the rule that gives the number of matchsticks in terms of the number of squares. (Hint : If you remove the vertical stick at the end, you will get a pattern of Cs.)
(b) Fig 11.7 gives a matchstick pattern of triangles. As in Exercise 11 (a) above, find the general rule that gives the number of matchsticks in terms of the number of triangles.
Summary:
(a)The pattern rule for the number of matchsticks in the pattern of squares is 3n + 1, where n = number of squares
(b) The general rule for the matchstick pattern of triangles is 2n + 1, where n = number of triangles
☛ Related Questions:
- Find The Rule Which Gives The Number Of Matchsticks Required To Make The Following Matchsticks Patterns Use A Variable To Write The Rule A A Pattern Of Letter T As
- We Already Know The Rule For The Pattern Of Letters L C And F Some Of The Letters From Ql Given Above Give Us The Same Rule As That Given By L Which Are These Why Does This
- Cadets Are Marching In A Parade There Are 5 Cadets In A Row What Is The Rule Which Gives The Number Of Cadets Given The Number Of Rows 5n Is The Rule Which Gives The Number Of Cadets
- If There Are 50 Mangoes In A Box How Will You Write The Total Number Of Mangoes In Terms Of The Number Of Boxes
visual curriculum