A day full of math games & activities. Find one near you.

Volume

Volume is the measure of the capacity that an object holds. For example, if a cup can hold 100 ml of water up to the brim, its volume is said to be 100 ml. Volume can also be defined as the amount of space occupied by a 3-dimensional object. The volume of a solid like a cube or a cuboid is measured by counting the number of unit cubes it contains. The best way to visualize volume is to think of it in terms of the space enclosed/occupied by any 3-dimensional object or solid shape. This can be seen through a simple exercise at home:

Take a rectangular sheet of paper of length 'l' cm and width 'h' cm.
Join the opposite sides of the sheet of paper without folding the sheet.
You have made a 3-D object that encloses space inside it, from a 2-D sheet.

1.	Volume Definition
2.	Volume of 3d Shapes
3.	List of Volume Formulas
4.	How to Calculate the Volume?
5.	Units of Volume
6.	Volume Calculator
7.	FAQs on Volume

Volume Definition

Volume is defined as a capacity occupied by a three-dimensional solid shape. In any shape, it is hard to visualize but can be compared between shapes. For example, the volume of a compass box is greater than the volume of an eraser placed inside it. For calculating the area of any two-dimensional shape, we divide the portion into equal square units. Similarly, while calculating the volume of solid shapes we will divide it into equal cubical units. Let us learn how to calculate the volume of different solid shapes in our next section.

Volume of 3D-Shapes

Every object in our surroundings has a nature of occupying space. These real-life objects can be easily compared with the basic 3-D shapes. Let us have a look at the volume of these solid shapes in detail.

Volume of a Cuboid

Suppose we have some rectangular sheets with length 'l' and width 'b'. If we stack them one on top of the other up to height 'h', we get a cuboid of dimensions l, b, h. This can be seen in the following figure which shows the length, width (breadth), and height of the cuboid thus formed.

To calculate the amount of space enclosed by this cuboid, we use the formula: Volume of a Cuboid = l × b × h

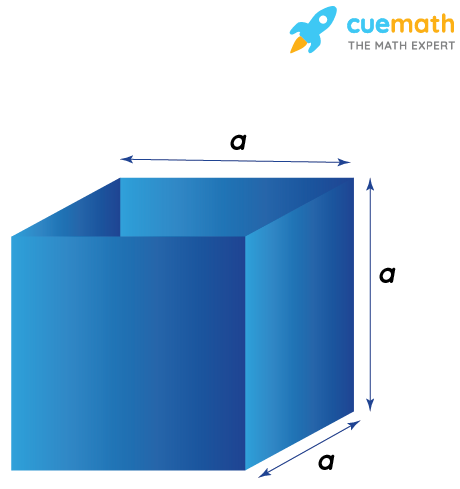
Volume of a Cube

A cube is a special case of a cuboid where all three sides are equal in measure. If we represent this equal value as ‘a’, then the volume of this cube can then be calculated with the formula: Volume of a Cube = a × a × a = a³. Observe the following figure to see the equal sides of a cube and the space it occupies.

Volume of a Cylinder

Just as we built up a cuboid using rectangles, we can build a cylinder using circles of the same size.

A cylinder is a tube-like structure with two parallel circular bases which are joined by a curved surface at a fixed distance from the center. The distance between these two bases is the height of the cylinder. If we consider 'r' as the radius of the circular base (and top) and 'h' as the height of the cylinder, then the volume of the cylinder can be expressed as Volume of a Cylinder = π r² h

Volume of a Pyramid

Pyramids have a polygon as their base and triangular faces that meet at the apex. The volume of a pyramid is calculated with the help of the formula: Volume of a Pyramid = 1/3 × Base length × Base width × height of the pyramid. This formula can also be written as 1/3 × Base area of the polygon × height of the pyramid.

Volume of Cone

The difference between a cone and a pyramid is that the base of a cone is circular whereas the base of a pyramid is a polygon. The volume of a cone is calculated with the formula: 1/3 ×πr²h.

Volume of Sphere

The volume of a sphere is the space occupied by it.

sphere with radius r
The volume of a sphere whose radius r is 4/3 πr³.

Now that we are familiar with the formulas of various geometric shapes, let’s take a look at the different units of volume.

List of Volume Formulas

Given below is a detailed tabular list of volume formulas in a nutshell describing volume formulas for all the possible 3-D (solid) shapes.

Shape	Volume
Cube	a³
Cuboid	l × b × h
Cone	(1/3)πr²h
Cylinder	πr²h
Sphere	(4/3)πr³
Hemisphere	(2/3)πr³
Prism	B × h
Pyramid	(1/3) (Bh)

How To Calculate the Volume?

Here are the steps to calculate volume of any solid shape:

Identify all the given parameters that are useful and are required to substitute in the respective volume formula. Such as the radius to be 'r' and height to be 'h', the slant height, diameter, etc.
Make sure all the parameters are of the same units.
Substitute the values in the volume formula of the respective shapes.
Write the units as cubic units.

Let us understand the steps with help of an example.

Example: Find the volume of a right circular cylinder of radius 25 m and height 1 meter. Use π = 3.142.

Solution:

The radius of the cylinder is, r = 25 m.
Its height is h = 1 meter.
Its volume of cylinder is, V = πr²h = (3.142)(25)²(1) = 1963.75m³.
The volume of a cylinder is 1963.75 cubic meters.

Units of Volume

The S.I. unit of volume is cubic meter (m³) since volume is a quantity of the three-dimensional space occupied by a shape or surface. However, the most commonly used unit for volume is liter. Apart from this, large and small volumes are measured in other units like milliliter (ml), pints, gallons, and others. The following table shows a few units related to volume and its metric equivalents.

Unit of Side	Unit of Volume	Metric Equivalent
Inch	Cubic Inches (in³)	1 cu.in = 16.387064 ml
Foot	Cubic Feet (ft³)	1 cu.ft = 28.316846592 l
cm	Cubic centimeter (cm³)	1 cubic centimeter = 1 ml
Yard	Cubic Yard (yd³)	1 cu.yd = 764.554857984 L 1 cu.yd = 0.764554857984 m³

While the US standard unit is a cubic yard or cubic inch, the more widely used units are gallons, pints, or fluid ounces. The following table shows a few of these units and their equivalent metric conversions.

Unit	Equivalent conversion
1 Pint (pt)	2 cups
1 Quart (qt)	2 pt
1 Gallon (gal)	3.78 liters
1 liter	1000 cubic centimeter

Volume Calculator

The volume calculator helps to calculate the volume of any given shape quickly and easily. Volume is a branch of mathematics that deals with measuring the capacity of different solid shapes. Try Cuemath volume calculator now. It is an online tool available for easy and fast calculations.

☛Also check:

☛Related Articles

Check out the articles related to the volume of different solid shapes.

Volume Examples

Example 1: Edwin has a conical vessel with a radius of 6 inches and a height of 7 inches. What is the volume of the vessel? Use π = 22/7.

Solution.
The vessel is in the shape of a cone.
Volume of cone = 1/3 π r² h = 1/3 × 22/7 × 6 × 6 × 7 = 264 in³
∴ Volume of the vessel is 264 in³
Example 2: Joe loves playing with building blocks. He built a structure with 15 cubes. If the length (edge) of each cube is 3 inches, what would be the volume of his structure?

Solution.
Let's calculate the volume of one cube. Volume of Cube = Edge × Edge × Edge = 3 × 3 × 3 = 27 in³
There are 15 cubes in his structure. So, the volume of the whole structure is:
Volume of structure =15 × volume of one cube = 15 × 27 = 405 in³
∴ Volume of the structure is 405 in³.
Example 3: If the diameter of a ball is 14 inches, how much air can the ball hold? Use π = 3.14

Solution.
The amount of air inside the ball will occupy the entire space in the ball. So, we need to find the volume of the ball.
The radius of the ball is 14/2 inches = 7 inches
Volume of the ball = 4/3 πr³
= [4/3 × 3.14 × (7)³]
= 1436.02 in³
∴ The ball contains 1436.02 in³ of air.

go to slide go to slide go to slide

Have questions on basic mathematical concepts?

Become a problem-solving champ using logic, not rules. Learn the why behind math with our certified experts

Book a Free Trial Class

Practice Questions on volume

go to slide go to slide

FAQs on Volume

What is the Meaning of Volume?

Volume is the measure of the capacity that an object holds. Let say, if a cup can hold 1000 ml of juice, its volume is said to be 1000 ml. In this case, volume can also be defined as the amount of juice occupied by a cup. Volume is always calculated by dividing the capacity of shapes into equal cubical units.

☛Download now for more practice.

What is the Formula of the Volume of a Partial Cone?

The volume of a partial cone can be calculated with the help of the formula: V = 1/3 × πh(R² + Rr + r²), where 'R' is the radius of the base of the cone and 'r' is the radius of the top surface.

☛ Read basics here:

How Do you Find the Volume of Pyramids?

The volume of a pyramid is calculated with the help of the formula: V = 1/3 × Base Area × Height.

☛ Also Check:

How Do We Find the Volume of a Cone?

The volume of a cone is 1/3rd of the volume of a cylinder with the same height and same base. The formula used to find the volume of a cone is 1/3 × π r² h; where 'r' is the radius and 'h' is the height of the cone.

How Do We Find the Volume of a Cylinder?

The volume of a cylinder is calculated with the help of the formula: V = Area of the base of a cylinder × Height, and is also represented as volume of a cylinder = π r² h; where 'r' is the radius of the cylinder and 'h' is the height.

What is the Difference Between Volume and Area?

The volume of a shape or solid is the space occupied by it, which also includes its height or depth. It is measured in cubic units. The area is the space occupied by the surface of a flat shape. It is measured in square units.

☛ Check the list of important formulas in math:

What is the Volume of a ball?

Since a ball is a sphere, its volume will be calculated using the formula for the volume of a sphere. The formula for the volume of a sphere is 4/3 πr³, where 'r' is the radius of the sphere.

What Is the Formula of the Volume of a Cylinder?

The formula for the volume of a cylinder = πr²h; where 'r' is the radius of the base of the cylinder and 'h' is the height.

How to Find the Volume of Prism?

We can find the volume of the prism by writing the given dimensions of the prism. Then substituting the values in volume formula V = B × H where "V", "B", and "H" are the volume, base area, and height of the prism. Once the value of the volume of the prism is obtained, write the unit of volume of the prism in the end (in terms of cubic units).

☛Check:

How do you Find the Volume of a Tank?

The volume of a tank depends on its shape. Depending on the size we can use the formulas given below:

Volume of a cuboid = l × b × h; where 'l' is length of the cuboid, 'b' is breadth (width) of the cuboid and 'h' is height of the cuboid.
Volume of a cube = a³, where 'a' is edge of the cube.
Volume of a cylinder = π r²h; where 'r' is radius of the base of the cylinder and 'h' is the height of the cylinder.
Volume of a cone = 1/3 π r² h, here, 'r' is radius of the circular base of the cone and 'h' is the height of the cone.
Volume of a sphere = 4/3 πr³, where 'r' is the radius of the sphere.

Download FREE Study Materials

Worksheets on Volume