Surface Area of Triangular Pyramid Formula
The surface area of a triangular pyramid is the total area of all faces of a triangular pyramid. Basically, a triangular pyramid has a triangular base and is bounded by three lateral triangular faces that meet at one vertex. A triangular pyramid has all faces as triangles. This pyramid has 4 faces, 6 edges, and 4 corners or vertices. Few types of the triangular pyramid are given below:
- Regular triangular pyramid - all faces are equilateral triangles and are known as tetrahedrons.
- Right triangular pyramid - the base is an equilateral triangle while other faces are isosceles triangles.
- An irregular triangular pyramid - a scalene or isosceles triangle forms the base.
What Is The Surface Area of a Triangular Pyramid?
The surface area of any three-dimensional geometrical shape is the sum of the areas of all of the faces or surfaces of that enclosed solid. A triangular pyramid has four triangular faces. Thus, The formula for calculating the surface area of a triangular pyramid involves the area of the base, the perimeter of the base, and the slant height of any side of the pyramid. The surface area is always measured in square units like cm2, m2, ft2, or cubits2. The surface area of a triangular pyramid is \(\begin{align} \text {Base Area}+\!\frac{1}{2} \text {(Perimeter}\!\times\!\text {Slant Height}) \end{align}\).
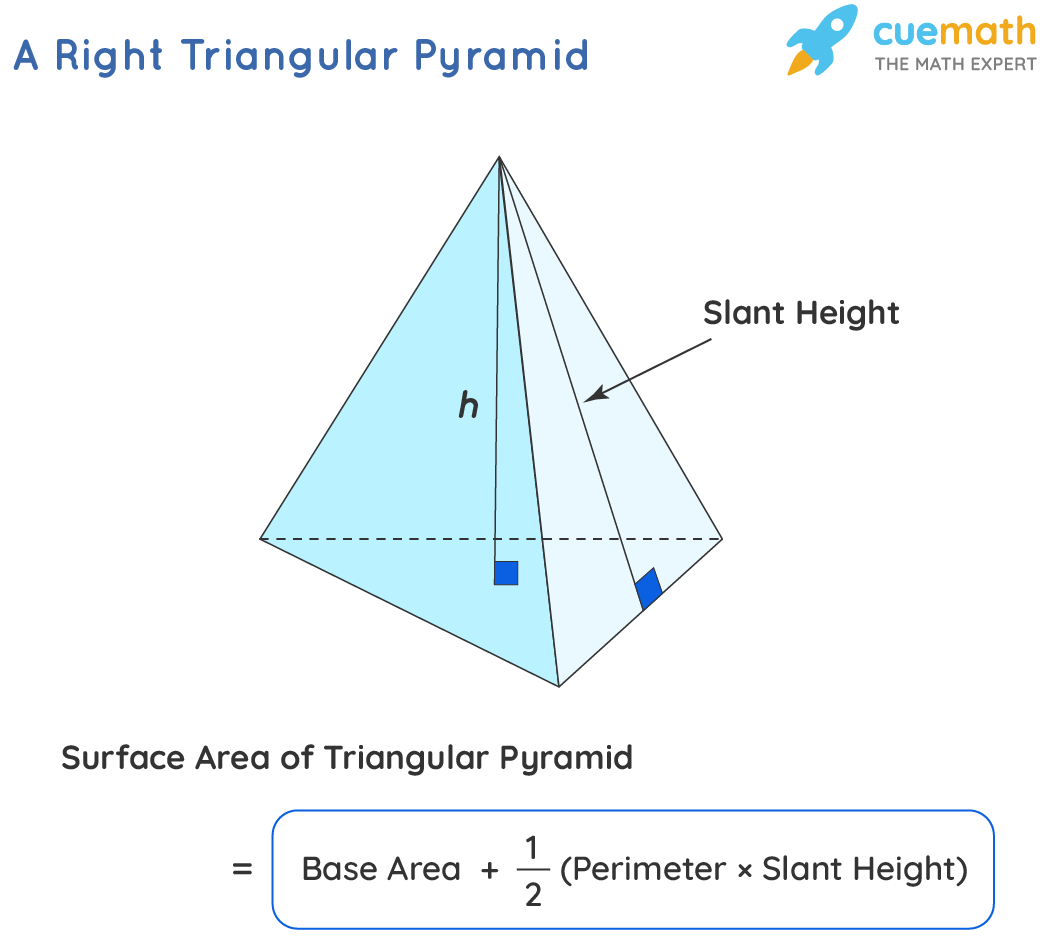
Surface Area of Triangular Pyramid Formula
The Formula for the surface area of a triangular pyramid is calculated by adding up the area of all triangular faces of a pyramid. The surface area of a right triangular pyramid formula is \(\begin{align} \text {Base Area}+\!\frac{1}{2} \text {(Perimeter}\!\times\!\text {Slant Height}) \end{align}\).
After putting the values we get an expression of the surface area of the triangular pyramid formula as 1⁄2(a × b) + 3⁄2(b × s).
Where,
- b is the side of the triangle pyramid.
- a is the height of the base triangle
- s is the slant height of a triangular pyramid.
- 1⁄2(a × b) is the base area of the triangular faces.
- 3⁄2(b × s) is the product of the perimeter and slant height of a pyramid.
How to Calculate the Surface Area of Triangular Pyramids?
The surface area of a triangular pyramid can be calculated by representing the 3D shape into a 2D net, to make the shapes easier to see. After expanding the 3D shape into 2D shape we will get four triangles.
The following steps are used to calculate the surface area of a triangular pyramid:
- Step 1: Find the area of the base triangles: The area of the base triangles is (1/2 × base of the triangle × height of the triangle) which becomes base × height.
- Step 2: Find the perimeter of triangular faces: The perimeter of a triangle is the sum of all sides of a triangle which is \((side)_{1}\) + \((side)_{2}\) + \((side)_{3}\).
- Step 3: Find the slant height of triangular faces: The slant height of a triangular pyramid is generally represented by 's'.
- Step 4: Add all the areas together. Thus, the surface area of a triangular pyramid formula is 1⁄2(a × b) + 3⁄2(b × s) in squared units.
Lateral Surface Area of Triangular Pyramid
The lateral surface area is the area of the non-base faces or we can say that only the lateral surface area of any object is calculated by removing the base area. The lateral area of a triangular pyramid can be calculated by removing the base area of a triangle from the product of the perimeter of the base and the slant height of a pyramid.
Thus, the lateral surface area of a right triangular pyramid is 1⁄2(perimeter of the base × slant height) which further becomes 3⁄2(side × slant height).
Where,
- b is the side of a pyramid.
- s is the slant height of the base.
Examples on Surface Area of Triangular Pyramid Formula
-
Example 1: Each side of a triangular pyramid is of length 3 units, the height of the base triangle is 6, and the slant height is 5. Find its total surface area.
Solution
The surface area of a triangular pyramid of side a is
Surface Area = 1⁄2(a × b) + 3⁄2(b × s)
On Substituting the values, we get,
Surface Area = 1⁄2(6 × 3) + 3⁄2(3 × 5)
Surface Area = (9) + (22.5) = 31.5 units2
Answer: The total Surface Area of the triangular pyramid is 31.5 units2.
-
Example 2: Find the surface area of a triangular pyramid whose area of the base triangle is 24 units2, the perimeter is 12 units, and the slant height of the pyramid is 18.
Solution
The surface area of a triangular pyramid of side a is
Surface Area = 1⁄2(a × b) + 3⁄2(b × s)
The surface area of a triangular pyramid = \(\begin{align} \text {Base Area}+\!\frac{1}{2} \text {(Perimeter}\!\times\!\text {Slant Height}) \end{align}\)
Putting the values in the formula,
The surface area of a triangular pyramid = \(\begin{align} \text {24}+\!\frac{1}{2} \text {(12}\!\times\!\text {18}) \end{align}\)
= 132 square units.
Answer: The surface area of a triangular pyramid is 96 units2.

Practice Questions on Surface Area of Triangular Pyramid Formula
FAQs on Surface Area of Triangular Pyramid Formula
How Do You Find the Surface Area of a Triangular Pyramid?
The Formula for the surface area of a pyramid is calculated by adding up the area of all triangular faces of a pyramid. which is 1⁄2(a × b) + 3⁄2(b × s). Where b is the side of a pyramid, a is the height of a base triangle, and s is the slant height of a pyramid.
What Is the Formula for the Volume of a Triangular Pyramid?
The volume of a triangular pyramid can be found by using the formula, 1/3 × Base Area × Height.
How Do You Find the Area of the Base of a Right Triangular Pyramid?
The area of the base of a right triangular pyramid is 1/2 × height of a base triangle × the bottom edge of the base triangle.
What Is the Lateral Surface of a Triangular Pyramid?
The lateral surface of a triangular pyramid is calculated following the steps given below.
- Step 1: Look for the given parameters.
- Step 2: Multiply 1/3 with the perimeter of the base triangle and the slant height of a triangular pyramid.
- Step 3: Write the result in squared units.
How To Find the Total Surface Area of a Triangular Pyramid When Its Lateral Surface Area and Base area are Given?
The formula to calculate the total surface area of a triangular pyramid is 1⁄2(a × b) + 3⁄2(b × s).
- Step 1: Check for the given parameters.
- Step 2: Add the value of its lateral surface area and the base area.
- Step 3: Write the sum so obtained in squared units.
visual curriculum
