Perimeter of a Triangle
The perimeter of a triangle is defined as the total length of its boundary. A triangle is a polygon with 3 sides and it can be classified into different types based on the measure of its sides and angles. There are different formulas and methods to calculate the perimeter of a triangle based on the type of triangle. Let us learn how to find the perimeter of a triangle using the perimeter of triangle formula.
| 1. | What is the Perimeter of a Triangle? |
| 2. | Perimeter of a Triangle Formula |
| 3. | How to Find the Perimeter of a Triangle? |
| 4. | FAQs on Perimeter of a Triangle |
What is the Perimeter of a Triangle?
The perimeter of a triangle means the sum of all three sides. The word perimeter is a combination of two Greek words - 'peri' which means around and 'metron' which means measure. The total distance around any 2D shape is defined as its perimeter. Since the perimeter gives the length of the boundary of a shape, it is expressed in linear units.
Real-Life Example of Triangle's Perimeter: Imagine that we need to fence the triangular park shown below. Now, to know the dimensions of the fence, we add the lengths of the three sides of the park. This length or distance of the boundary of a triangle is called the perimeter of the triangle.

Perimeter of a Triangle Formula
To calculate the perimeter of a triangle, we simply add the lengths of the sides given. The basic formula used to calculate the perimeter of a triangle is:
Perimeter = sum of the three sides
Let us understand this formula with the different types of triangles.
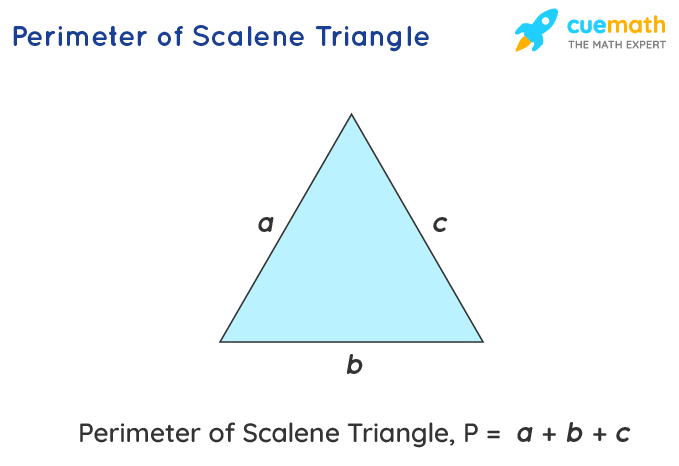
Perimeter of a Scalene Triangle
If a triangle has all three sides of different lengths, it is a scalene triangle. The perimeter of a scalene triangle can be calculated by finding the sum of all the unequal sides. The formula for the perimeter of a scalene triangle is Perimeter = a + b + c, where 'a', 'b', and 'c' are the three different sides.
Perimeter of an Isosceles Triangle
If a triangle has two sides of equal length, it is an isosceles triangle. The perimeter of an isosceles triangle can be calculated by finding the sum of the equal and unequal sides. The formula for the perimeter of an isosceles triangle is: Perimeter of an isosceles triangle = 2a + b
where,
- a = sides of equal length
- b = the third side
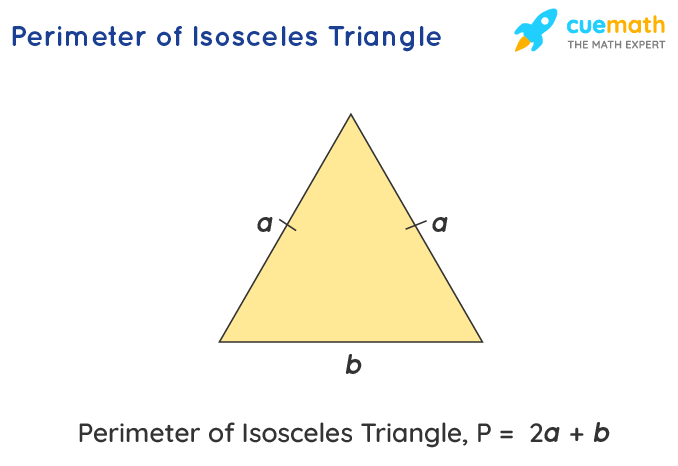
Perimeter of an Equilateral Triangle
An equilateral triangle has all the sides of equal measure. The formula for the perimeter of an equilateral triangle is:
Perimeter of an equilateral triangle = (3 × a)
where 'a' = length of each side of the triangle.

Perimeter of a Right Triangle
A triangle that has one of the angles as 90° is called a right-angled triangle or a right triangle. The perimeter of a right triangle can be calculated by adding the given sides. The formula to calculate the perimeter of a right triangle is:
Perimeter of a right triangle, P = a + b + c
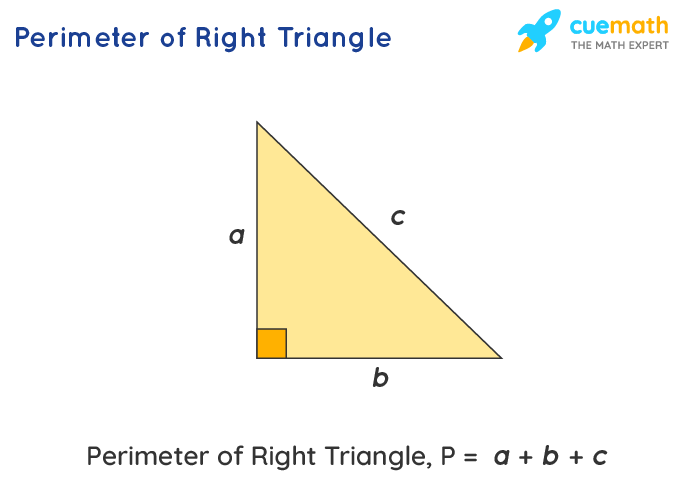
Since this is a right triangle, we can use the Pythagoras theorem, if any one side of this triangle is not known. The Pythagoras theorem says that the square of the hypotenuse is equal to the sum of squares of the other two sides. Referring to the figure given above:
- a = Perpendicular
- b = Base
- c = Hypotenuse of the right triangle
Hence, according to the Pythagoras theorem, c2 = a2 + b2. In this case, the perimeter of a right triangle can also be written as: P = a + b + √(a2 + b2). This is because c2 = a2 + b2 , therefore, c = √(a2 + b2).
Perimeter of Isosceles Right Triangle
A right triangle with two equal sides and two equal angles is called an isosceles right triangle. The perimeter of an isosceles right triangle can be calculated by adding the given sides.

The formula to calculate the perimeter of an isosceles right triangle is P = 2l + h, where l is the length of two equal legs or sides of the triangle, and h is the hypotenuse. Now, let us see how we can express 'h' in terms of 'l' and vice versa to find the perimeter of a triangle if only 'h', or, only 'l' is given.
- Using the Pythagoras theorem, we know, h = √(l2 + l2), which can further be simplified as, h = √2 × l, or, l = h/√2.
- Now, using these expressions, the perimeter of an isosceles right triangle can also be calculated if we know only 'l'. This will be, P = 2l + √2l, where we have replaced 'h' as (√2l), so, P = (2 + √2)l
- Similarly, the perimeter can be calculated if we know only 'h'. When we can express 'l' in terms of 'h', we get, l = h/√2.This will be, P = 2(h/√2) + h = (√2 × h) + h
How to Find The Perimeter of a Triangle?
The perimeter of a triangle can be calculated by following the steps given below:
- Step 1: Note the measurements of all the sides of a triangle and check that all the sides should have the same unit.
- Step 2: Calculate the sum of all the sides.
- Step 3: Give the answer along with the unit.
Let us see how to find the perimeter of a triangle using an example.
Example: Find the perimeter of △ABC having the following dimensions: AB = 6 inches, BC = 8 inches, AC = 10 inches.
Solution:
Step 1: Check if all three sides of the triangle are known.
AB = 6 inches, BC = 8 inches, AC = 10 inches
Step 2: Use the appropriate formula and add the sides to get the perimeter. Since this is a scalene triangle, we use the formula, Perimeter = a + b + c. Write the perimeter along with its units.
Perimeter of triangle ABC = 6 + 8 + 10 = 24 inches.
☛ Related Articles
Check out some interesting articles related to the perimeter of a triangle.
Perimeter of a Triangle Examples
-
Example 1: Find the perimeter of a right triangle PQR having PR as the hypotenuse and with sides PQ = 4 inches, and QR = 3 inches.
Solution:
Given, PQ = 4 inches, QR = 3 inches, PR = ?
To calculate the perimeter of the triangle, we need to know all three sides.
We will calculate the length of the hypotenuse (PR) using the Pythagoras theorem.
PR² = PQ² + QR²
PR² = 4² + 3²
PR² = 16 + 9
Therefore, PR = √25 inches
PR = 5 inches.
Now, we can calculate the perimeter of the triangle.
Perimeter of triangle PQR = Sum of the three sides
= 3 + 4 + 5 = 12
Therefore, the perimeter is 12 inches.
-
Example 2: Find the length of the missing side of a triangular-shaped road sign whose perimeter is 48 inches and the two sides are 17 inches each.
Solution:
Let the length of the missing side be b.
Given, Perimeter = 48 inches
Length of the two equal sides = 17 inches each
Perimeter of a triangle = sum of lengths of three sides
48 = 17 + 17 + b
48 = 34 + b
b = 14
Therefore, b = 14 inches
Answer: Length of the missing side = 14 inches.
-
Example 3: The perimeter of a rectangular wire is 297 inches. The same wire is bent into the shape of an equilateral triangle. Find the length of each of its sides.
Solution:
We know that, the perimeter of a rectangle = total length of the wire
Length of the wire used = Perimeter of the triangle formed
Perimeter of an equilateral triangle = 3 × a
297 = 3 × a
a = 99
Answer: The length of each side of the triangle = 99 inches

FAQs on Perimeter of Triangle
What is the Perimeter of a Triangle in Math?
The perimeter of a triangle is defined as the total length of its boundary. It is the sum of all three sides of the triangle and is expressed in linear units.
What is the Formula of Perimeter of Triangle?
The perimeter of a triangle can be calculated by simply adding the length of all the sides. The basic perimeter of triangle formula which is used to calculate the perimeter is, perimeter of triangle = a + b + c, where, 'a', 'b', and 'c' are the sides of the triangle.
How to Find the Perimeter of a Triangle With Three Equal Sides?
To calculate the perimeter of a triangle with three equal sides, we add the length of all sides or multiply the length of any one side by 3. Such a triangle is called an equilateral triangle. The formula to calculate the perimeter of an equilateral triangle is 3a, where 'a' is the length of each side.
Can a Triangle Have the Same Area and Perimeter?
A triangle can have the same perimeter and area only in some special cases. These shapes having an equal perimeter and area are called equable shapes. Thus, a triangle with an equal perimeter and area is called an equable triangle.
How to Find the Third Side and Perimeter of a Right Triangle Given Two Sides?
The third side of a right-angled triangle can be calculated using the measure of the other two sides, by applying the Pythagoras theorem. According to the Pythagoras theorem, for any right-triangle with sides 'a', 'b', and 'c',
c2 = a2 + b2
where,
- a = Perpendicular
- b = Base
- c = Hypotenuse of the right triangle
The perimeter of a right triangle is calculated with the formula: Perimeter = a + b + c
How to Find the Perimeter of a Triangle With Coordinates?
If the coordinates of a triangle are given, then the length of all its sides can be calculated using the distance formula. Once these lengths are obtained, we can simply add them to find the perimeter of the given triangle.
How to get the Perimeter of a Triangle With Two Equal Sides?
To calculate the perimeter of a triangle with two equal sides, we find the sum of lengths of all the sides. This type of triangle is called an isosceles triangle. The formula to calculate the perimeter of an isosceles triangle is 2a + b, where 'a' is the length of one of the equal sides, and 'b' is the length of the third side.
How to Find the Area and Perimeter of a Triangle?
The area of a triangle is the space occupied by it. The area of a triangle depends on the kind of triangle and the parameters that are known. The basic formula for the area of a triangle is, Area of triangle = 1/2 × b × h, where b = base, and h = height. It should be noted that the area of triangle is expressed in square units.
The perimeter of a triangle is defined as the total length of its boundary. This is calculated by adding the three sides of the triangle. So, the basic formula for the perimeter of triangle is, perimeter of triangle = a + b + c, where a, b, and c are the sides of the triangle. It should be noted that the perimeter of a triangle is expressed in linear units.
What is the Circumference of a Triangle?
The circumference of a triangle is another name for the perimeter of a triangle. This means that the circumference of a triangle is the total length of its boundary. Therefore, the circumference of triangle formula is expressed as, circumference of a triangle = a + b + c, where a, b, and c are the sides of the triangle.
visual curriculum
