Diameter of a Sphere Formula Using Volume

The volume of a sphere formula can be found in terms of the diameter. The diameter of a sphere is the longest line that is inside the sphere and that passes through the center of the sphere. A sphere is a three-dimensional shape that is perfectly symmetrical and round in shape. Some examples of spheres are a ball, a globe, etc. The volume of a sphere is the amount of space that is inside it (or) the capacity of the sphere that it can hold. In this article, we will derive the diameter of a sphere formula using the volume.

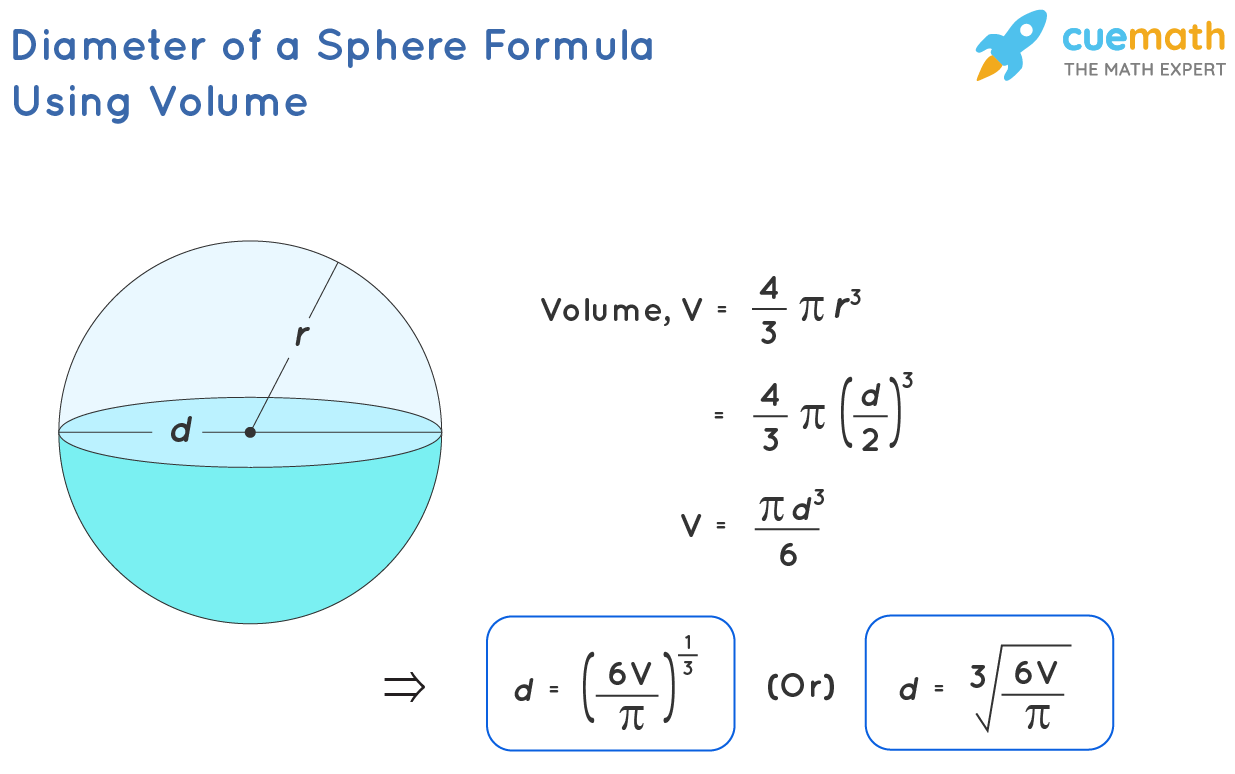
We know that the volume, V of a sphere of radius 'r' is calculated using the formula V = (4/3) π r³. This is the formula for the volume of a sphere in terms of radius. We know that the diameter of a sphere is twice the radius. i.e., the diameter, d = 2r. From this, we get r = d/2. Substituting this in the volume formula,

V = (4/3) π (d/2)³ = (4/3) π (d³/8) = (πd³)/6.

Thus the volume of a sphere in terms of diameter (d) is, V = (πd³)/6.

In the previous section, we have already learned the formula of finding the volume (V) of a sphere using its diameter (d). We can simply solve this formula for the diameter (d) to find the diameter of a sphere formula using its volume. The volume of a sphere using diameter is,
V = (πd³)/6
⇒ 6V = πd³ (Multiplied both sides by 6)
⇒ 6V/π = d³ (Divided both sides by π)
⇒ (6V/π)^(1/3) = d (raised the power on both sides by 1/3)

Thus, the formula for the diameter of a sphere using its volume is, d = (6V/π)^(1/3).

Note: Here π is a constant which is approximately equal to 22/7 (or) 3.14159...

Let us consider a sphere of radius r, diameter d, and volume V. We can calculate the diameter of the sphere using its volume in 3 ways:

1. Substitute the value of V in the volume of a sphere formula in terms of radius, V = (4/3) π r³, solve it for r. Then use d = 2r.
2. Substitute the value of V in the volume of a sphere formula in terms of diameter, V = (πd³)/6, solve it for d.
3. Directly substitute the value of V in the diameter of a sphere formula using volume, d = (6V/π)^(1/3).

How To Calculate the Volume of a Sphere With Diameter?

The volume of a sphere (V) in terms of its radius (r) is V = (4/3) π r³. If d is its diameter, we have d = 2r. From this, we get r = (d/2). Substituting this in the volume formula, the volume of a sphere in terms of diameter is V = (πd³)/6.

How Do You Find the Diameter of a Sphere From the Volume?

There are multiple methods to find the diameter (d) of a sphere from the volume (V). One of the methods is to substitute V directly into the formula, d = (6V/π)^(1/3). Alternatively, we can substitute V into the volume of a sphere formula, V = (4/3) π r³, solve for r, and double the radius (r) to find the diameter.

What Is the Diameter of a Sphere Formula Using Volume?

The diameter of a sphere formula with volume is, d = (6V/π)^(1/3), where d is the diameter of the sphere and V is its volume. Alternatively, we can substitute the value of V, in the volume formula, V = (4/3) π r³, solve it for r, and make it double to find the diameter.

What Is the Relation Between the Diameter and Volume of a Sphere?

The relationship between the diameter of a sphere and its volume can be studied from the formula, V = (πd³)/6, where, d is the diameter and V s the volume of the sphere.

What Is the Formula to Calculate the Radius and Diameter of Sphere Using Volume?

The radius and diameter of a sphere can be calculated, given the volume. The formulas that can be used to find the radius and diameter are given below,

• Formula for radius, r of sphere using Volume, V = (4/3) π r³.
• Formula for diameter, d of sphere using Volume, V = (πd³)/6.