Linear Approximation Formula
The concept behind the linear approximation formula is the equation of a tangent line. We know that the slope of the tangent that is drawn to a curve y = f(x) at x = a is its derivative at that point. i.e., the slope of the tangent line is f'(a). Thus, the linear approximation formula is an application of derivatives. Let us learn more about this formula in the upcoming sections.
What is Linear Approximation?
The linear approximation of a function is nothing but approximating the value of the function at a point using a line. As soon as we see a curve (of a function) and a point on it, we remember the concept of the tangent line. If we find the equation of the tangent line at the given point, the value of the function at any point that is very close to the given point can be approximated using the equation of the tangent line. This concept is known as the linear approximation and since it is done using the tangent line, it is also known as the tangent line approximation.
Linear Approximation Formula
As we discussed in the previous section, the linear approximation formula is nothing but the equation of a tangent line. Let us find the equation of a tangent line that is drawn to the curve y = f(x) at the point x = a (or) (a, f(a)). As we know the slope of this tangent is the derivative f '(a), its equation using the point-slope form is:
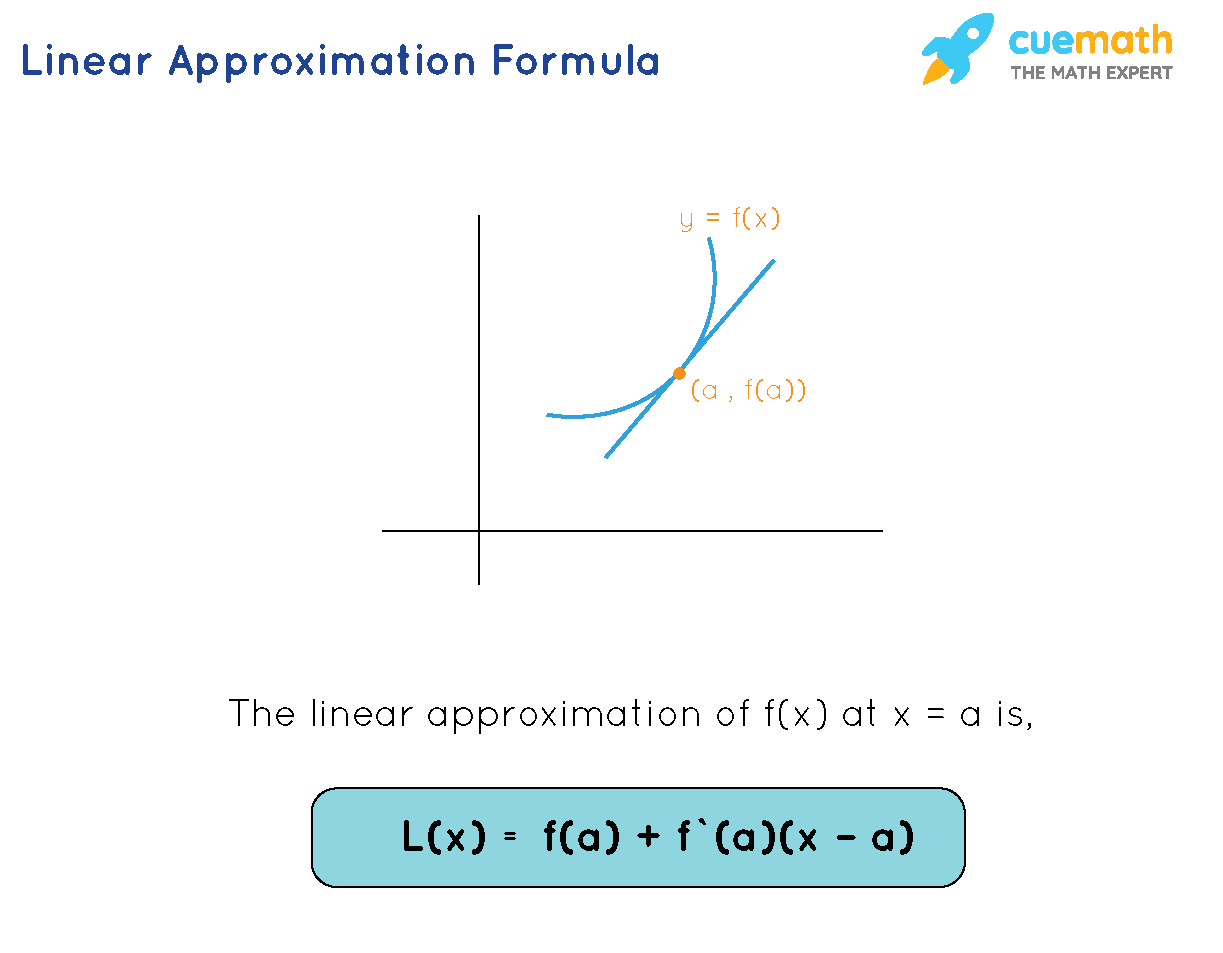
The linear approximation formula is:
L(x) = f(a) + f '(a) (x - a)
where,
- L(x) is the linear approximation of f(x) at x = a.
- f '(a) is the derivative of f(x) at x = a.

We will see the linear approximation formula in the upcoming section.
Examples Using Linear Approximation Formula
Example 1: Find the equation of linear approximation of the function f(x) = cos x at x = π/2.
Solution:
The given function is, f(x) = cos x.
We have to find the linear approximation of f(x) at a = π/2.
So f(a) = cos π/2 = 0.
The derivative of f(x) is,
f ' (x) = - sin x
f ' (a) = f ' (π/2) = - sin π/2 = -1.
The linear approximation formula of f(x) is,
L(x) = f(a) + f '(a) (x - a)
L(x) = 0 + (-1) (x - π/2)
L(x) = -x + π/2
Answer: The equation of linear approximation is, L(x) = -x + π/2.
Example 2: Find the linear approximation of f(x) = √x at x = 4. Using this, find the approximate value of √4.04.
Solution:
The given function is, f(x) = √x.
We have to find the linear approximation of f(x) at a = 4.
So f(a) = √4 = 2.
The derivative of f(x) is,
f ' (x) = d/dx (√x) = d/dx (x1/2) = 1/2 . x -1/2 = 1/ (2√x)
(for more detailed information of finding this derivative, click here)
f ' (a) = f ' (4) = 1/ (2√4) = 1/4.
The linear approximation formula of f(x) is,
L(x) = f(a) + f '(a) (x - a)
L(x) = 2 + 1/4 (x - 4)
L(x) = 2 + (1/4)x - 1
L(x) = (1/4)x + 1
Using this,
√4.04 ≈ L(4.04) = (1/4) (4.04) + 1 = 2.01
Answer: The linear approximation of f(x) is, L(x) = (1/4)x + 1 and √4.04 ≈ 2.01.
Example 3: Use the linear approximation formula to find the approximate value of ∛27.05. Round your answer to 4 decimals.
Solution:
Let us assume that f(x) = ∛x.
Since 27.05 is very close to 27, let us find the linear approximation of f(x) at x = 27. Thus, a = 27.
f(a) = ∛27 = 3.
The derivative of f(x) using the power rule is,
f ' (x) = d/dx (∛x) = d/dx (x1/3) = 1/3 . x -2/3
f ' (a) = f ' (27) = 1/3 . (27) -2/3 = 1/27
The linear approximation formula of f(x) is,
L(x) = f(a) + f '(a) (x - a)
L(x) = 3 + 1/27 (x - 27)
L(x) = 3 + (1/27)x - 1
L(x) = (1/27)x + 2
Using this,
∛27.05 ≈ L(27.05) = (1/27)(27.05) + 2 = 3.0019
Answer: ∛27.05 ≈ 3.0019.
FAQs on Linear Approximation Formula
What is Linear Approximation Formula?
The linear approximation formula, as its name suggests, is a function that is used to approximate the value of a function at the nearest values of a fixed value. The linear approximation L(x) of a function f(x) at x = a is, L(x) = f(a) + f '(a) (x - a).
How To Use Linear Approximation Formula?
We can use the linear approximation of a function f(x) to find the values of f(x) at the nearest values of a fixed number x = a. The linear approximation is denoted by L(x) and is found using the formula L(x) = f(a) + f '(a) (x - a), where f '(a) is the derivative of f(x) at a x = a.
What Is Linear Approximation Formula Based On?
The linear approximation formula is based on the equation of the tangent line of a function at a fixed point. The linear approximation of a function f(x) at a fixed value x = a is given by L(x) = f(a) + f '(a) (x - a).
What Is √8 Using Linear Approximation Formula?
We know that √9 = 3. Thus, we define the function as f(x) = √x and a = 9. Then f(a) = √9 = 3 and its derivative is f'(a) = 1 / (2√x) = 1 / (2 · 3) = 1/6. Using the linear approximation formula,
L(x) = f(a) + f '(a) (x - a)
L(x) = 3 + (1/6) (x - 9)
L(x) = 3 + (1/6) x - 3/2
L(x) = (1/6) x + 3/2
To find √8, we have to substitute x = 8 in the above approximation.
√8 ≈ L(8) = (1/6) (8) + 3/2 ≈ 2.833.
visual curriculum
