Limit Formula

The limit formula is used to calculate the derivative of a function. The limit is the value of the function approaches as the input approaches mentioned value. Limits are used as a way of making approximations used in the calculation as close as possible to the actual value of the quantity. Let us understand the limit formula in detail in the following section.

What is Limit Formula?

The limit formula is the representation of the behavior of the function at a specific point and the formula analyzes that function. Limit describes the behavior of some quantity that depends on an independent variable, as that independent variable approaches or comes close to a particular value. 

Limit Formula

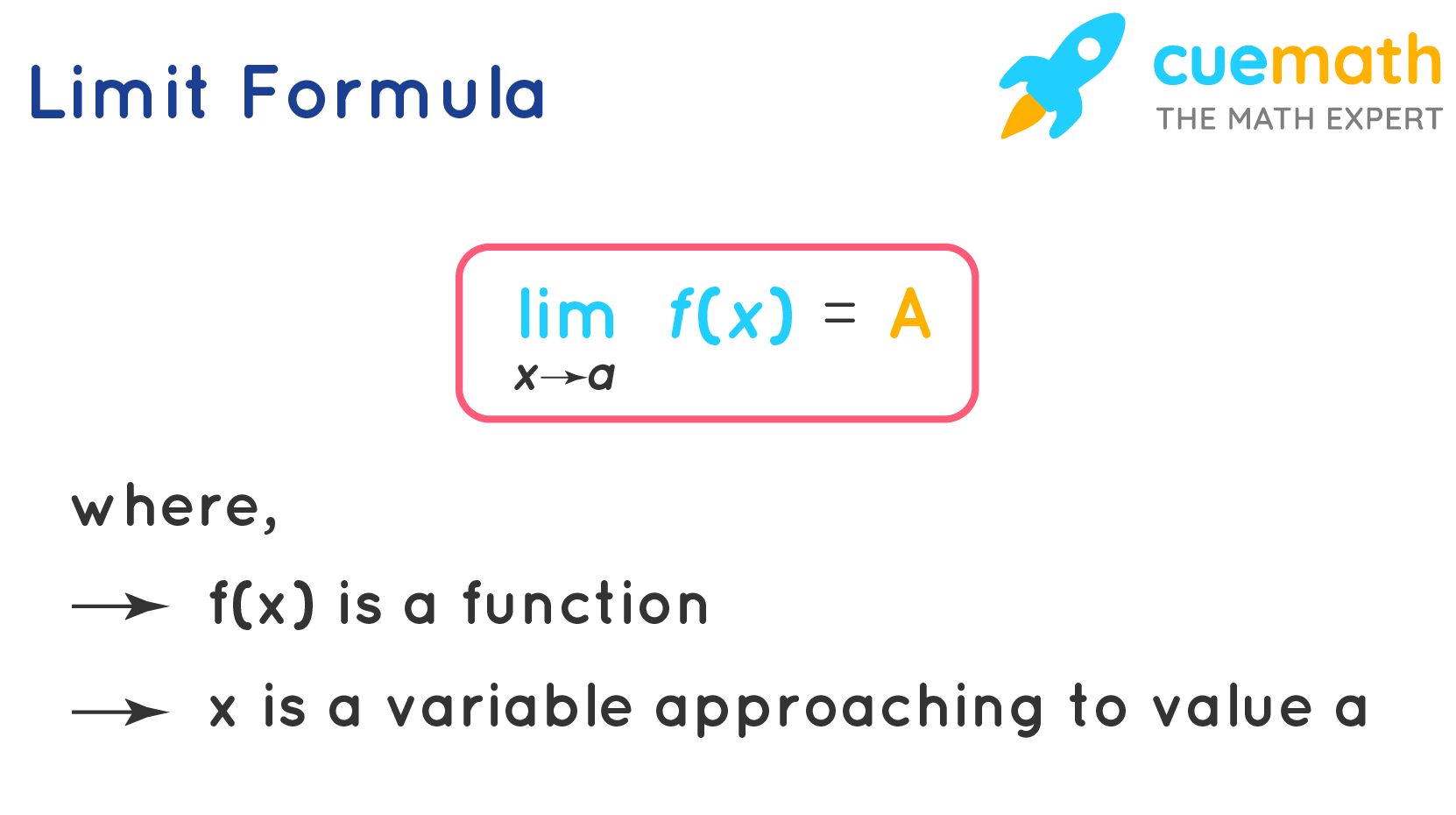
Let y = f(x) as a function of x.  If at a point x = a, f(x) takes indeterminate form, then we can consider the values of the function which is very near to a. If these values tend to some definite unique number as x tends to a, then that obtained a unique number is called the limit of f(x) at x = a. We can write the formula as:

\(\mathop {\lim }\limits_{x \to a} f(x) = A \)

where,

• f(x) is a function
• x is a variable approaching to value a

It is read as the limit of a function of x equals A as and when x approaches a.

Limits Formulas 

The formulas mentioned in the image below are a few limits formulas, 

Properties of Limit Formula

There a few properties of the limit formula used while calculation, they are as shown below.

Examples Using Limit Formula

Example 1: Using the limit formula, find the value of \(\mathop {\lim }\limits_{x \to 2} x^2 + 5.\)

Solution:

Putting values in the limit formula,

\(\mathop {\lim }\limits_{x \to 2} x^2 + 5 = 2^2 + 5= 9\)

Therefore, the value of: \(\mathop {\lim }\limits_{x \to 2} x^2 + 5\)is 9.

Example 2: Find the value of: \(\mathop {\lim }\limits_{x \to 0} 3x^3 + 4x+ 5. \)

Solution:       

Putting values in the limit formula,

\(\begin{align*}\mathop {\lim }\limits_{x \to 0} 3x^3 + 4x+ 5 &= 3(0)^3 + 4(0) + 5 \\  &= 5\end{align*} \)

Therefore, the value of: \(\mathop {\lim }\limits_{x \to 0} 3x^3 + 4x+ 5\) is 5.

Example 3: Using the limit formula, find the value of \(\mathop {\lim }\limits_{x \to 3} 6x^2 + 10x+ 15. \)

Solution:

Putting values in the limit formula,

\(\mathop {\lim }\limits_{x \to 3} 6x^2 + 10x + 15 = 6(3)^2 + 10(3) + 15 = 99\)

Therefore, the value of \(\mathop {\lim }\limits_{x \to 3} 6x^2 + 10x+ 15. \) is 99