Problems On Doing Geometry With Complex Numbers

Example - 37

If \(z{_1}\text{ and}\;z{_2}\)  are fixed and \(z\) satisfies

\[|z - {z_1}{|^2} + |z - {z_2}{|^2} = k,\]

find the possible values of  \(k \) so that this equation represents a circle.

Solution: Let us try to reduce (simplify) this equation

\[\begin{align}&\qquad \;\;|z - {z_1}{|^2} + |z - {z_2}{|^2} = k\\ &\Rightarrow \quad 2|z{|^2} - 2{\mathop{\rm Re}\nolimits} (z{{\bar z}_1} + z{{\bar z}_2}) + |{z_1}{|^2} + |{z_2}{|^2} = k\\ &\Rightarrow \quad |z{|^2} - {\mathop{\rm Re}\nolimits} \left( {z({{\bar z}_1} + {{\bar z}_2})} \right) = \frac{1}{2}\left( {k - |{z_1}{|^2} - |{z_2}{|^2}} \right)\end{align}\]

Careful thinking will show that the left hand side can be transformed into a perfect square with the following manipulation.

Thus, the centre is \(\begin{align}\,{z_0} = \frac{{{z_1} + {z_2}}}{2}\end{align}\) and the radius is \(\begin{align}\frac{1}{2}\sqrt {2k - |{z_1} - {z_2}{|^2}} .\end{align}\) For the radius to be defined the condition that \(k\) must satisfy is

\[k > \frac{1}{2}|{z_1} - {z_2}{|^2}\]

These are the possible values of \(k.\)

Example - 38

Let \(\bar bz + b\bar z = c,\,\,b \ne 1\) be a line in the complex plane. If a point \(z{_1}\)  is the reflection of a point \(z{_1}\) through the line, show that \(c = {\bar z_1}b + {z_2}\bar b,\)

Solution:

From the figure, it is obvious that the midpoint of \(AB\) must lie on the given line, i.e, \(\begin{align}&\frac{{{z_1} + {z_2}}}{2}\end{align}\) should satisfy the equation of the line. Also, \(AB\) must be perpendicular to the given line, i.e the complex slopes of \(AB\) and the given line must add to 0.

\[\begin{align} &\Rightarrow \,\,\,\bar b\left( {\frac{{{z_1} + {z_2}}}{2}} \right) + b\left( {\frac{{{{\bar z}_1} + {{\bar z}_2}}}{2}} \right) = c\,\,\,\,\,\,\,...{\text{ }}\left( 1 \right) \\\\ &\text{and}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\frac{{{z_1} - {z_2}}}{{{{\bar z}_1} - {{\bar z}_2}}} + \left( {\frac{{ - b}}{{\bar b}}} \right) = 0 \\\\&\Rightarrow \,\,\,\bar b\left( {{z_1} - {z_2}} \right) - b\left( {{{\bar z}_1} - {{\bar z}_2}} \right) = 0\,\,\,\,\,\,\,\,\,...{\text{ }}\left( 2 \right)\\\\& 2 \times \left( 1 \right) - \left( 2 \right)\,\,\,{\text{gives us the required relation.}}\\\end{align} \]

Example - 39

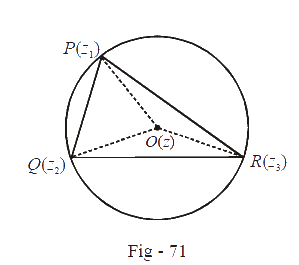
Find the circumcenter of the triangle whose vertices are given by the complex numbers \(z{_1}{\rm{ }},z{_2}{\rm{\;and\; }}z{_3}{\rm{ }}.\)

Solution:

We have to find \(z,\) the circumcenter \(O\) of triangle \(PQR.\) By virtue of being the circumcenter, \(z\) is equidistant from \(z{_1}{\rm{ }},z{_2}{\rm{\;and\; }}z{_3}{\rm{ }}.\) Therefore,

\[\begin{align}&\qquad\qquad\left| {z - {z_1}} \right| = \left| {z - {z_2}} \right| = \left| {z - {z_3}} \right|\\ &\Rightarrow \,\,\,(z - {z_1})(\bar z - {{\bar z}_1}) = (z - {z_2})(\bar z - {{\bar z}_2}) = (z - {z_3})(z - {{\bar z}_3})\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,&\qquad\qquad\underbrace {\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;}_{{\rm{Equality}}\,{\rm{A}}}\,\,\;\;\;\underbrace {\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;}_{{\rm{Equality B}}}\\ \end{align}\]

\(\Rightarrow \) From the first two terms in the equality above (Equality \(A\)) we get:

\[\begin{gathered} z\, - z{{\bar z}_1} - {z_1}\bar z + {z_1}{{\bar z}_1} = z\, - z{{\bar z}_2} - {z_2}\bar z + {z_2}{{\bar z}_2} \hfill \\\Rightarrow \,\,\,\bar z({z_2} - {z_1}) = z({{\bar z}_1} - {{\bar z}_2}) + {\left| {{z_2}} \right|^2} - {\left| {{z_1}} \right|^2}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,...\left( 1 \right) \hfill \\\end{gathered} \]

Similarly, from equality \(B,\) we get

\[\bar z({z_3} - {z_2}) = z({\bar z_2} - {\bar z_3}) + {\left| {{z_3}} \right|^2} - {\left| {{z_2}} \right|^2}\,\,\,\,\,\,\,\,\,...\left( 2 \right)\]

Dividing (1) by (2), we get :

\[\frac{{{z_2} - {z_1}}}{{{z_3} - {z_2}}} = \frac{{z({{\bar z}_1} - {{\bar z}_2}) + {{\left| {{z_2}} \right|}^2} - {{\left| {{z_1}} \right|}^2}}}{{z({{\bar z}_2} - {{\bar z}_3}) + {{\left| {{z_3}} \right|}^2} - {{\left| {{z_2}} \right|}^2}}}\]

Solving for \(z,\) we get

\[z = \frac{{{{\left| {{z_1}} \right|}^2}({z_2} - {z_3}) + {{\left| {{z_2}} \right|}^2}({z_3} - {z_1}) + {{\left| {{z_3}} \right|}^2}({z_1} - {z_2})}}{{{{\bar z}_1}({z_2} - {z_3}) + {{\bar z}_2}({z_3} - {z_1}) + {{\bar z}_3}({z_1} - {z_2})}}\]

Example - 40

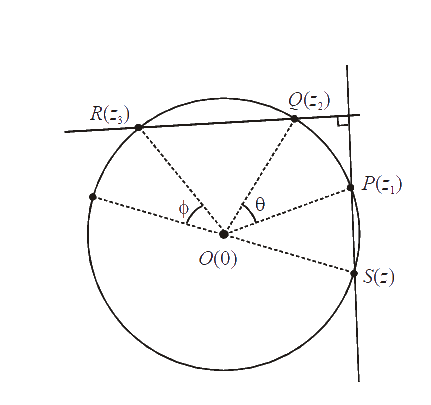
\({z_1},\;{z_2}\;{\rm{and}}\;{z_3}\) are three points on a circle centered at the origin. A point \(z\) is chosen on the circle such that the line joining \(z\) and \({z_1}\) is perpendicular to the line joining \({z_2}\) and \({z_3}\) . Show that \(z{z_1} + {z_2}{z_3} = 0\)

Solution:

We observe \(\theta  = \phi \) that, because of the perpendicularity of the two secants.

Now \(\angle \,SOR + \angle SOQ - \angle SOP = \pi \) , (by using the fact that \(\theta  = \phi \) ). Therefore,

\[\begin{align}& \qquad\;\; \left( {\arg \left( {{z_3}} \right) - \arg (z)} \right) + \left( {\arg \left( {{z_2}} \right) - \arg (z)} \right) - \left( {\arg \left( {{z_1}} \right) - \arg (z)} \right) = \pi \\ &\Rightarrow \quad \arg \left( {{z_3}} \right) + \arg \left( {{z_2}} \right) = \pi + \arg \left( {{z_1}} \right) + \arg \left( z \right)\\ &\Rightarrow \quad \arg \left( {{z_2}{z_3}} \right) = \pi + \arg \left( {z{z_1}} \right)\\ &\Rightarrow \quad {z_2}{z_3} = - z{z_1} \Rightarrow \quad z{z_1} + {z_2}{z_3} = 0\end{align}\]

TRY YOURSELF - IX

Q. 1 Find the real slopes of the following lines

(a) \(iz - i\bar z + 1 = 0\)

(b) \(\omega z + {\omega ^2}\bar z + 1 = 0\)

(c) \({e^{i\frac{\pi }{4}}}z + {e^{ + \frac{\pi }{4}}}\bar z = 0\)

(d) \(3z + 3\bar z + 2 = 0\)

Q. 2 Write the complex forms for the following lines

(a) \(x + y + 1 = 0\)

(b) \(\sqrt 3 x - y + 1 = 0\)

(c) \(x = 1\) (d) y = 1

Q. 3 Consider a point  \(z{_0}\) on the circle \(|z|\; = r\) . What will be the equation of the tangent at \(z{_0}\) ?