Infinite Geometric Series Formula
Before learning the infinite geometric series formula, let us recall what is a geometric series. A geometric series is the sum of a sequence wherein every successive term contains a constant ratio to its preceding term. An Infinite geometric series has an infinite number of terms and can be represented as a, ar, ar2, ..., to ∞. Let us learn the infinite geometric series formula in the upcoming section.
What Is Infinite Geometric Series Formula?
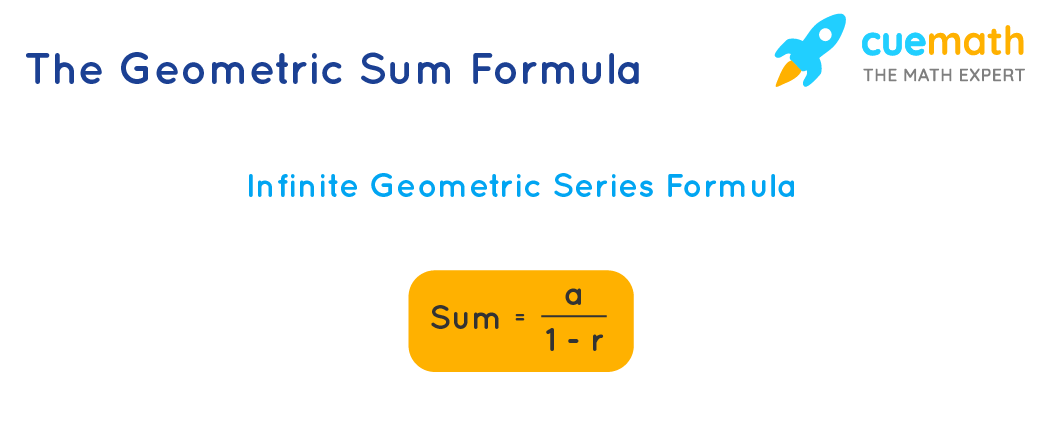
The sum of the infinite geometric series formula of the infinite series formula is also known as the sum of infinite GP. The infinite series formula if the value of r is such that −1<r<1, can be given as,
Sum = a/(1-r)
Where,
- a = first term of the series
- r = common ratio between two consecutive terms and −1 < r < 1
Infinite Geometric Series Formula
The geometric series converges to a sum only if r < 1. If r> 1, the series does not converge and doesn't have a sum. For example 8, 12, 18, 27, .... is the given geometric series.
To find the sum : 8 + 12 + 18 + 27 + ..... , we find that a = 8 and r = 12/8 = 18/ 12 = 3/2
Here r > 1. Thus the sum does not converge and the series has no sum.

Let us now have a look at a few solved examples using the Infinite Series Formula.
Examples using Geometric Infinite Series Formula
Example 1: Find the sum of the terms 1/9 + 1/27 + 1/81 + ... to ∞?
Solution: To find: Sum of the geometric series
Given:
a = 1/9, r = 1/3
Using the infinite geometric series formula,
Sn = a /(1-r)
Sn = (1/9)( 1 - 1/3)
Sn = 1/6
Answer: The sum of the given terms is 1/6
Example 2: Calculate the sum of series 1/5, 1/10, 1/20, .... if the series contains infinite terms.
Solution: To find: Sum of the geometric series
Given:
a = 1/5, r = 1/5
Using the infinite geometric series formula,
Sum = a /(1-r)
= (1/5)( 1 - 1/5)
= 1/4
Answer: The sum of the given terms is 1/4
Example 3: Find the sum of the geometric series 125, 25, 5, 1......∞
Solution: The series is,125+25+5+1+ ........ a =125, and = 25/125 = 1/5
Using the infinite geometric series formula,
Sum = a /(1-r)
= 125/(1-1/5)
= 125/(4/5)
= 625/4
Thus sum to infinity terms is
Answer: Thus sum to infinity in the given series 125+25+5+1+ ........ =& 625/4
FAQs on Geometric Infinite Series Formula
What Is Geometric Infinite Series Formula?
The infinite geometric series formula is used to find the sum of all the terms in the geometric series without actually calculating them individually. The infinite geometric series formula is given as:
\(S_{n}=\dfrac{a}{1-r}\)
Where
- a is the first term
- r is the common ratio
A tangent of a circle in geometry is defined as a straight line that touches the circle at only one point.
Can the Sum of an Infinite Geometric Series be Negative?
The sum of an infinite series implies that the series is geometric and an infinite arithmetic series can never converge. So if the common ratio is positive there can be no negative sum.
What Is the Sum of Infinite GP?
The sum to infinite GP means, the sum of terms in an infinite GP. The infinite geometric series formula is S∞ = a/(1 – r), where a is the first term and r is the common ratio.
What Is a and r in Infinite Series Formula?
In finding the sum of the given infinite geometric series If r<1 is then sum is given as Sum = a/(1-r). In this infinite series formula, a = first term of the series and r = common ratio between two consecutive terms and −1<r<1
visual curriculum
