Sum of Angles in a Polygon
The sum of the angles in a polygon depends on the number of edges and vertices. There are two types of angles in a polygon - the Interior angles and the Exterior Angles. Let us learn about the various methods used to calculate the sum of the interior angles and the sum of exterior angles of a polygon.
Types of Polygons
Polygons are classified into various categories depending upon their properties, the number of sides, and the measure of their angles. Based on the number of sides, polygons can be categorized as:
- Triangle (3 sides)
- Quadrilateral (4 sides)
- Pentagon (5 sides)
- Hexagon (6 sides)
- Heptagon (7 sides)
- Octagon (8 sides)
- Nonagon (9 sides)
- Decagon (10 sides) and so on
Based on the other properties, polygons can be categorized as:
- Regular Polygons and Irregular Polygons
- Concave Polygons and Convex Polygons
- Equilateral Polygons and Equiangular Polygons
Types of Angles in a Regular Polygon
A regular polygon is a polygon in which all the angles and sides are equal. There are 2 types of angles in a regular polygon:
- Interior Angles- The angles that lie inside a shape, generally a polygon, are said to be its interior angles.
- Exterior Angles- An exterior angle of a polygon is the angle between a side and its adjacent extended side.
Sum of Interior Angles in a Polygon
The interior angles of a polygon are those angles that lie inside the polygon. Observe the interior angles A, B, and C in the following triangle. The interior angles in a regular polygon are always equal to each other. Therefore, to find the sum of the interior angles of a polygon, we use the formula: Sum of interior angles = (n − 2) × 180° where 'n' = the number of sides of a polygon.
Another way to calculate the sum of the interior angles is by checking the number of triangles formed inside the polygon with the help of the diagonals. Since the interior angles of a triangle sum up to 180°, the sum of the interior angles of any polygon can be calculated by multiplying 180° with the number of triangles formed inside the polygon. For example, a quadrilateral can be divided into two triangles using the diagonals, therefore, the sum of the interior angles of a quadrilateral is 2 × 180° = 360°. Similarly, a pentagon can be divided into 3 triangles, so, the pentagon's interior angles will sum up to 3 × 180° = 540°
Example:
What is the Sum of the Interior Angles in a Hexagon?
Solution:
A hexagon has 6 sides, therefore, n = 6
The sum of interior angles of a regular polygon, S = (n − 2) × 180
S = (6-2) × 180°
⇒ S = 4 × 180
⇒ S=720°
Therefore, the sum of interior angles of a hexagon is 720°.
Sum of Exterior Angles in a Polygon
An exterior angle (outside angle) of any shape or regular polygon is the angle formed by one side and the extension of the adjacent side of that polygon. Observe the exterior angles shown in the following polygon.
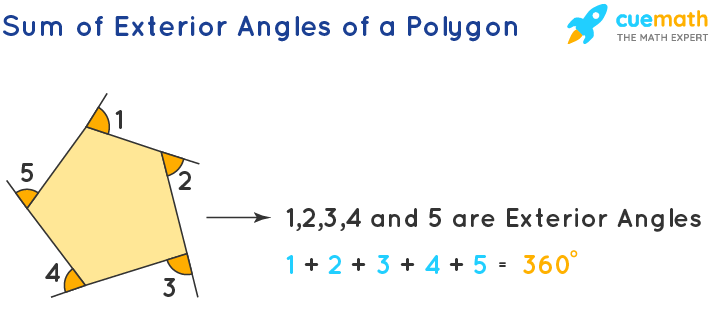
The sum of the exterior angles of a polygon is equal to 360°. This can be proved with the following steps:
- We know that the sum of the interior angles of a regular polygon with 'n' sides = 180 (n-2).
- The interior and exterior angle at each vertex form a linear pair. Therefore, there will be 'n' linear pairs in the polygon. Now, since each linear pair sums upto 180°, the sum of all linear pairs will be: 180n°.
- So, the sum of exterior angles = Sum of all linear pairs - Sum of interior angles
- This means: Sum of exterior angles = 180n – 180(n-2) = 180n – 180n + 360. Hence, the sum of exterior angles of a pentagon equals 360°.
Related Articles:
Check out these interesting articles related to the Sum of Angles in a Polygon. Click to know more!
FAQs on Sum of Angles in a Polygon
What is the sum of all interior angles of a regular polygon?
The sum of all interior angles of a regular polygon is calculated by the formula S=(n-2) × 180°, where 'n' is the number of sides of a polygon. For example, to find the sum of interior angles of a pentagon, we will substitute the value of 'n' in the formula: S=(n-2) × 180°; in this case, n = 5. So, (5-2) × 180° = 3 × 180°= 540°.
What is the sum of all exterior angles of a regular polygon?
The sum of all exterior angles of a regular polygon is 360°.
What is the sum of an interior angle and the exterior angle on the same vertex?
The sum of an interior angle and the exterior angle on the same vertex is always 180° since they form a linear pair.
What is the sum of all interior angles of a quadrilateral?
The formula used to find the sum of the interior angles of a polygon is: S=(n-2) × 180°. In this case, n = 4. Therefore, the sum of the interior angles of a quadrilateral is (4-2) × 180° =360°.
How do you find the measure of an interior angle of a regular polygon?
The measure of an interior angle of a regular polygon is calculated with the help of the formula: 180° × (n-2)/n, where 'n' is the number of sides of a polygon.
How do you find the measure of an exterior angle of a regular polygon?
The measure of an exterior angle of a regular polygon is calculated with the help of the formula: 360°/n where 'n' is the number of sides of a polygon.