Slope Intercept Form

The slope intercept form of a straight line is one of the most common forms used to represent the equation of a line. The slope intercept formula can be used to find the equation of a line when given the slope of the straight line and the y-intercept( the y-coordinate of the point where the line intersects the y-axis). Equation of line is the equation that is satisfied by each point that lies on that line. There are various methods to find this equation of a straight line given as,

• Slope-intercept form
• Point slope form
• Two-point form
• Intercept form

Let us understand slope intercept formula, its derivation using solved examples.

The slope intercept form is a method used to determine the equation of a straight line in the coordinate plane. The equation of a straight line will be that relation which:

• the coordinates of any point on the line must satisfy.
• the coordinates of any point not on the line will not satisfy.

The determination of this equation is straightforward. To find the slope intercept form of a straight line, we would need the slope, or the angle of inclination of this straight line from the x-axis and the intercept that it makes with the y-axis.

Slope Intercept Form Definition

The slope-intercept form of a straight line is used to find the equation of a line. For the slope-intercept formula, we have to know the slope of the line and the intercept cut by the line with the y-axis. Let us consider a straight line of slope 'm' and y-intercept 'b'. The slope intercept form equation for a straight line with a slope, 'm', and 'b' as the y-intercept can be given as: y = mx + b.

Slope Intercept Form Examples

Some examples of the slope intercept form are shown here.

• The equation of a line with slope (-1) and y-intercept (4) is found using: y = -x + 4.
• The equation of a line with slope (2) and passing through origin(y-intercept = 0) is given as: y = 2x.

Note: The slope of the line for which angle of inclination, θ is given can be calculated as tan θ. Also, in the case when we are given two points (x₁, y₁) and (x₂, y₂) lying on the straight line, the slope can be given as: (y₂ - y₁)/(x₂ - x₁). Let us have a look at the slope-intercept formula and its derivation for a better understanding of the concept.

The slope-intercept formula is used to find the slope, the y-intercept, the x-intercept, or the equation of a straight line given the requisite parameters. There are different formulas available to find the equation of a straight line. The slope-intercept formula is one of these formulas which is used when we know the slope of the straight line, which is denoted by m, and the y-intercept of the straight line, which is denoted by b or (0, b). Let us learn the slope-intercept formula with a few solved examples. Here is the slope-intercept formula.

Slope Intercept Formula in Math

Using the slope-intercept formula, the equation of the line is:

y = mx + b

where,

• m = the slope of the line
• b = y-intercept of the line
• (x, y) represent every point on the line
  x and y have to be kept as the variables while applying the above formula.

Note: The slope-intercept formula cannot be applied to find the equation of a vertical line. Here's an example to understand the application of slope intercept formula.

Example : The equation of a line is 3x + 4y + 5 = 0. Determine the slope and y-intercept of the line using the slope intercept form.

Solution: We re-arrange the equation of the line to write it in the standard form y = mx + b.
We have:

4y = -3x - 5
⇒ y = (-3/4)x + (-5/4)

Thus, m = -3/4 , b = -5/4

Answer: The slope of the given straight line, m = -3/4 and the y-intercept, b = -5/4.

Let us consider a line whose slope is 'm' that intersects the y-axis at (0, b), i.e., its y-intercept is b. Also, let us consider an arbitrary point (x, y) on the line.

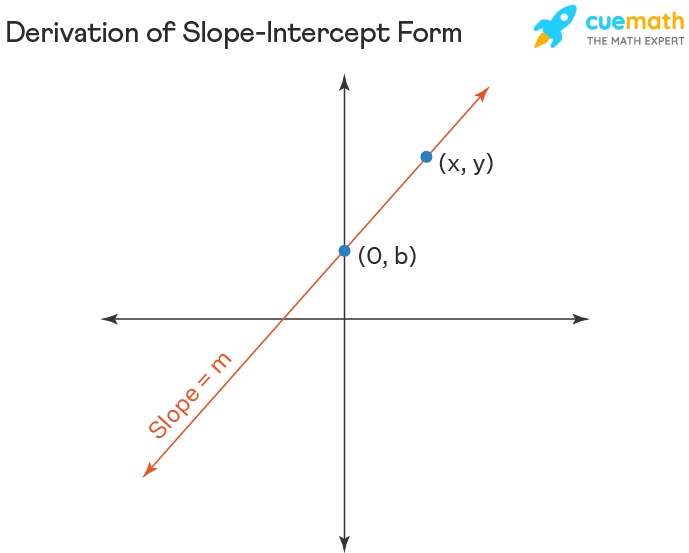
Let us assume that (x₁, y₁) = (0, b) and (x₂, y₂) = (x, y).

Using the slope formula, the slope of a line joining two points (x₁, y₁) and (x₂, y₂) is, m = (y₂ - y₁)/(x₂ - x₁)

Using this formula, the slope of the above line is,
m = (y - b) / (x - 0)
⇒ m = (y - b) / (x)

Multiplying both sides by x,
mx = y - b

Adding 'b' on both sides,
y = mx + b

This is the general equation of a straight line involving its slope and its y-intercept. This form of the equation of the line is therefore termed the slope-intercept form. Hence, the slope intercept formula is derived.

To find the equation of a line with an arbitrary inclination, we would need two quantities: the inclination of the line (or its slope or the angle, θ, it makes with say, the x-axis) and the placement of the line (i.e. where the line passes through with reference to the axes; we can specify the placement of the line by specifying the point on the y-axis through which the line passes, or in other words, by specifying the y-intercept, b). Any line can be determined uniquely using these two parameters.

The steps to find the equation of a line using the slope-intercept form are given below,

Step 1: Note down the y-intercept, 'b', and the slope of the line as 'm'. We can apply the slope formula to find the slope of any straight line, in case it is not given directly and other relevant data is provided.
Step 2: Apply the slope intercept formula: y = mx + b.

Example: A line is inclined at an angle of 60° to the horizontal, and passes through the point (0, - 1). Find the equation of the line.

Solution: We have, m = tan 60º = √3

Thus, the equation of the line is, y = mx + c
⇒y = (√3)x + (−1)
⇒y = √3x − 1

We can convert the equation of a line given in the standard form to slope intercept form by rearranging and comparing. We know that the standard form of the equation of a straight line can be given as, Ax + By + C = 0. Rearranging the terms to find the value of 'y', we get,
B × y = -Ax - C
⇒y = (-A/B)x + (-C/B),
where, (-A/B) makes the slope of the line and (-C/B) is the y-intercept.

Topics Related to Slope Intercept Form:

• Euclidean Distance Formula
• Geometry
• x and y axis

Important Notes on Slope Intercept Form:

• A line may have a negative slope in case the angle it makes with the positive x-direction is an obtuse angle. The value of tan θ, in this case, will be negative, so m will be negative.
• For any line passing through the origin, the y-intercept will be (b = 0), so its equation will be of the form: y = mx.

What is Slope Intercept Form in Math?

The slope intercept form in math is one of the forms used to calculate the equation of a straight line, given the slope of the line and intercept it forms with the y-axis. The slope intercept form is given as, y = mx + b, where 'm' is the slope of the straight line and 'b' is the y-intercept.

What is the Slope Intercept Form Equation?

The slope intercept equation is used to find the general equation of a straight line using its slope and the point where it intersects the y-axis. Slope intercept form equation is given as, y = mx + b.

How do you Find Slope-Intercept Form?

The slope intercept form of any line can be calculated simply using the slope and y-intercept. The slope intercept form of a straight line is given as,
y = mx + b
where,

• (x, y) is an arbitrary point on the line
• m is the slope of the line
• b is the y-intercept

How to Find the Equation of a Straight Line Using Slope Intercept Form?

We need the slope of the straight line and its point of intersection with the y-axis to find the straight-line equation using the slope intercept form. The slope of a line can be calculated using the slope formula. Using the slope intercept form, equation of straight line can be calculated as, y = mx + b, where 'm' is the slope of the straight line and 'b' is the y-intercept.

What is Slope-Intercept Formula?

The slope-intercept formula is one of the formulas used to find the equation of a line. The slope-intercept formula of a line with slope m and y-intercept b is, y = mx + b. Here (x, y) is any point on the line.

How To Derive the Slope-Intercept Formula?

Let us consider a line whose slope is m and whose y-intercept is (0, b). To find the equation of the line, consider a random point (x, y) on it. Then using the slope formula, (y - b) / (x - 0) = m. Solving it for y, we get y = mx + b.

What are the Applications of the Slope-Intercept Formula?

The slope-intercept formula is used to

• find the equation of a line.
• graph a line using the y-intercept and slope.
• find the slope of a line easily.
• find the intercepts of a line easily.

How to Find the Slope of a Line Using the Slope-Intercept Form?

We can find the slope of a line using the slope-intercept form given as, y = mx + b, where 'm' is the slope of the line and 'b' is the y-intercept. Here is an example. Let us find the slope of the line 6x - 3y = 5. Let us solve this for 'y' to get into the slope-intercept form. Then we get y = 2x - (5/3). Comparing this with the slope-intercept formula, y = mx + b, we get its slope to be m = 2.

How to Convert Standard Form of Straight Line Equation to Slope Intercept Form?

The standard form of equation of a straight line is given as, Ax + By + C = 0. Rearranging this standard form, we can find the slope intercept of any straight line given in this form as, y = (-A/B)x + (-C/B), where (-A/B) makes the slope of the line and (-C/B) is the y-intercept.