Segment Addition Postulate
The segment addition postulate in geometry is applicable on a line segment containing three collinear points. It states that if there are two given points on a line segment A and C, then point B lies on the same line segment somewhere between A and C only if the sum of AB and BC is equal to AC.
By applying the segment addition postulate, we can precisely determine the length of a line segment when given specific measurements of its parts. Also, this postulate enables us to divide a line segment into different sections and explore the relation (ratios) between their lengths.
| 1. | Segment Addition Postulate Definition |
| 2. | Segment Addition Postulate Formula |
| 3. | FAQs on Segment Addition Postulate |
Segment Addition Postulate Definition
The segment addition postulate states that if a line segment has two endpoints, A and C, a third point B lies somewhere on the line segment AC if and only if the equation AB + BC = AC is satisfied. Look at the image given below to have a better understanding of this postulate.
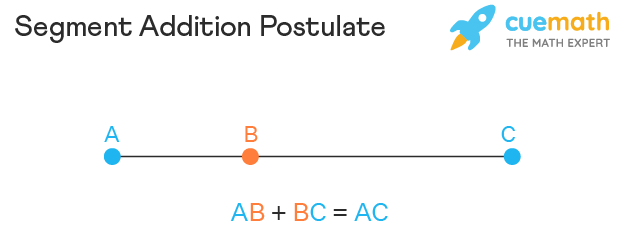
If we carefully look at its name "Segment Addition Postulate", it is very easy to understand.
- A segment, here, means a line segment. It emphasis that this postulate is applicable only on a line segment, and not on a ray or a line. A line segment is part of a line bounded by two defined endpoints. We can have an infinite number of points between the endpoints of a segment.
- The "addition" means that we are adding the distances between points.
- Finally, "postulate" means this axiom is taken as a fact or valid without any proof.
Another way of stating the segment addition postulate is that if point B lies on the line segment AC, then AB + BC = AC.
Segment Addition Postulate Formula
If the end-points of a line segment are denoted as A and C, and there lies a point B on the line segment, then the segment addition postulate formula is given as AB + BC = AC.
Further, extending this theorem to two points, If there are two points B and D on the segment, we will have the formula as AB + BD + DC = AC.
☛ Related Topics:
Segment Addition Postulate Examples
-
Example 1: In the given figure, if B is the mid-point of line segment AC, find the length of segment AC.
Solution:
By using the segment addition postulate, we know that the sum of segments AB and BC is equal to AC. It can be written mathematically as AB + BC = AC. Also, B is the midpoint of AC. It implies AB = BC.
AB = BC
⇒ 3x = 4x-6
⇒ 6 = 4x - 3x
⇒ x = 6
Now, put the value of x in the equation AB + BC = AC.
AC = 3x + 4x - 6
⇒ AC = 7x - 6
⇒ AC = 7 × 6 - 6
⇒ AC = 42 - 6
⇒ AC = 36
Answer: ∴ The length of the segment AC is 36 units.
-
Example 2: Find whether Q is the mid-point of segment PR or not, if the length of PR is 45 units. [Refer to the figure below]
Solution: There are three collinear points on the given segment which are points P, Q, and R. By using the segment addition postulate, we know that PQ + QR = PR. Substitute the value of PR as 45 units, we get,
PQ + QR = 45
⇒ 9x + 7 + (-3x+20) = 45
⇒ 9x - 3x + 7 + 20 = 45
⇒ 6x + 27 = 45
⇒ 6x = 18
⇒ x = 3
Now, let us find the values of PQ and QR.
PQ = 9x + 7 = 9 (3) + 7 = 34 units
QR = -3x+20 = -3 (3) + 20 = 11 units
Answer: ∴ PQ ≠ QR. Q is not the midpoint of segment PR.
FAQs on Segment Addition Postulate
What is Segment Addition Postulate in Geometry?
The segment addition postulate in geometry is the axiom which states that the length of a line segment divided into smaller pieces is the sum of the lengths of all those smaller segments. So, if we have three collinear points A, B, and C on segment AC such that B is somewhere between A and C, then AB + BC = AC. It is a mathematical fact that can be accepted without proof.
What are the Two Conditions of the Segment Addition Postulate?
The two conditions of the segment addition postulate are given below:
- A point P lies on a segment MN if and only if points M, P, and N are collinear taken in order.
- The distance between MP and PN must be equal to MN.
What are the Examples of Segment Addition Postulate?
As per the segment addition postulate, if we have an iron rod of length 30 inches that is cut into two parts where the length of one part is 14 inches, it means the length of the other part of the rod is 30 - 14 = 16 inches.
What is a Segment Addition Postulate Used For?
We can apply this postulate in calculating the missing lengths. It can be used to find the sum of the smaller parts of a segment to find the total length. The segment addition postulate has its applications in construction, architecture, design, etc.
How to Solve for x with Segment Addition Postulate?
If we have a missing length, let's say x, and we know the total length and the length of the other part of the segment, then we can apply the segment addition postulate to find x. For example, if AB = 3, BC = x, and AC = 5, then we can find x by subtracting AB from AC. This implies AC - AB = 5 - 3 = 2 = BC. i.e., x = 2.
How to Use the Segment Addition Postulate to Show that ae=ab+bc+cd+de?
If a segment AE has three points on it, marked as B, C, and D in order, then according to the segment addition postulate, their sum is equal. So, AE = AB + BC + CD + DE. This is possible by applying the postulate for more than one time.
What is Segment Addition Postulate in Proofs?
The segment addition postulate does not require any proof. It is accepted as a mathematical fact. But many times, we use this axiom in stating proofs for line segments. One such proof is given as "If two congruent segments are added to the line segments of the same length, then their sum is also equal."