SAS- Side Angle Side Congruence and Similarity
The word "congruent" for figures means equal in every aspect, majorly in terms of shape and size. The relation of two congruent figures is described by congruence. Congruence is the term used to describe the relation of two figures that are congruent. Now let's discuss the SAS congruence of triangles. In order to be sure that the two triangles are similar, we do not necessarily need to inquire about all sides and all angles. Congruence of triangles means:
- All corresponding angle pairs are equal.
- All corresponding sides are equal.
In this mini-lesson, we will learn about the SAS similarity theorem in the concept of the SAS rule of congruence, using similar illustrative examples.
| 1. | What do you mean by Side Angle Side? |
| 2. | The SAS Congruence Rule |
| 3. | The SAS Similarity Rule |
| 4. | How do you Solve a SAS Triangle? |
| 5. | FAQs on SAS |
What do you mean by Side Angle Side?
SAS congruence is the term which is also known as Side Angle Side congruence, which is used to describe the relation of two figures that are congruent. Let's discuss the SAS congruence of triangles in detail to understand the meaning of SAS. Look at ΔABC and ΔPQR:

These two triangles are of the same size and shape. Thus, we can say that these are congruent. They can be considered as congruent triangle examples. We can represent this in a mathematical form using the congruent triangles symbol (≅). (ΔDEF≅ΔPQR). This means D falls on P, E falls on Q, and F falls on R. ED falls on PQ, EF falls on QR, and DF falls on PR. Thus, we can conclude that the corresponding parts of the congruent triangles are equal.
SAS Criterion
Under this criterion, if the two sides of a triangle are equal to the two sides of another triangle, and the angle formed by these sides in the two triangles are equal, then these two triangles are congruent. The SAS Criterion stands for the 'Side-Angle-Side' triangle congruence theorem.

The SAS Congruence Rule
The Side-Angle-Side theorem of congruency states that, if two sides and the angle formed by these two sides are equal to two sides and the included angle of another triangle, then these triangles are said to be congruent.
Verification:
Let's perform an activity to show the proof of SAS. Given: AB=PQ, BC=QR, and ∠B=∠Q. To prove: ΔABC ≅ ΔPQR
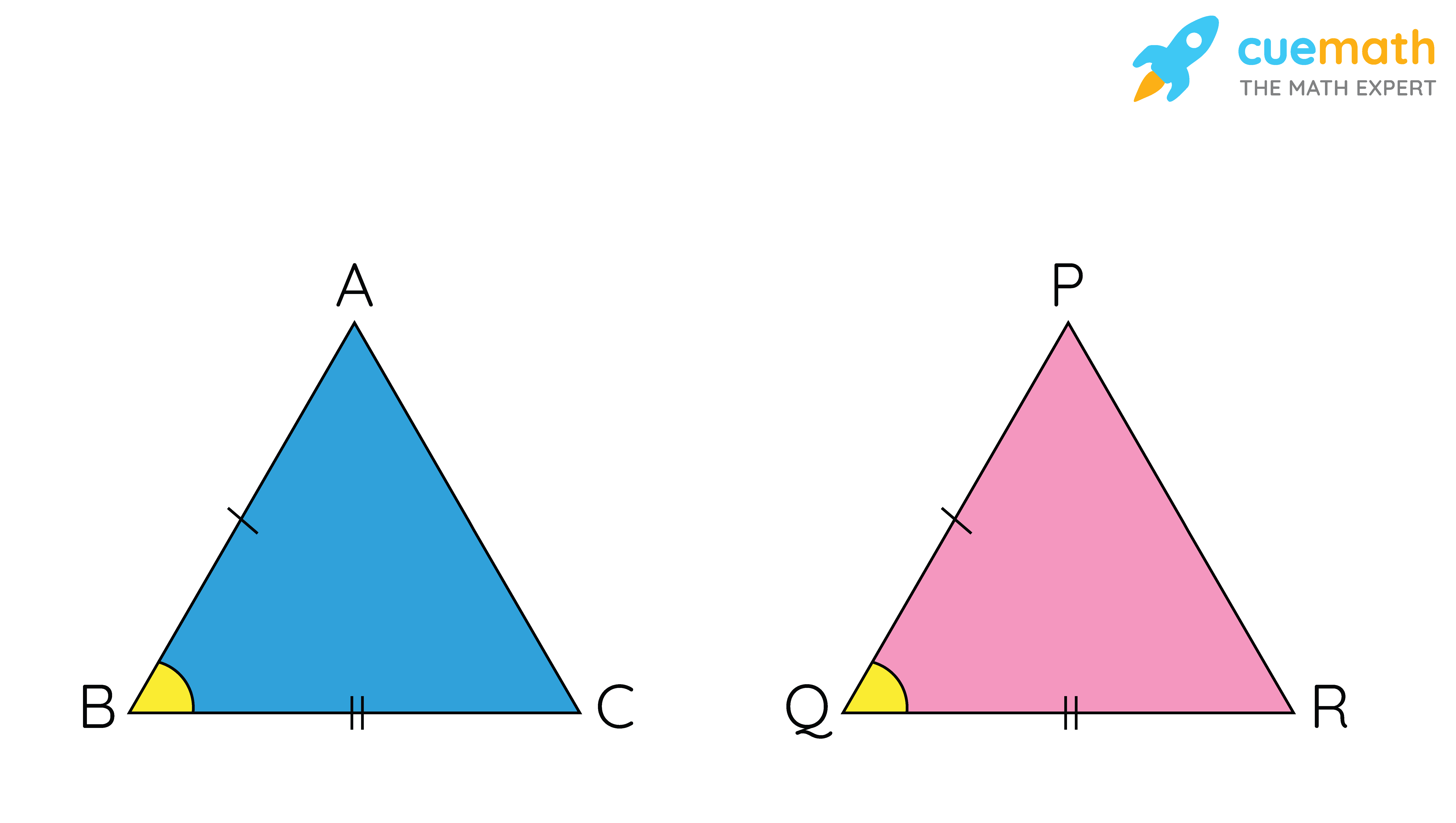
Place the triangle ΔABC over the triangle ΔPQR such that B falls on Q and side AB falls along the side PQ.
- Since AB=PQ, so point A falls on point P.
- Since ∠B=∠Q, so the side BC will fall along the side QR.
- BC=QR, so point C falls on point R. Thus, BC coincides with QR and AC coincides with PR.
So, ΔABC will coincide with ΔPQR. Therefore, ΔABC≅ΔPQR. This demonstrates SAS criterion of congruence.
The SAS Similarity Rule
The SAS similarity criterion states that If two sides of one triangle are respectively proportional to two corresponding sides of another, and if the included angles are equal, then the two triangles are similar.

Given: DE/AB=DF/AC and ∠D=∠A. To prove: ΔDEF is similar to ΔABC
The SAS criterion tells us that ΔABC ~ ΔDEF. Let us see the justification of this.
Construction:
- Take a point X on AB such that AX = DE.
- Through X, draw segment XY∥ BC, intersecting AC at Y.

Proof:
Since XY II BC, we can note that ΔAXY ~ ΔABC, and thus: AX/AB = AY/AC....(1)
Now, we will show that ΔAXY and ΔDEF are congruent. It is given that DE/AB=DF/AC....(2)
Since AX=DE (By construction) and from (1) and (2), we have: DE/AB = AX/AB = AY/AC = DF/AC. Thus, AY=DF
Now, by the SAS congruency criterion, ΔAXY≅ΔDEF⇒ΔAXY∼ΔDEF
While we already have, ΔAXY ~ ΔABC. This means ΔDEF and ΔABC are similar. Hence Proved.
How do you Solve a SAS Triangle?
Observe the triangle ABC where

AB= 2 units, BC= 4 units and ∠ABC=50°
Now, to find the value of side AC, we will use the law of cosine.
The low of cosine gives the formula b2 = a2 + c2 − 2ac cos B, where AB = c; BC = a; and AC = b.
= b2 = a2 + c2 − 2ac cosB
= x2 = 42 + 22 − 2 (2) (4) cos 50°
= 16 + 4 − 16 (0.643)
= 20 − 10.288
= x = √9.712 = 3.116
Now apply the sine rule in the triangle ABC and calculate the value of ∠C.
sin C/2 = sin B/x
sin C/2 = sin 50°/3.116
sin C = 2 × 0.766/3.116 = 0.492
∠C = sin−1 0.492 = 29.47°
Then to calculate the value of ∠A use the sum of interior angles of a triangle is 180°
∠A + ∠B + ∠C = 180°
∠A + 50° + 29.47° = 180°
∠A = 180° − 79.47°
=100.53°
Importat Notes:
- Two triangles are said to be congruent if their shape and size are exactly the same.
- We represent the congruence of triangles using the symbol (≅).
- If the two sides and the angle formed at their vertex of one triangle are equal to the two corresponding sides and the angle formed at their vertex of another triangle then the triangles are congruent by SAS Criterion for Congruence.
Related Articles on SAS-Side Angle Side Congruence and Similarity
Examples on SAS
-
Example.1: James wanted to know which congruency rule says that these triangles are congruent. Let's help him.

Solution:
Here, EF = MO = 3in, FG = NO = 4.5in, ∠EFG = ∠MON = 110°. Thus, △EFG ≅ △MNO ( By SAS rule ). ∴ These triangles are congruent by the SAS rule.
-
Example 2: Triangle ABC is an isosceles triangle and the line segment AD is the angle bisector of the angle A. Can you prove that ΔADB is congruent to the ΔADC by using SAS rule?

Solution:
The triangle, ABC is an isosceles triangle where it is given that AB=AC. Now the side AD is common in both the triangles ΔADB and ΔADC. As the line segment AD is the angle bisector of the angle A then it divides the ∠A into two equal parts. Therefore, ∠BAD=∠CAD. Now according to the SAS rule, the two triangles are congruent. Hence, ΔADB≅ΔADC.
-
Example 3: Jolly was doing geometrical construction assignments in her notebook. She drew an isosceles triangle PQR on a page. She marked L, M as the midpoints of the equal sides (PQ and QR) of the triangle and N as the midpoint of the third side. She states that LN=MN. Is she right?

Solution:We will prove that ΔLPN ≅ ΔMRN. We know that ΔPQR is an isosceles triangle and PQ=QR. Angles opposite to equal sides are equal. Thus, ∠QPR=∠QRP. Since L and M are the midpoints of PQ and QR respectively, PL = LQ = QM = MR = QR/2. N is the midpoint of PR, hence, PN = NR. In ΔLPN and ΔMRN:
- LP = MR
- ∠LPN = ∠MRN
- PN = NR
Thus, by SAS Criterion of Congruence, ΔLPN ≅ ΔMRN. Since congruent parts of congruent triangles are equal, LN=MN.

FAQs on SAS
How do you Prove the SAS Congruence Rule?
If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the two triangles are said to be congruent by the SAS congruence rule.
What is a SAS Triangle?
A triangle whose two sides and the angle formed by them is known as a SAS triangle.
What is the SAS Axiom?
SAS axiom is the rule which says that if the two sides of a triangle are equal to the two sides of another triangle, and the angle formed by these sides in the two triangles are equal, then these two triangles are congruent by the SAS criterion.
How do you Prove the SAS Similarity Theorem?
SAS similarly can be proved by showing that one pair of side lengths of one triangle is proportional to one pair of side lengths of the other triangle and included angles are equal.
What is the Difference Between SAS and SSS?
Both SAS and SSS rules are the triangle congruence rules. The full form of SAS is "Side-Angle-Side" and SSS stands for "Side-Side-Side."
- In the SAS postulate, two sides and the angle between them in a triangle are equal to the corresponding two sides and the angle between them in another triangle.
- In the SSS postulate, all three sides of one triangle are equal to the three corresponding sides of another triangle.
What are the Four Triangle Similarity Theorems?
The Four triangle similarity theorems are:
- Angle-Angle (AA)
- Side-Angle-Side (SAS)
- Side-Side-Side (SSS)
- Right-Hand-Side (RHS)
What does SAS Mean in Math?
SAS stands for the Side-Angle-Side theorem in the congruency of triangles. The SAS congruency is used when two triangles have one angle common and two sides equal, so as to prove that such triangles are congruent.
What is SAS and ASA Congruence Rule?
The SAS congruence rule states that if two sides of a triangle along with an angle in between is equal to two sides and included the angle of another triangle, then the two triangles are said to be congruent. Whereas the ASA congruence rule states that when two angles with an included side are equal to the two angles along with the included side of another triangle, then these two triangles are said to be congruent.
What is SAS, SSS, ASA, and AAS?
The four congruence rules of a triangle are:
- Angle-Angle-Side (AAS): When two angles of a triangle along with a side i.e excluded are equal to two angles and a side of another triangle, the triangles are considered to be congruent.
- Side-Angle-Side (SAS): When two sides with an angle included are equal to two sides with an angle of another triangle, the two triangles are considered to be congruent.
- Side-Side-Side (SSS): When all three sides of a triangle are equal to all three sides of another triangle, the two triangles are considered to be congruent.
- Angle-Side-Angle (ASA): When two angles including the side are equal to two angles with a side of another triangle, the two triangles are said to be congruent.
visual curriculum
