Right Rectangular Prism
A right rectangular prism is a three-dimensional solid shape with 6 faces, 12 edges, and 8 vertices. It is also called a cuboid. The six faces of a right rectangular prism are rectangular in shape. Some examples of a right rectangular prism are books, aquarium, bricks. Similar to other two-dimensional and three-dimensional shapes, the right rectangular prism also has a surface area.
| 1. | Right Rectangular Prism Definition |
| 2. | Formulas of a Right Rectangular Prism |
| 3. | Rectangular Prism Net |
| 4. | FAQs on Right Rectangular Prism |
Right Rectangular Prism Definition
A right rectangular prism is a three-dimensional shape with six faces (with all the 6 faces being rectangular in shape), 12 edges and 8 vertices. All the faces of the prism are rectangles. All the angles formed at the vertices are of 90° or right angles.
Properties of a Right Rectangular Prism
It is very easy to identify a right rectangular prism if we know its basic properties. Listed below are its properties.
- The angles between the base and the sides are right angles.
- All its faces are rectangles.
- Each corner of the prism represents a right angle.
- Each base and top of the prism are congruent.
Formulas of a Right Rectangular Prism
To find the surface area, volume, and length of the diagonal of a right rectangular prism, it is easy if we apply some formulae to make our calculations easier. Let us learn about each of the formulas related to the right rectangular prism in this section.
Surface Area of a Right Rectangular Prism
Surface area is the space occupied by the outer surface of any solid shape. The surface area of a right rectangular prism is the space occupied by all the faces of the right rectangular prism.
Surface area of a right rectangular prism = lw+lw+wh+wh+lh+lh, which is equal to 2(lw+wh+lh) square units.
Surface Area = 2(lw+wh+lh) square units.
Volume of Right Rectangular Prism
Volume is the space occupied by a closed surface of a solid shape. Volume of a right rectangular prism can be defined as the product of the area of one face multiplied by its height.
The volume of a right rectangular prism (V) for a length (l), height (h), and width (w) is given by,
Volume = (l × w × h) cubic units.
Diagonal of a Right Rectangular Prism
A diagonal is a line that joins two opposite corners of a shape that has straight sides. The diagonal of a right rectangular prism is the square root of the sum of the squares of the length, width, and height.
The diagonal of a right rectangular prism of length (l), width (w), and height (h) is given by,
Diagonal = \(\sqrt{l^{2}+w^{2}+h^{2}}\)
Important Notes
- A rectangular prism has six faces - the base, the top, and the four sides.
- The base and top always have the same area. The pairs of opposite sides have the same area as well.
- The volume of Rectangular Prism: V = lwh
- Surface Area of Rectangular Prism: S = 2(lw + lh + wh)
- In a right rectangular prism, edges = 12, faces = 6, vertices = 8
- When all sides of a right rectangular prism are equal, it is called a cube. Its surface area is 6a2 and volume is a3.
Challenging Questions
- Is a cube a rectangular prism?
- Can you determine the volume of a rectangular prism when the area of its base and height are given but the length and width are not given as separate measurements?
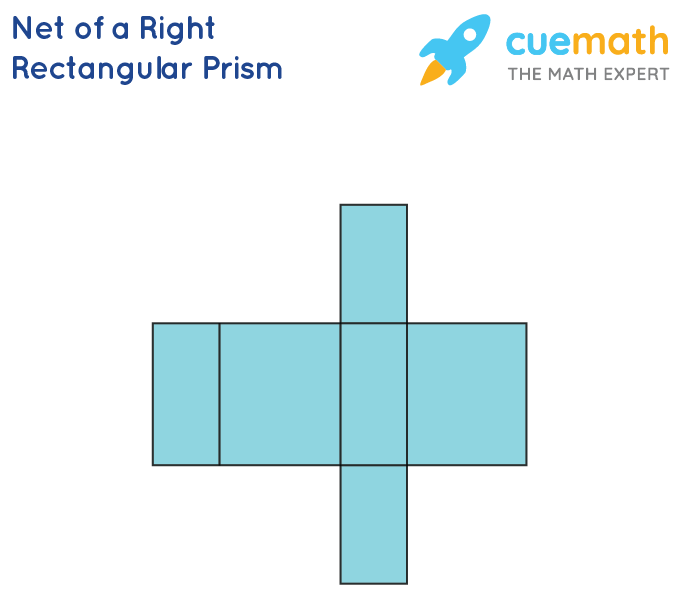
Rectangular Prism Net
A net is defined as a model of a two-dimensional shape that can be folded and made into a three-dimensional shape. The net of any geometrical three-dimensional shape is obtained by unfolding it along its edges and faces. The net of the right rectangular prism is equal to its surface area. The image given below shows the net of a rectangular prism. We can clearly observe that it is made up of rectangles. By calculating the area of each rectangle, and adding them up we can find the net or the surface area of the right rectangular prism.
Topics Related to Right Rectangular Prism
Check out these interesting articles to know more about right rectangular prism.
Solved Examples
-
Example 2:
Identify the right rectangular prisms from the following.
Solution: For an object to be a right rectangular prism, it should have all six faces as rectangles, the opposite faces should be equal and the cross-section along the length is the same. Since all the above conditions are satisfied, we can say that all three are similar to a right rectangular prism. -
Example 4: Tim wanted to add soil to his gardening bed which is in the shape of a rectangular prism and has the following dimensions: length = 8 units, width = 4 units, and height = 1 units. What is the maximum amount of planting soil that can be used to fill the gardening bed?
Solution:Tim's gardening bed resembles that of a rectangular prism having the following dimensions: Length, l = 8 units Width, w = 4 units and Height, h = 1 unit.
The maximum amount of planting soil that can be used to fill the bed is the volume of the bed which is equal to = (l × w × h) cubic units. That is, 8 x 4 x 1 = 32 cubic units. Therefore, 32 cubic units of the soil can be used to fill the gardening bed.
FAQs on Right Rectangular Prism
What is a Right Rectangular Prism?
A right rectangular prism is a three-dimensional object that has 6 faces, 12 edges, and 8 vertices. It is also known as a cuboid.
What is the Formula for Finding the Volume of a Right Rectangular Prism?
The formula to find the volume of a right rectangular prism is given as, Volume of a right rectangular prism = (length × width × height) cubic units.
How to Find Surface Area of Right Rectangular Prism?
To find the surface area of a right rectangular prism, we add the areas of every face. The formula to find the surface area is 2(lw + wh + lh) square units.
What are Some Real-Life Examples of Right Rectangular Prism?
Books, bricks, laptops. aquarium are some real-life examples of a right rectangular prism.
Which Two Dimensional Shape Does Each Face of a Right Rectangular Prism Resemble?
All the faces of a right rectangular prism are rectangular in shape.