Alternate Interior Angles
Alternate interior angles are the angles formed on the opposite sides of the transversal. In other words, when two parallel lines are intersected by a transversal, eight angles are formed. Among these, the angles that lie on the inner side of the parallel lines but on the opposite sides of the transversal are known as the alternate interior angles.
| 1. | What are Alternate Interior Angles? |
| 2. | Converse of the Alternate Interior Angles Theorem |
| 3. | How to Find the Alternate Interior Angles? |
| 4. | FAQs on Alternate Interior Angles |
What are Alternate Interior Angles?
When two parallel lines are crossed by a transversal, the pair of angles formed on the inner side of the parallel lines, but on the opposite sides of the transversal are called alternate interior angles. These angles are always equal. This can also be understood in another way. The alternate interior angles can prove whether the given lines are parallel or not. If these angles are equal, then the given lines which are crossed by a transversal are said to be parallel.
Observe the following figure to see the alternate interior angles. Here AB and CD are two parallel lines crossed by a transversal.
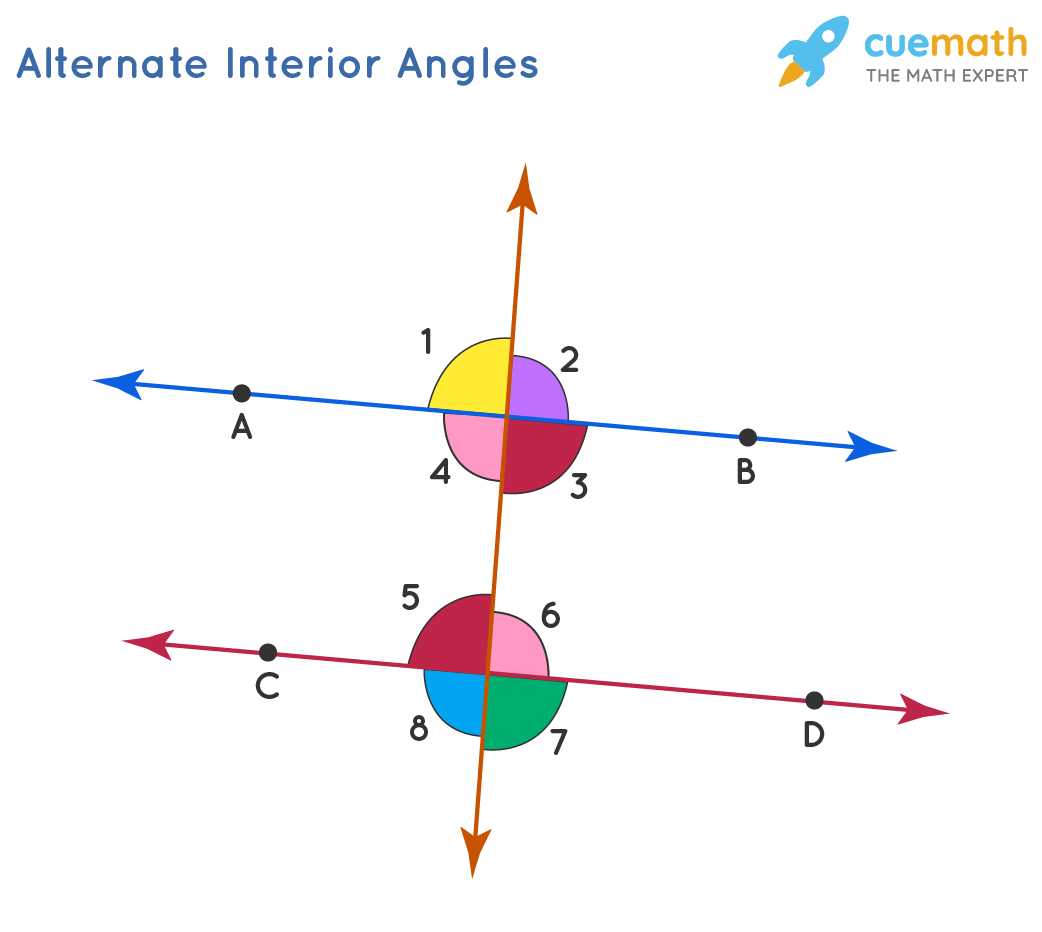
By the alternate interior angles theorem, the pairs of alternate interior angles in the above figure are:
- ∠4 and ∠6
- ∠3 and ∠5
Let us briefly discuss what are alternate exterior angles and how they are different from alternate interior angles.
Alternate Exterior Angles
Alternate exterior angles are those angles that have different vertices, lie on the alternate sides of the transversal, and are exterior to the lines. When a transversal intersects two parallel lines, the alternate exterior angles formed are always equal. In the same figure, ∠1 & ∠7 and ∠2 & ∠8 are the pairs of alternate exterior angles.
Converse of the Alternate Interior Angles Theorem
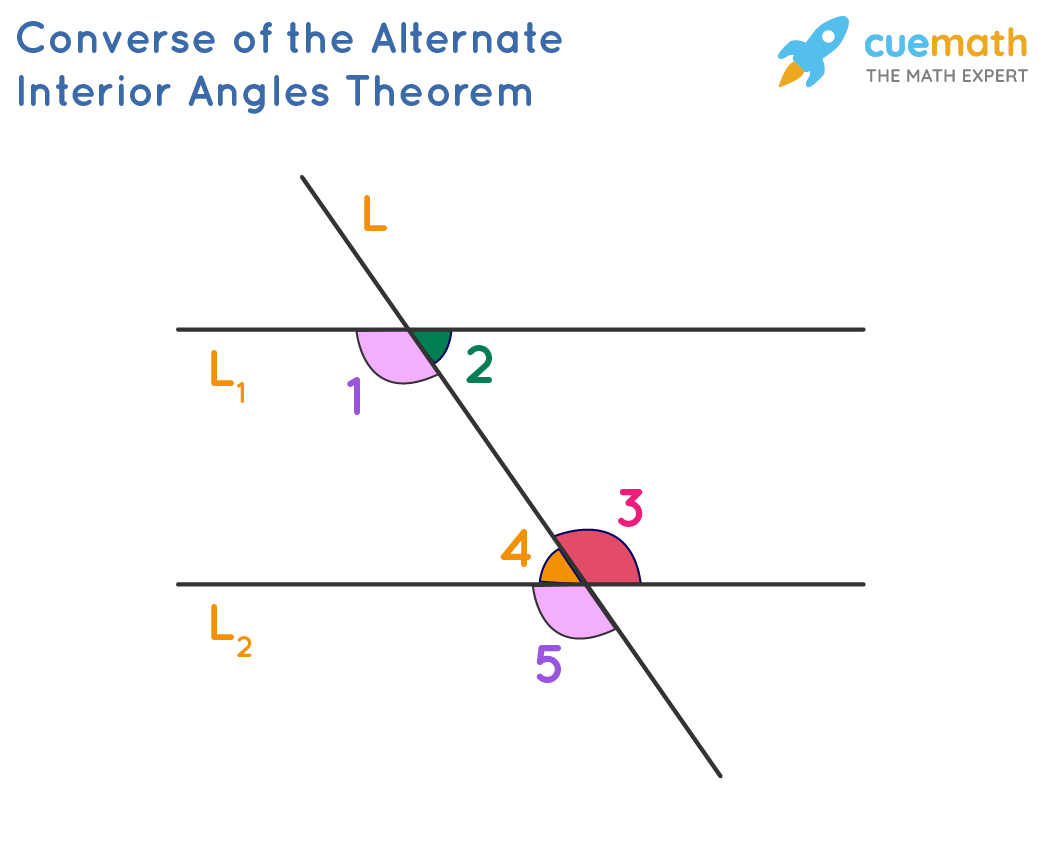
According to the converse of the alternate interior angles theorem, if a transversal intersects two lines such that the alternate interior angles are equal, then the two lines are said to be parallel.
Let us understand this with the help of the following figure which shows: ∠1 = ∠5 (corresponding angles), ∠3 = ∠5 (vertically opposite angles). Thus, ∠1 = ∠3. Similarly, we can prove that ∠2 = ∠4. This proves that since the alternate interior angles in the two given lines are equal, these lines are parallel to each other.
How to Find the Alternate Interior Angles?
According to the alternate interior angles theorem, the alternate interior angles of two parallel lines are equal. We use this fact to find alternate interior angles. Let us understand this with an example.
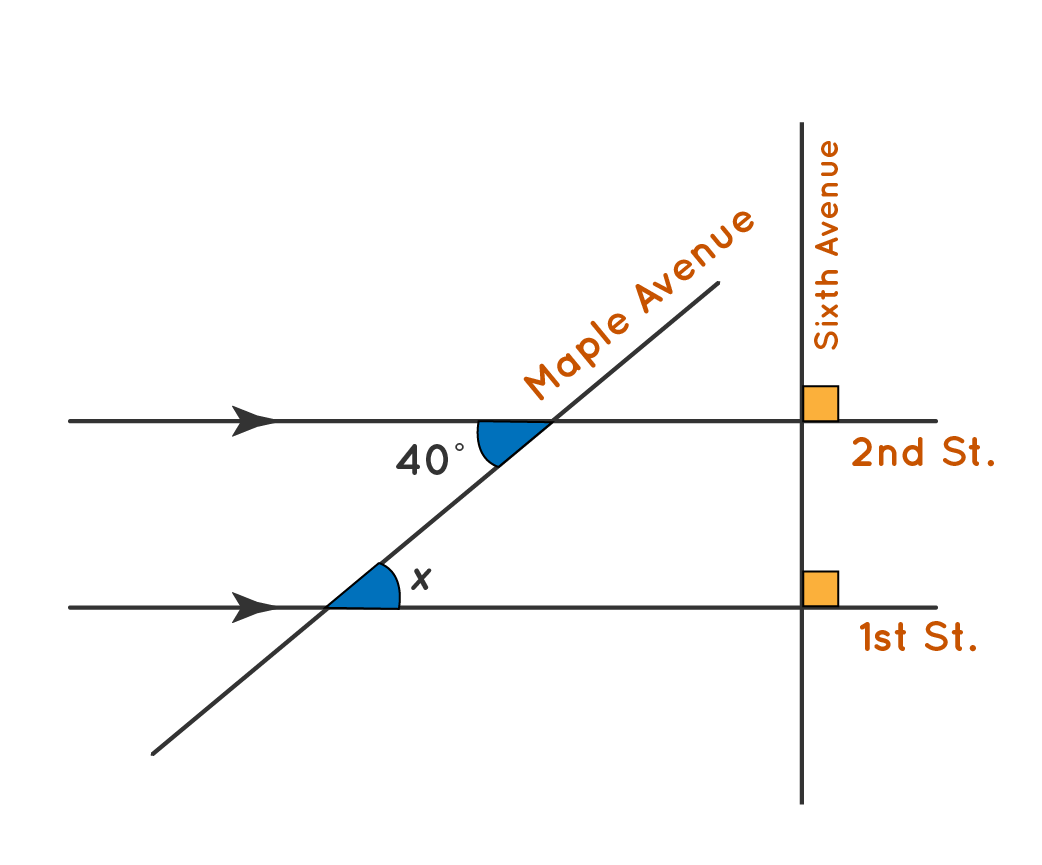
Example: The following figure shows a map in which the road named Sixth Avenue runs perpendicular to the1st Street and the 2nd Street, which are parallel. Another road named Maple Avenue makes an angle of 40° with the 2nd Street. Can you find the measure of angle x?
Solution:
Following the alternate interior angles theorem, if the two streets are parallel, and Maple Avenue is considered to be the transversal, then x and 40° are the alternate interior angles. Hence, both the angles are equal. Therefore, x = 40°.
Important Notes
- Each pair of alternate interior angles is equal.
- Each pair of co-interior angles is supplementary.
- Each pair of corresponding angles is equal.
- Each pair of alternate exterior angles is equal.
Challenging Question
In the following figure, \(\mathrm{AB}\|\mathrm{CD}\| \mathrm{EF}\)

Find the value of x.
☛Topics Related to Alternate Interior Angles
Check out the listed below interesting articles to learn more about alternate interior angles and the related topics.
Alternate Interior Angles Examples
-
Example 1: Find the measure of angle x in the following figure if the two lines are parallel and they are crossed by a transversal.

Solution:
By the alternate interior angles theorem, x and 20° are the alternate interior angles. Hence, they are equal. Therefore, x = 20°.
-
Example 2: In the following figure given to Mathew, MN || OP and ON || PQ. If ∠MNO = 55° then help Mathew in finding ∠OPQ.

Solution:
We will extend the lines in the figure to solve this.

Here, MN || OP and ON is a transversal. Thus, 55° and x are co-interior angles, hence, they are supplementary, i.e., 55° + x° = 180°, x = 125°. Again, ON || PQ and OP is a transversal. Thus, x and ∠OPQ are corresponding angles, hence, they are equal, i.e., ∠OPQ = x = 125°. Therefore, ∠OPQ = 125°.
-
Example 3: In the following figure, l || m and s || t. Find the value of x + y - z.

Solution:
If l || m and t is the transversal, y° and 70° are alternate interior angles. Hence, they are equal in measure (by the alternate interior angle theorem), i.e., y° = 70°. Again, s || t and m is a transversal, x°, and 70° are corresponding angles hence, they are equal, i.e., x° = 70°. Now, let us assume that the angle that is adjacent to x° is w°.

Since x° and w° form a linear pair, x° + w° = 180°
70° + w° =180°
w° = 110°
Now, w° and z° are corresponding angles, hence, they are equal, i.e., z° = w° =110°.
Now, let us substitute the values of the angles: x + y - z = 70° + 70° - 110° = 30°.
Therefore, x + y - z = 30°

FAQs on Alternate Interior Angles
What Are Alternate Interior Angles in Geometry?
In geometry, to define alternate interior angles we need to check the following listed characteristics:
- The alternate interior angles are equal in measure.
- They lie on the alternate sides of the transversal.
- They lie in between the interior of the two lines.
- In other words, the angles that lie on the inner side of the parallel lines but on the opposite sides of the transversal.
How To Solve Alternate Interior Angles?
According to the alternate interior angles theorem, if two parallel lines are crossed by a transversal, then the alternate interior angles are equal in measure. Using this theorem, we can find the measure of alternate interior angle if we know the measure of the corresponding alternate interior angle.
What is the Converse of the Alternate Interior Angles Theorem?
According to the converse of the alternate interior angles theorem, if a transversal intersects two lines such that the alternate interior angles are equal, then the two lines are said to be parallel.
What Is the Difference Between Alternate Interior and Exterior Angles?
Alternate interior angles are those angles that have different vertices, they lie on the alternate sides of the transversal and are in between the interior of the two lines. Whereas alternate exterior angles are those angles that have different vertices, they lie on the alternate sides of the transversal, but they lie on the outer side of the two lines.
What Is the Difference Between Corresponding and Alternate Angles?
Corresponding angles are two angles that lie on the same side of the transversal in which one is interior and the other is exterior. The alternate angles are two angles that lie on the opposite sides of the transversal.
How To Solve Alternate Interior Angles?
According to the alternate interior angles theorem, if two parallel lines are crossed by a transversal, then the alternate interior angles are equal in measure. Using this theorem, we can find the measure of alternate interior angle if we know the measure of the corresponding alternate interior angle.
Are Alternate Interior Angles Congruent or Supplementary?
As per the alternate interior angles theorem, the alternate interior angles of two parallel lines are congruent.
visual curriculum