Alternate Exterior Angles
Alternate exterior angles are formed when a transversal intersects two or more parallel lines at distinct points. The term exterior means something which is situated externally. Alternate exterior angles always
- lie outside two lines that are intersected by the transversal and
- they are located on the opposite sides of the transversal.
Thus, the two exterior angles which form at the alternate ends of the transversal in the exterior part are considered a pair of alternate exterior angles and they are always equal. We get two such pairs of alternate exterior angles when a transversal cuts two parallel lines. Let us learn more about them.
| 1. | What are Alternate Exterior Angles? |
| 2. | Alternate Exterior Angles Theorem |
| 3. | Converse of Alternate Exterior Angles Theorem |
| 4. | FAQs on Alternate Exterior Angles |
What are Alternate Exterior Angles?
Alternate exterior angles are angles that are formed on the outer side of the transversal on the different sides.
When any two parallel lines are intersected by a transversal, they create some pairs of angles with the transversal. Interior angles are created in the space inside the parallel lines whereas alternate exterior angles are created in the space outside the parallel lines.
In the given figure below, Line AB || Line CD and are intersected by the transversal MN. Here, the alternate exterior angle pairs are ∠1 and ∠7; ∠2 and ∠8. This means ∠1 = ∠7; ∠2 = ∠8
We can conclude that ∠2 and ∠8 are alternate exterior angles as
- ∠2 is on the right side of the transversal M and 8 is on the left side. i.e., they lie on either sides of the transersal and
- ∠2 is above the line AB whereas ∠8 is below the line CD. i.e., they lie outside the two lines.
The same rule applies to the other pair of angles (∠1 and ∠7). Therefore, the pair of angles that satisfy these conditions are called alternate exterior angles.
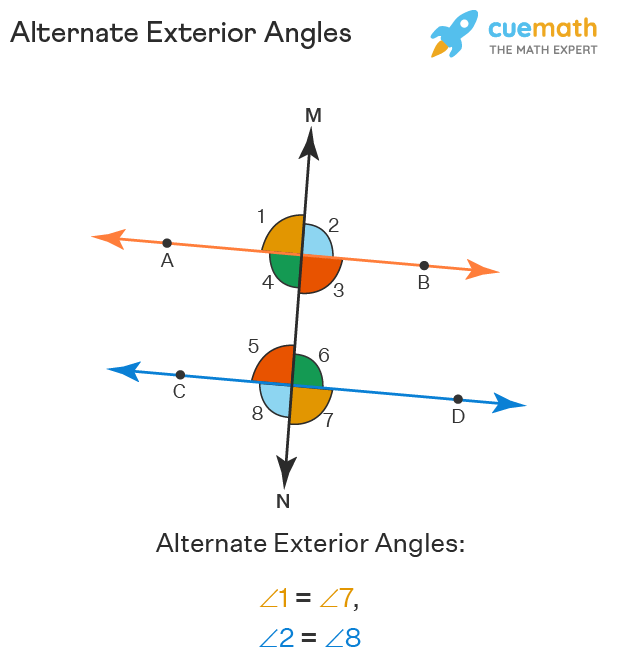
According to the figure, we can define alternate exterior angles as:
Two exterior angles that lie on two different lines cut by a transversal and are placed on opposite sides of the transversal are called alternate exterior angles.
In the figure, we can observe that the pairs ∠1 & ∠7 and ∠2 & ∠8 are alternate exterior angles because:
- Both lie on the exterior side of the lines; and
- They are placed on the opposite sides of the transversal.
Alternate Exterior Angles Theorem
The alternate exterior angle theorem states "if two lines are parallel and are intersected by a transversal, then the alternate exterior angles are considered as congruent angles or angles of equal measure."
Following the same figure given above, we can observe that ∠1 and ∠7; ∠2 and ∠8 are pairs of alternate exterior angles. Let us see how they are congruent (equal).
Given: Lines AB and CD are parallel lines on a transversal M.
[Hint: To prove the above theorem, we will be using the following axioms:
Corresponding Angle Axiom: When two lines are parallel the corresponding angles are congruent angles.
The converse of the Corresponding Angle Axiom: When the corresponding angles made by two lines are congruent, then those two lines are parallel.]
To Prove: ∠1 = ∠7
Proof:
To prove this result, we will consider the vertically opposite angle of ∠1. Here, the vertically opposite angle of ∠1 is ∠3.
So, ∠1 = ∠3 (vertically opposite angles)
Here, line AB || CD,
∠3 = ∠7 (corresponding angles axiom)
∴∠1 = ∠7 (transitivity) Hence, proved.
Converse of Alternate Exterior Angles Theorem
The converse of alternate exterior angle theorem states that, if the alternate exterior angles formed by two lines, which are cut by a transversal, are congruent then the lines are parallel.
Hence, in the above figure, if it is given that ∠1 = ∠7 then line AB || line CD.
Note: Apart from alternate exterior angles, there is one more type of exterior angles pair. This is called the pair of consecutive exterior angles (also known as co-exterior angles). There are two pairs of consecutive exterior angles in the above figure.
∠2 and ∠7
∠1 and ∠8
Consecutive exterior angles are supplementary, i.e., ∠2 + ∠7 = 180° and ∠1 + ∠8 = 180°
☛ Related Topics:
Alternate Exterior Angles Examples
-
Example 1: Identify the alternate exterior angles in the figure given below.

Solution:
By the alternate exterior angles definition,
∠1 & ∠8 and ∠5 and ∠4 are alternate exteriors as they lie outside the two lines and are on either side of the transversal in each pair.
Solution: ∠1 & ∠8; ∠4 & ∠5
-
Example 2: Using the alternate exterior angle theorem solve the given problem: Given: Line RS || Line PQ.
a) Find x.
b) Also check if line XY || line RS.
Solution:
i) Given: Line RS || Line PQ; ∠XBA = 30° and ∠QCD = 30°
Here, ∠QCD = ∠EDS (corresponding angles)
∴ x = 30°⋯(1)
ii) Now let us check if lines XY and RS are parallel or not.
It is given that Line RS || Line PQ
AE is a transversal bisecting three lines XY, PQ, and RS
Also, given that ∠XBA = ∠EDS = 30°, where ∠XBA = ∠EDS are alternate exterior angles.
Then by the converse of the alternate exterior angle theorem, we can conclude that XY is parallel to RS
Answer: ∴ a) x = 30° , b) line XY || line RS
Example 3:
In a given set of 2 parallel lines which are cut by a transversal, if the alternate exterior angles are shown as (2x + 26)° and (3x−33)°, find the value of x and the actual value of the alternate exterior angles with the help of the alternate exterior angles theorem.
Solution:
Since alternate exterior angles are always equal in measure for a given set of parallel lines, we can find the value of x.(2x + 26)° = (3x − 33)°
2x − 3x = −33° − 26°
−x = −59°
∴ x = 59°
By substituting the value of x in the given angles we can find the exact value of the angles.
a) (2x + 26)° = [2(59) + 26]° = 144°
b) (3x − 33)°= [3(59) − 33]° =144°
Answer: Both have the same value because they are alternate exterior angles.

FAQs on Alternate Exterior Angles
What are Alternate Exterior Angles in Math?
Alternate exterior angles are two exterior angles that lie on two different lines cut by a transversal and are placed on opposite sides of the transversal are called alternate exterior angles. If two lines are cut by a transversal, there are two pairs of alternate exterior angles formed.
What is the Alternate Exterior Angles Theorem?
The alternate exterior angle theorem states that when two parallel lines are intersected by a transversal, then the exterior angles formed on either side of the transversal are equal.
Do Alternate Exterior Angles Prove that the Lines are Parallel?
Alternate exterior angles prove that the lines are parallel only if they are congruent. This result is known as the converse of the alternate exterior angle theorem.
How are Alternate Exterior Angles Related?
According to the alternate exterior angle theorem, when two parallel lines are intersected by a transversal, then the alternate exterior angles are considered congruent angles (equal angles).
Do Alternate Exterior Angles Add up to 180?
No, alternate exterior angles do not add up to 180°. In fact, they are congruent to each other. Only in the case when they are 90° each, then they will sum up to 90° + 90° = 180°.
Are Alternate Exterior Angles Congruent?
Alternate exterior angles are congruent when the lines are parallel. If the lines are not parallel, the alternate exterior angles are not congruent.
What are Alternate Interior and Alternate Exterior Angles?
When any two parallel lines are intersected by a transversal, they create some pairs of angles with the transversal. Alternate interior angles are always equal and are formed on the inner side of the parallel lines, but are located on the opposite sides of the transversal. Alternate exterior angles are those angles that have different vertices, they lie on the alternate sides of the transversal and are exterior to the lines.
When are Alternate Exterior Angles Congruent?
Alternate Exterior angles are congruent only when the two lines that are intersected by a transversal are parallel.
visual curriculum